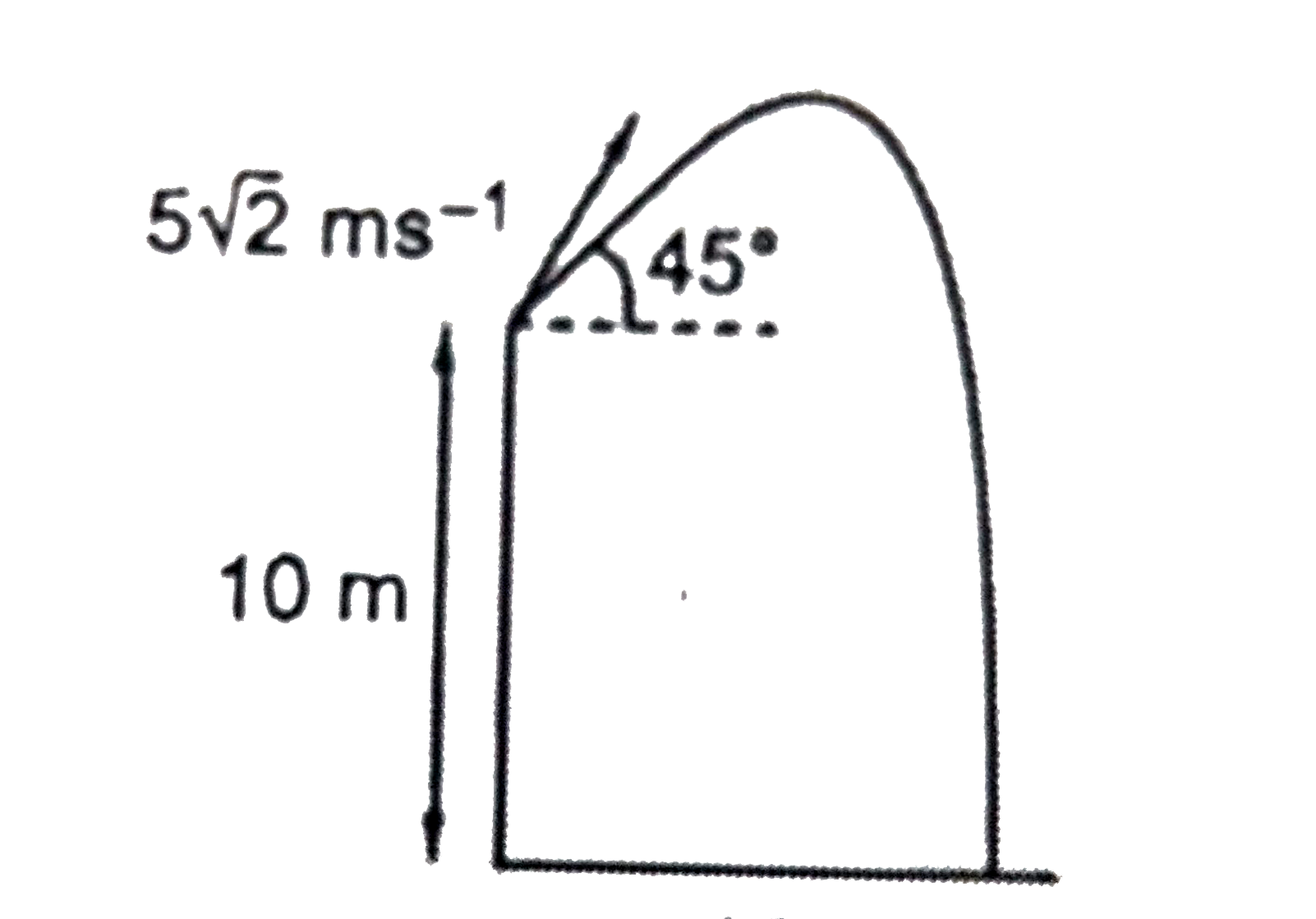
In the figures shown, three particles are thrown from a tower of height 40 m as shown in figure. In each case find the time when the particles strike the ground and the distance of this point from foot of the tower.
 ,
,  ,
,  .
.
The correct Answer is:A
For time calculation, apply
sy=uyt+12ayt2
In vertical direction.
In all figures, s=−40m,ay=−10m/s2
In first figures, uy=+20(√2)cos45∘=+20m/s
In second figure uy=0
In third figure, uy=−20(√2)cos45∘=−20m/s
For horizontal distance,
x=uxt
ux=20m/s in all cases
Substituting the proper values and then solving we get,
In first figure
Time t1=5.46s and horizontal distance x1=109.2m
In second figure
Time t2=2.83 s and the horizontal distance x2=56.6m
In third figure
Time t3=1.46 s and the horizontal distance x3=29.2m.
Topper's Solved these Questions
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Example Type 3
4 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Example Type 4
1 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Example Type 1
1 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEYCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
Similar Questions
Knowledge Check
In the figure shown, find
(a) the time when the particle strikes the ground at P.
(b) the horizontal distance QP
(c) velocity of the particle at P.
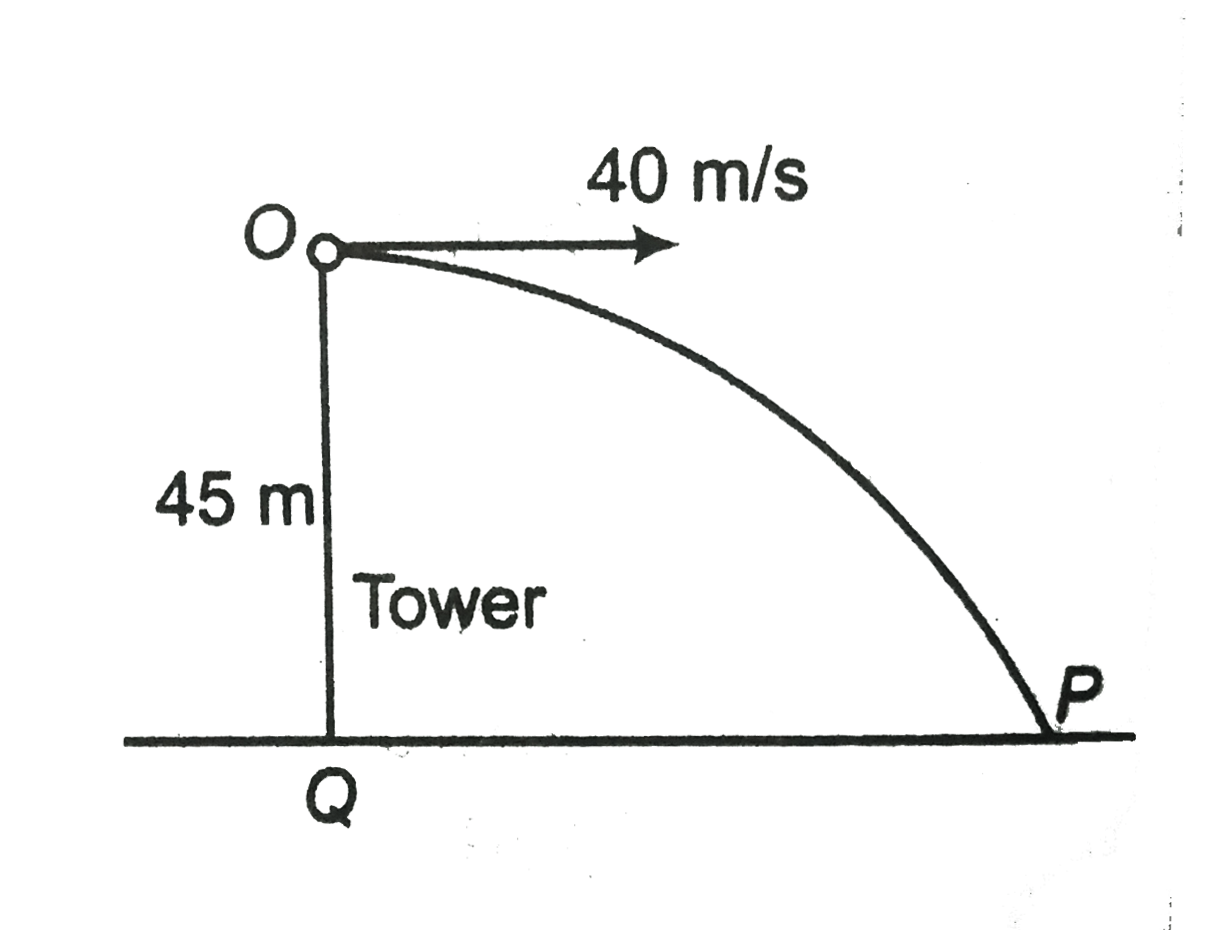 A6s , 120m , 40m/s , 50m/s 37∘B3s , 12m , 40m/s , 50m/s 37∘C3s , 120m , 4m/s , 50m/s 37∘D3s , 120m , 40m/s , 50m/s 37∘
A6s , 120m , 40m/s , 50m/s 37∘B3s , 12m , 40m/s , 50m/s 37∘C3s , 120m , 4m/s , 50m/s 37∘D3s , 120m , 40m/s , 50m/s 37∘
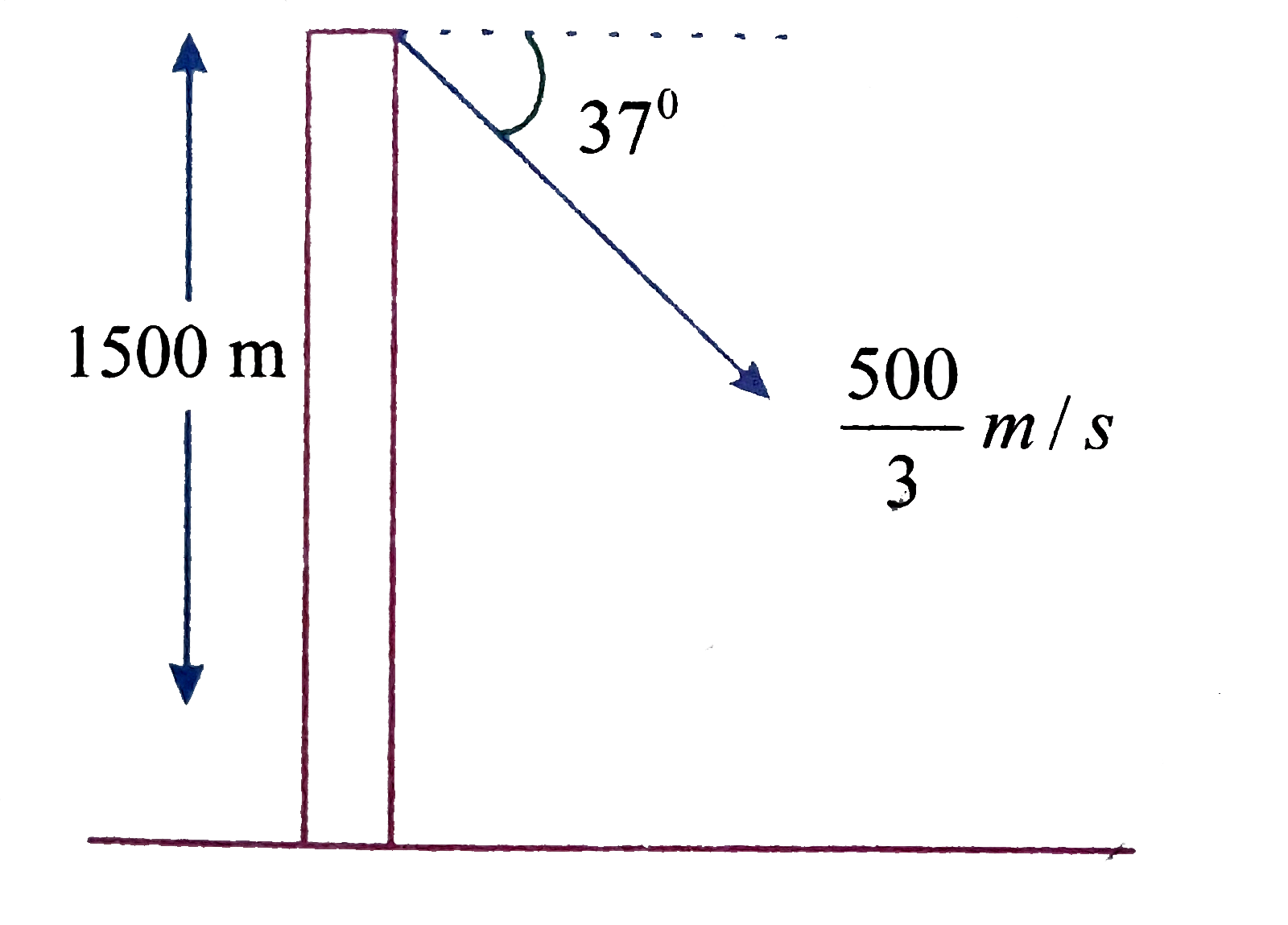
A particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
View SolutionA particle is dropped from the top of a tower of height 80m. Find the time of jurney and the speed with which it strikes the ground.
View SolutionFrom the top of a tower 180 m high, it was observed that the angle of depression of the bottom of a cat sitting on the ground was 30∘. Find the distance of the cat from the foot of the tower.
View SolutionA particle is projected from a tower of height 25 m with velocity 20√2m/s at 45∘. Find the time when particle strikes with ground. The horizontal distance from the foot of tower where it strikes. Also find the velocity at the time of collision.
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
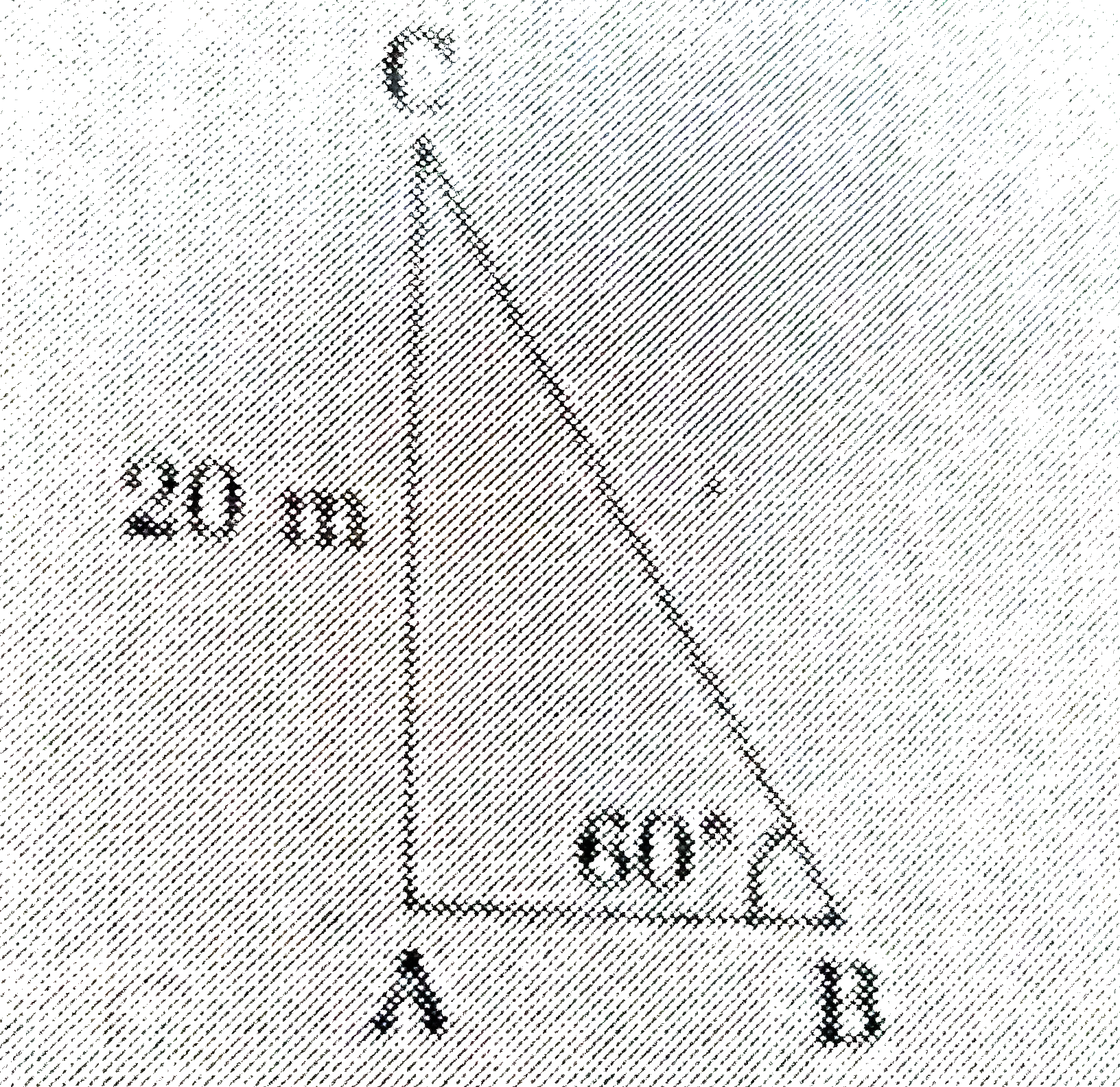
View SolutionA particle is thrown horizontally from a tower of height 20m. If the speed of projection is 20ms then the distance of point from the tower,where the particle hit the ground is:-
View Solution