Two particles are moving along x-axis. Their x-coordinate versus time graph are as shown below.
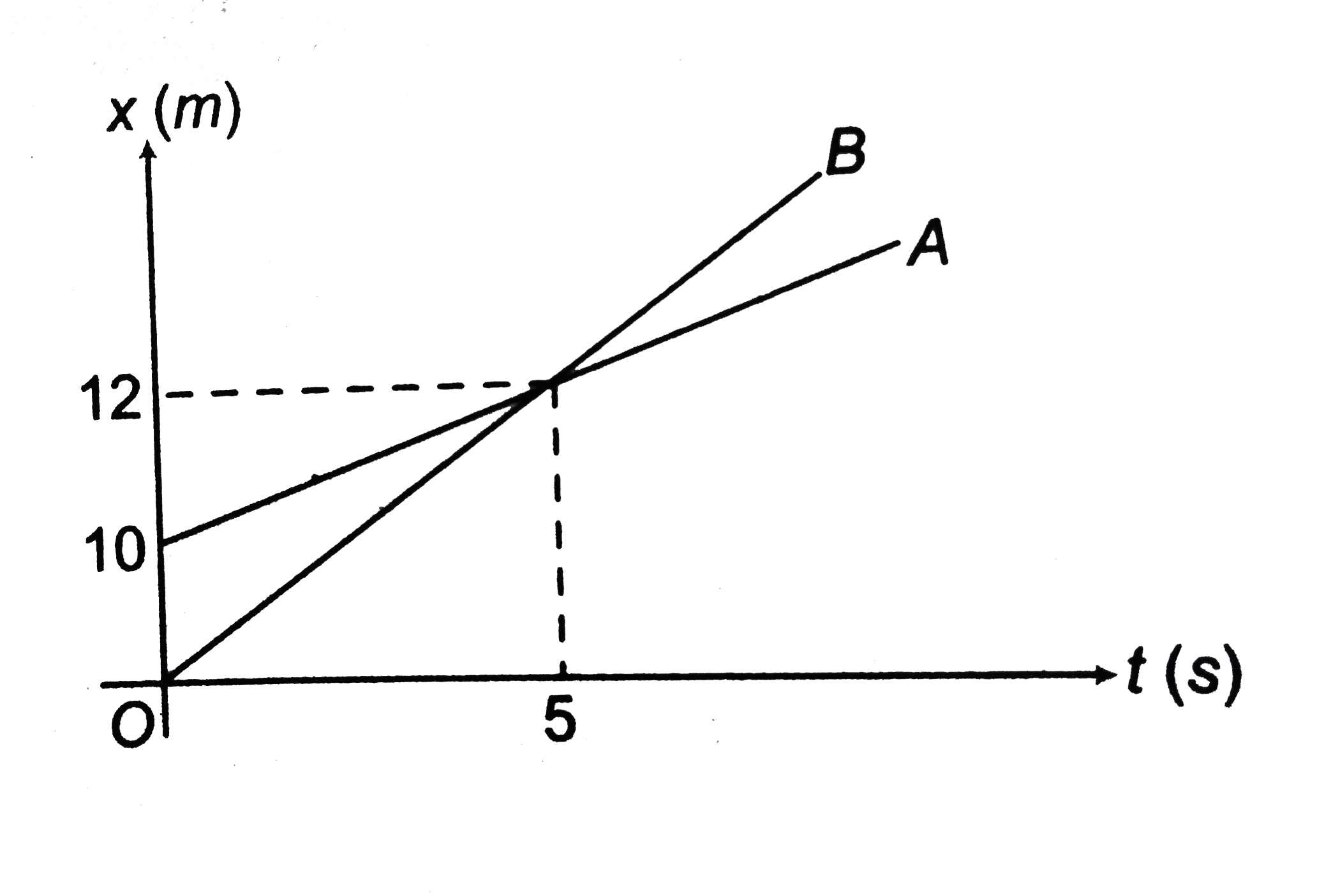
Find velocity of A w.r.t. B
The correct Answer is:B
vA=SlopeofA=25=0.4m/s
vB=SlopeofB=125=2.4m/s
∴VAB=vA−vB=−2m/s
Topper's Solved these Questions
- KINEMATICSBook:DC PANDEYChapter:KINEMATICSExercise:Assertion And Reason
Explore 12 Videos
- KINEMATICSBook:DC PANDEYChapter:KINEMATICSExercise:Objective
Explore 45 Videos
- KINEMATICSBook:DC PANDEYChapter:KINEMATICSExercise:Exercise 6.8
Explore 5 Videos
- GRAVITATIONBook:DC PANDEYChapter:GRAVITATIONExercise:(C) Chapter Exercises
Explore 45 Videos
- KINEMATICS 1Book:DC PANDEYChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
Similar Questions
Two particles A and B are moving along x-axis. Their x-coordinate versus time graphs are as shown below

(a) Find the time when the particles start their journey and the x-coordinate at that time.
(b) Find velocities of the two particles.
(c) When and where the particles strike with each other.
View SolutionTwo bodies P and Q are moving along positive x-axis their position-time graph is shown below. If →VPQ is velocity of
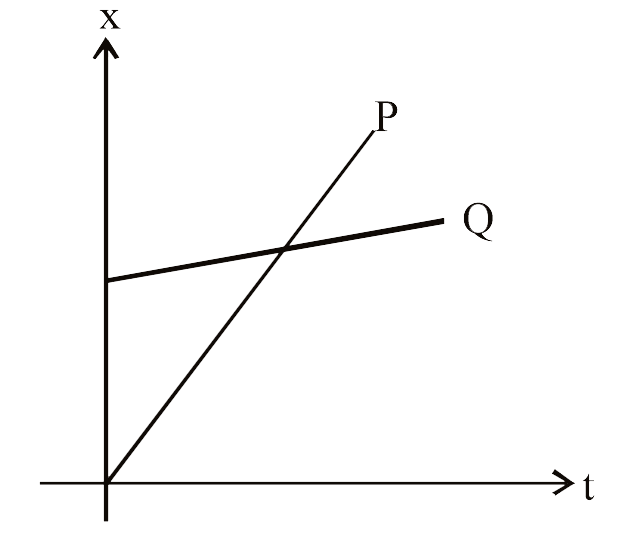
View Solution
Knowledge Check
A particle is moving along x-axis. Its x-coordinate versus time graph is as shown below.
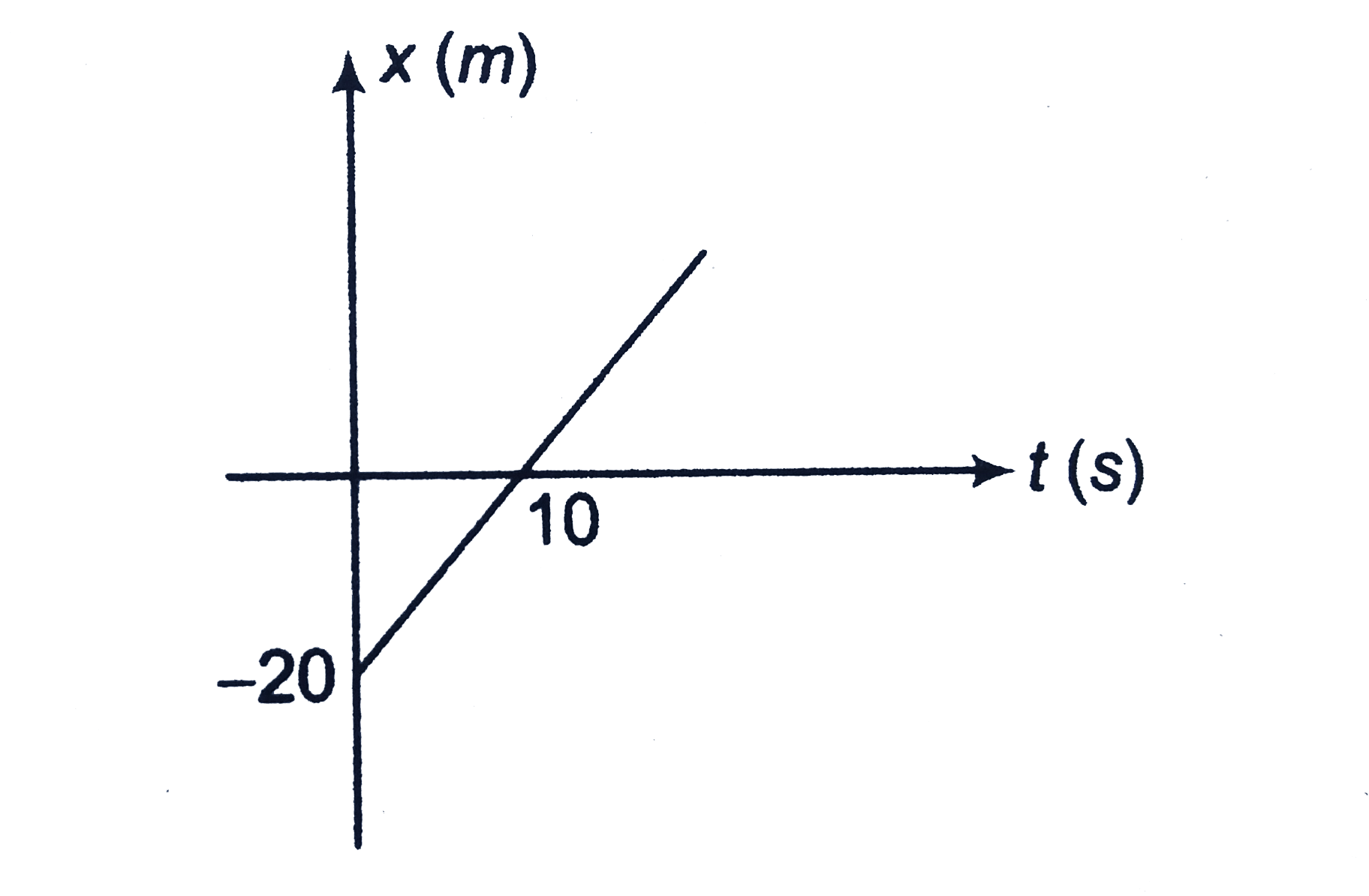 Draw some conclusion from the given graph. A+2msB+1msC+12msD+20ms
Draw some conclusion from the given graph. A+2msB+1msC+12msD+20msA particle starts to move along a straight line. The acceleration versus time graph of particle is as shown in figure. The correct velocity versus time graph is :
 A
A B
B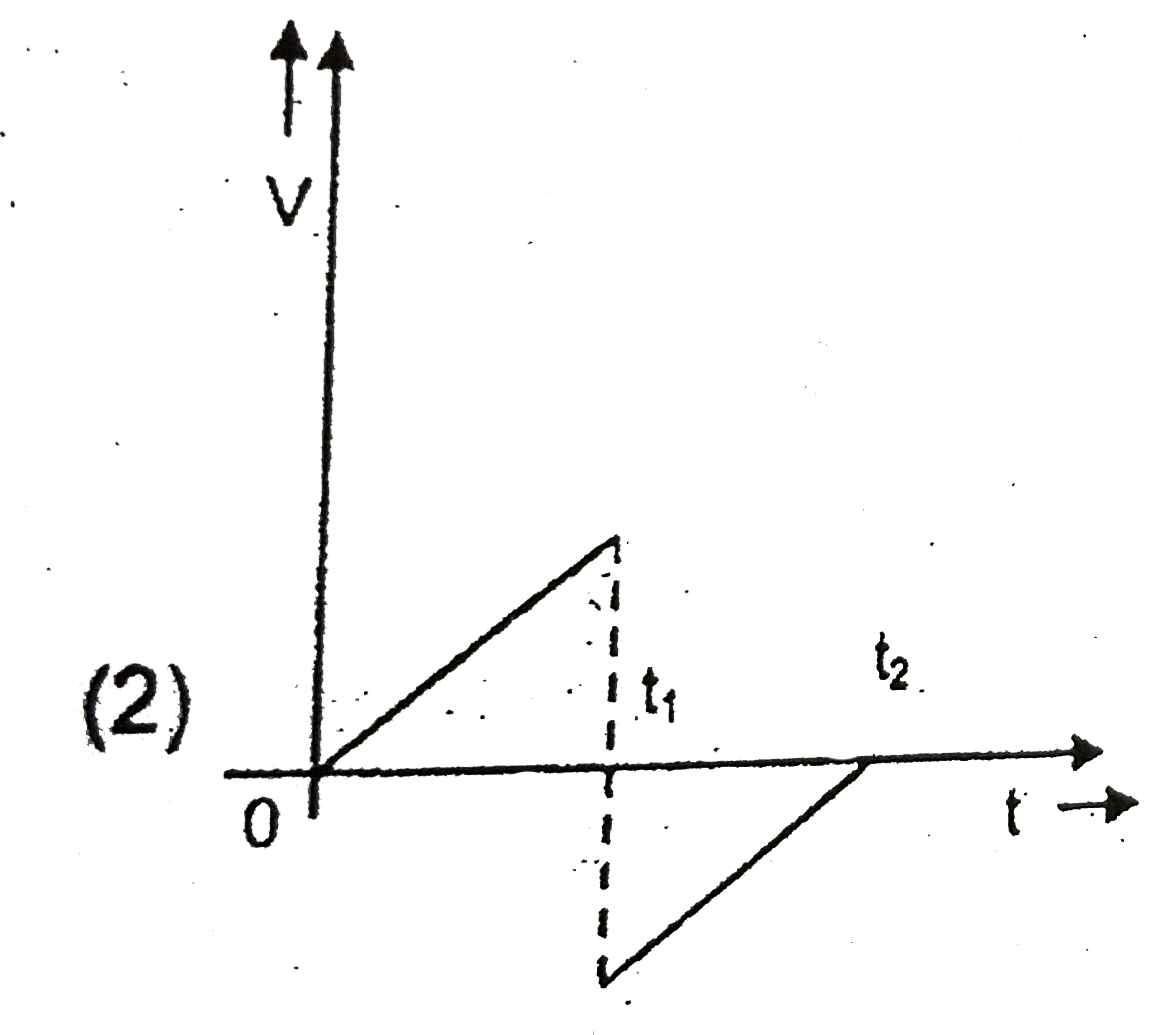 C
C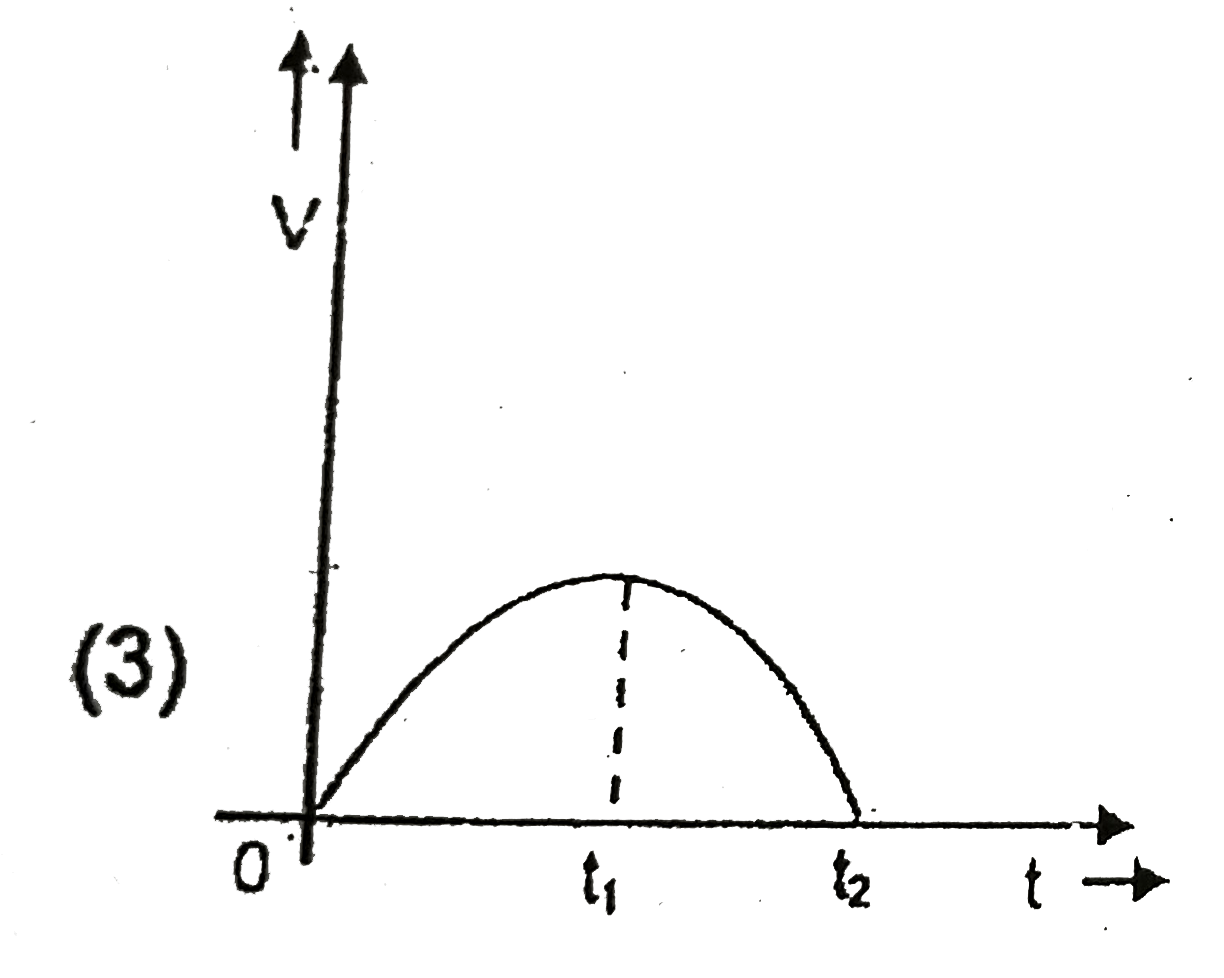 D
D
For a particle moving along x-axis, velocity-time graph is as shown in figure. Find the displacement of the particle?
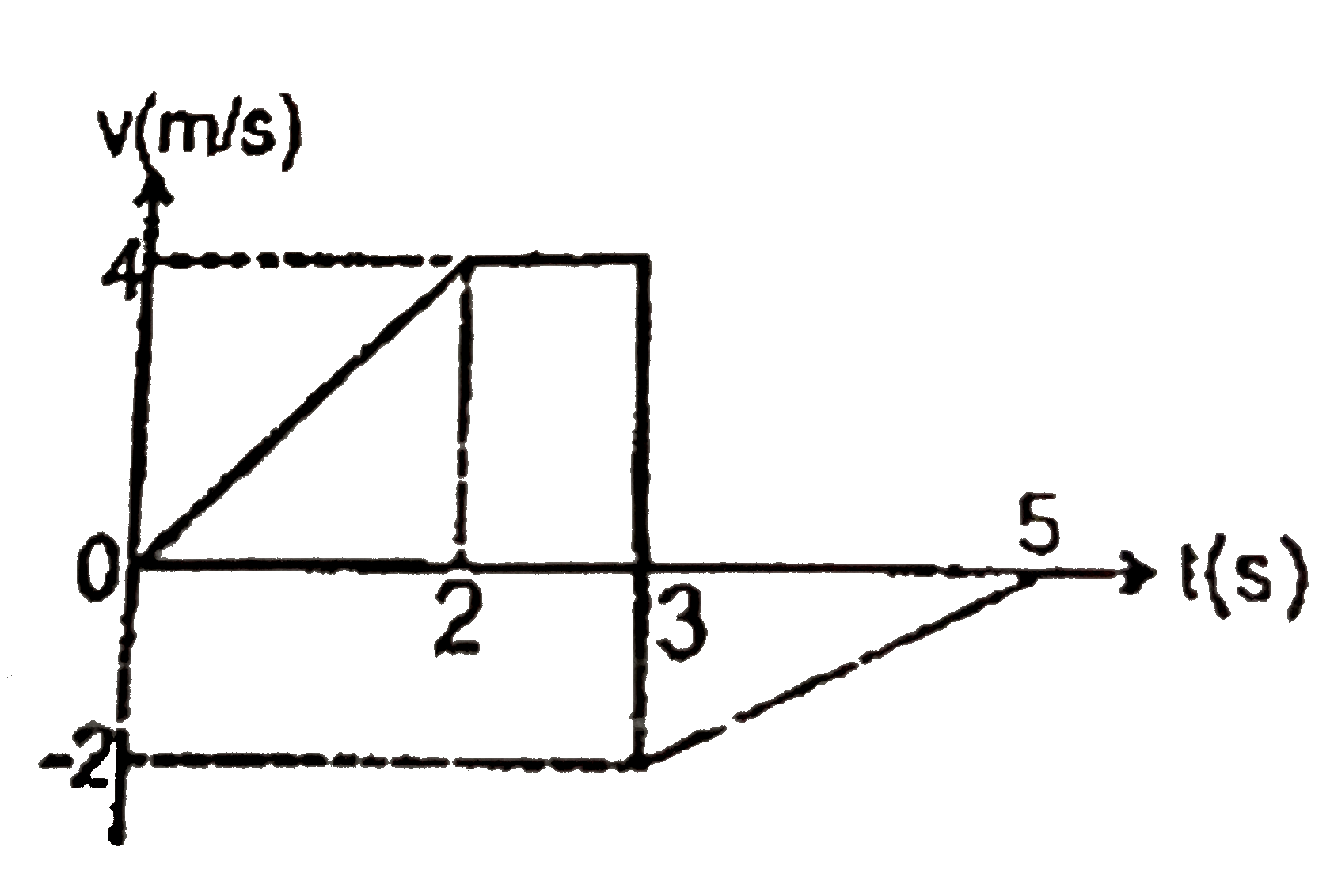
View SolutionThe acceleration-time graph of a particle moving along x-axis is shown in the figure. If the particle starts with velocity 3 m/s at t = 0, find the velocity of particle at t = 4 s.

View Solution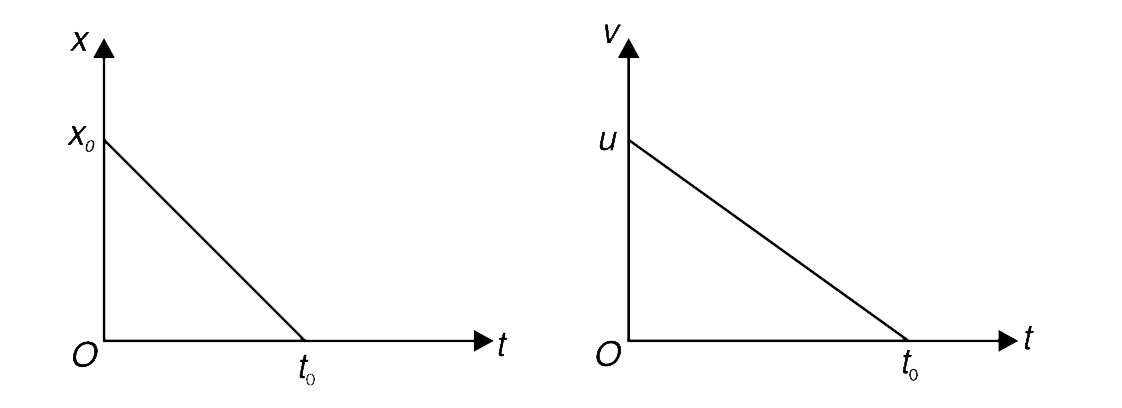
Two particles 1 and 2 move along the x axis. The position (x) - time (t) graph for particle 1 and velocity (v) - time (t) graph for particle 2 has been shown in the figure. Find the time when the two particles collide. Also find the position (x) where they collide. It is given that x0=ut0, and that the particle 2 was at origin at t=0.
View SolutionA particle is moving along x-axis. Its X-coordinate varies with time as, X=2t2+4t−6 Here, X is in meters and t in seconds. Find average velocity between the time interval t=0→t=2s.
View SolutionA particle is moving along the x-axis and its position-time graph is shown. Determine the sign of acceleration.
View SolutionThe magnitude of force acting on a particle moving along x-axis varies with time (t) as shown in figure. If at t = 0 the velocity of particle is v0, then its velocity at t=T0 will be

View Solution