In an ΔABC as showin in Fig. 2 . (2) .71 (a) prove
that asinA=bsinB=CsinC.
Video Solution
Text Solution
From polygon law of vector addition we can see AB+BC+CA=0
∴cˆi+(asinBˆj−acosBˆi)
+(−bcosAˆi−bsinAˆj)=0
∴(c−acosB−bcosA)ˆi
+(asinB−bsinA)ˆj=0
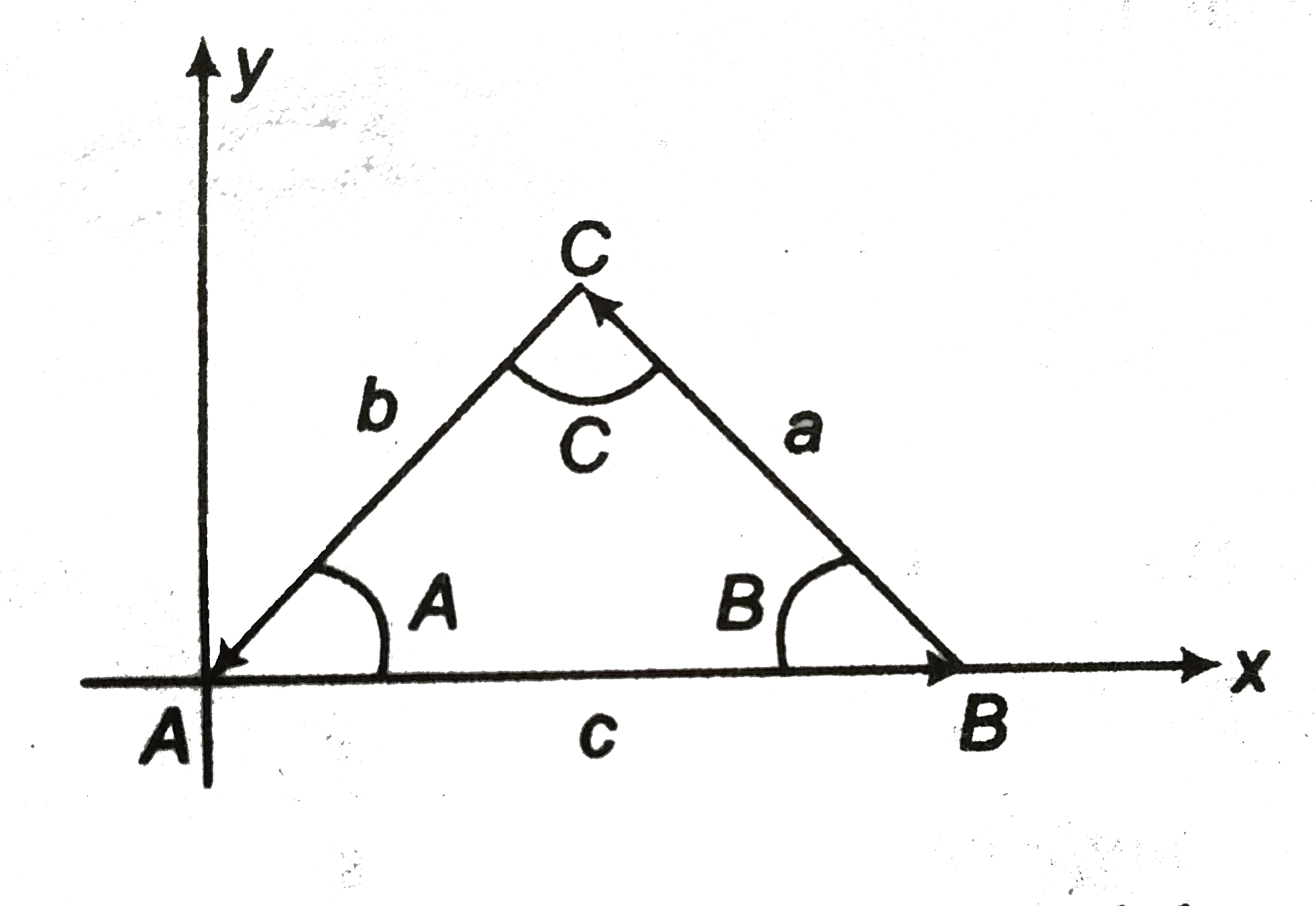
Putting coefficient of ˆj=0, we find that,
asinA=bsinB
Now taking B as origin and BC as the x-axis, we can
prove other relation.
|
Topper's Solved these Questions
- VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - Example
39 Videos
- VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - check point 2.1
15 Videos
- VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - Single Correct
39 Videos
- UNITS, DIMENSIONS & ERROR ANALYSIS BOOK - DC PANDEYCHAPTER - UNITS, DIMENSIONS & ERROR ANALYSIS EXERCISE - Medical entrances gallery
32 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
Similar Questions
In any ΔABC, prove that sinBsin(B+C)=ba
View SolutionIn any △ABC, prove that asinA−bsinB≡csin(A−B).
View SolutionIn a ΔABC, if ∠C=90∘ , prove that sin2A+sin2 B = 1.
View SolutionIf A,BandC are the vetices of a triangle ABC, then prove sine rule asinA=bsinB=csinC.
View SolutionIn a Δ(ABC) prove that cos(A+B2)=sinC2.
View SolutionIn a ΔABC(a2sinA+b2sinB+c2sinC)⋅sinA2sinB2sinc2 simplifeis to
View SolutionIn any ΔABC, prove that sinBsinC=c−acosBb−acosC
View SolutionIn any ΔABC , prove that: sinBsinC=c−acosBb−acosC
View SolutionIn △ABC , prove that sin(A−B)sin(A+B)=a2−b2c2.
View SolutionIn ΔABC, if sin2A+sin2B=sin2C, then show that a2+b2=c2.
View Solution
