Area bounded by curve y=sinx, whit x-axis, when x varies from 0 to π2 is :-
A
1 unit
B
2 units
C
3 units
D
0
Video Solution
Text Solution
The correct Answer is:A
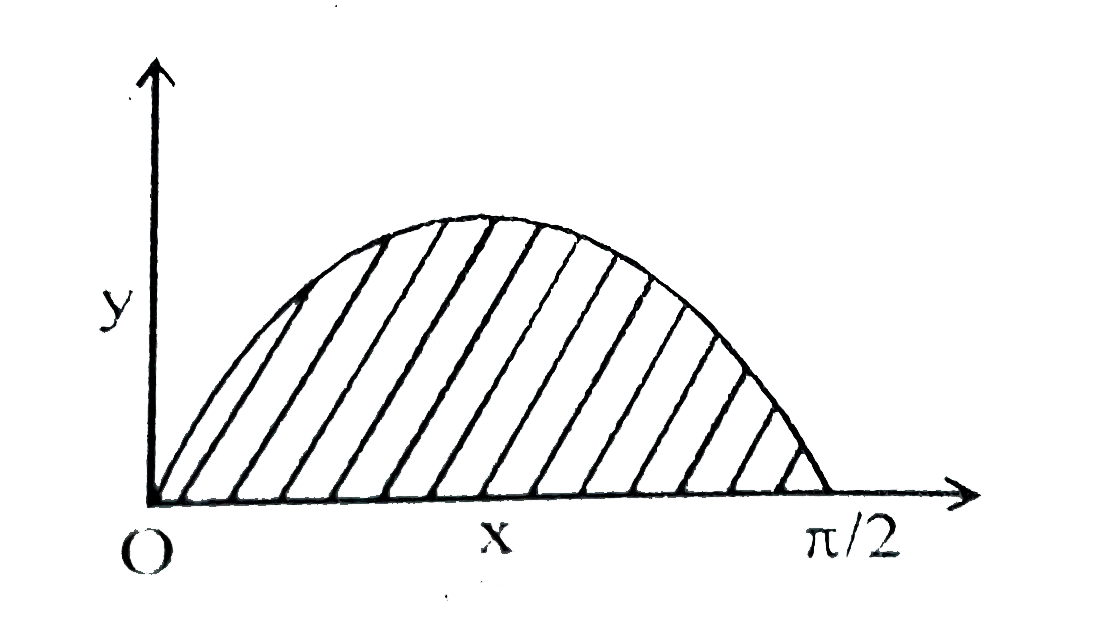
area under of curve ∫π/20ydx
=∫π/20sinxdx
=−cosx∣∣π/20
=−[cosπ2−cos0∘]=1
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The area bounded by the curve y=sin2x, axis and y=1, is
A1B1/4Cπ/4Dπ/4−1/2The area bounded by curves y = tan x, x-axis and x=π3 is-
A2 log 2Blog 2Clog(2√3)D0The area bounded by the curve y=sin−1(sinx) and the x - axis from x=0 to x=4π is equal to the area bounded by the curve y=cos−1(cosx) and the x - axis from x=−π to x=a, then the value of a is equal to
Aπ2B2πCπD3π2
Area bounded by the curve y=max{sin x, cos x} and x -axis, between the lines x=π4 and x=2π is equal to
View SolutionArea bounded by the curves y=xsinxand x-axis between x=0andx=2π is
View SolutionThe area bounded by the curve y=sin2x−2sinx and the x-axis, where x∈[0,2π], is
View SolutionArea bounded by the curves y = x ,y =tanxandx=π4 is
View SolutionThe area bounded by the curve y=4sinx, x-axis from x=0 to x=π is equal to :
View Solution
