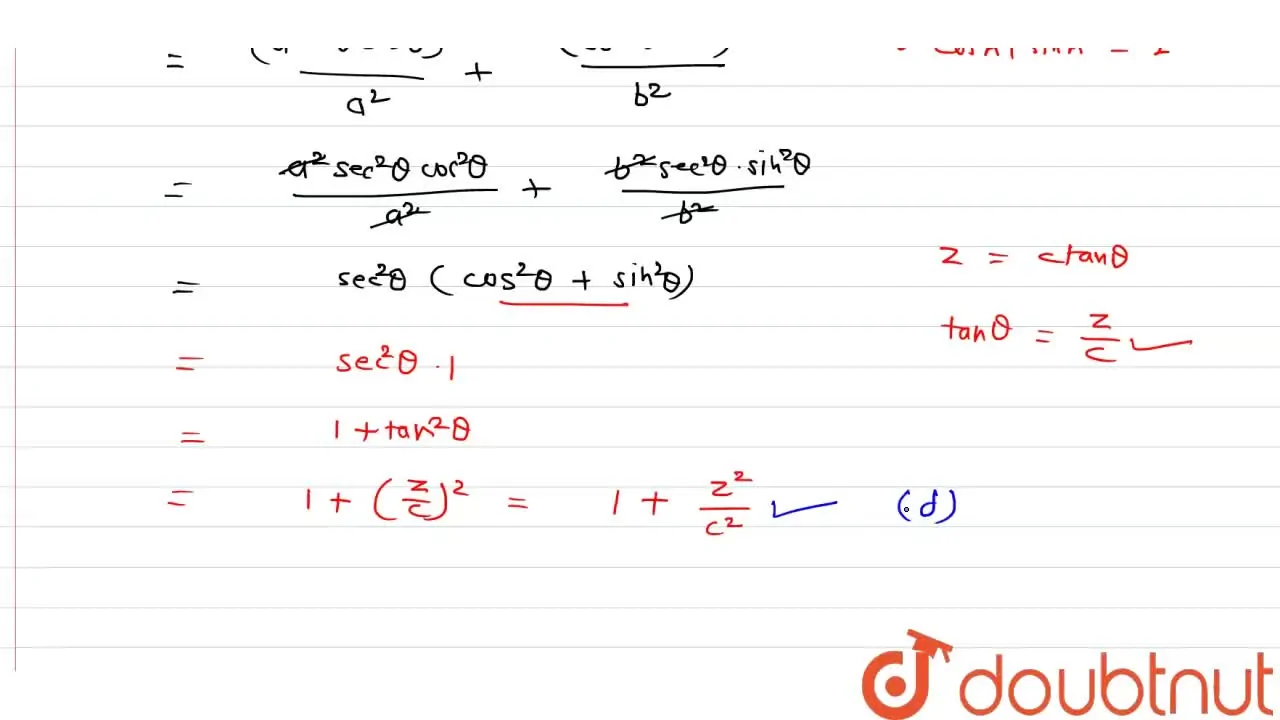
यदि $x = a sec θ cos θ, y = b sec θ sin θ$ तथा $z = c tan θ$ हो, तो $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} =$

$\frac{z^{2}}{c^{2}}$

$1 - \frac{z^{2}}{c^{2}}$

$\frac{z^{2}}{c^{2}} - 1$

$1 + \frac{z^{2}}{c^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

Answer

Step by step video, text & image solution for यदि x=a sec theta cos theta, y=b sec theta sin theta तथा z=c tan theta हो, तो (x^(2))/(a^(2))+(y^(2))/(b^(2))= by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS INTRODUCTION TO TRIGONOMETRY

Topper's Solved these Questions

त्रिकोणमितीय सर्वसमिकाएँ BOOK - RD SHARMACHAPTER - त्रिकोणमितीय सर्वसमिकाएँ EXERCISE - अति लघुत्तरात्मक प्रश्न
23 Videos
त्रिकोणमितीय के कुछ अनुप्रयोगBOOK - RD SHARMACHAPTER - त्रिकोणमितीय के कुछ अनुप्रयोगEXERCISE - बहु विकल्पीय प्रश्न
20 Videos
त्रिभुजेंBOOK - RD SHARMACHAPTER - त्रिभुजेंEXERCISE - बहु विकल्पीय प्रश्न
45 Videos

यदि $x = a sec θ cos θ, y = b sec θ sin θ$ तथा $z = c tan θ$ हो, तो $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} =$

The correct Answer is:D

Topper's Solved these Questions

Similar Questions

RD SHARMA-त्रिकोणमितीय सर्वसमिकाएँ -बहु विकल्पीय प्रश्न

यदि x=asecθcosθ,y=bsecθsinθ तथा z=ctanθ हो, तो x2a2+y2b2=

The correct Answer is:D

Topper's Solved these Questions

Similar Questions

RD SHARMA-त्रिकोणमितीय सर्वसमिकाएँ -बहु विकल्पीय प्रश्न

यदि $x = a sec θ cos θ, y = b sec θ sin θ$ तथा $z = c tan θ$ हो, तो $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} =$