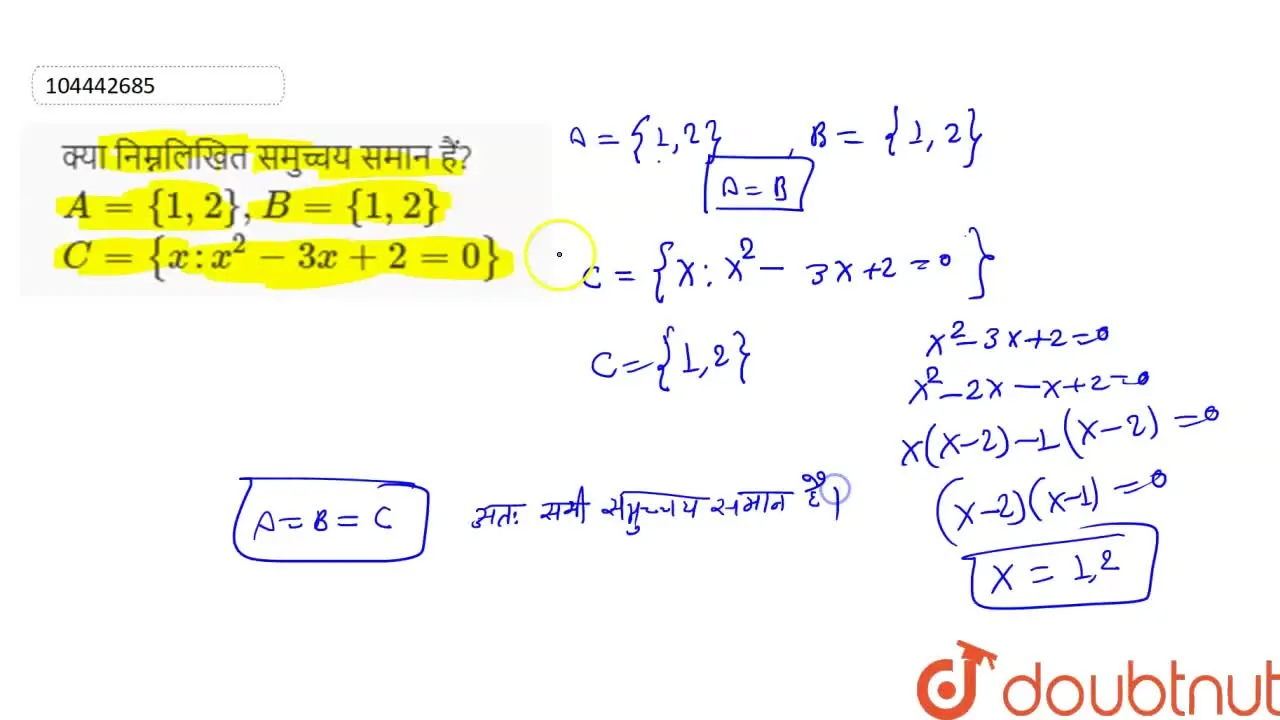
क्या निम्नलिखित समुच्चय समान हैं?
A={1,2},B={1,2}
C={x:x2−3x+2=0}
The correct Answer is:हाँ, A=B=C
Step by step video, text & image solution for क्या निम्नलिखित समुच्चय समान हैं? A={1, 2}, B={1, 2} C={x : x^(2)-3x+2=0} by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - KC SINHACHAPTER - समुच्चय EXERCISE - अभ्यास 1.258videos
समुच्चय
- BOOK - KC SINHACHAPTER - समुच्चय EXERCISE - अभ्यास 1.3124videos
समुच्चय
- BOOK - KC SINHACHAPTER - समुच्चय EXERCISE - वस्तुनिष्ठ प्रश्न39videos
समुच्चय
- BOOK - KC SINHACHAPTER - संबंधEXERCISE - OBJECTIVE QUESTIONS17videos
संबंध
- BOOK - KC SINHACHAPTER - सम्मिश्र संख्याएँEXERCISE - अभ्यास 10.425videos
सम्मिश्र संख्याएँ
Similar Questions
रिक्त स्थानों में संकेत ⊆ या cancel(sube) को भरकर सही कथन बनाइए :
{a,b,c}....{b,c,d}02:51View Solutionयदि A={1,2,3,4},B={3,4,5,6},C={5,6,7,8} और D={7,8,9,10} तो निम्नलिखित ज्ञात कीजिए। :
B∪C01:15View Solutionयदि A={x:x=3n+1,2≤n<6} जहाँ n एक प्राकृतिक संख्या है ,तो A के उपसमुच्चयों की संख्या है
02:04View Solutionदो परिमित समुच्चयों A और B में क्रमशः m और n अवयव हैं। यदि A से B में कुल 64 संबंध है तो m और n के संभव मान क्रमशः है:
01:09View Solution√i=?
02:40View Solution3x+4(x+1)2(x−1)=Ax−1+Bx+1+C(x+1)2 then A=
06:06View SolutionIf x+a is a factor of x4−a2x2+3x−6a, then a= 0 (b) −1 (c) 1 (d) 2
02:07View SolutionThe value of |[a,a+d,a+2d] , [a+d,a+2d,a+3d] , [a+2d,a+3d,a+4d]|+|[b
03:10View SolutionIf : f(x)=sec2x, lt br gt then : (1+tanA⋅tanB)2+(tanA−tanB)2=
02:52View SolutionIf 3x+4(x+1)2(x−1)=Ax−1+Bx+1+C(x+1)2 then A=
01:41View Solution3x−2(x+1)2(x+2)=Ax+1+B(x+1)2+Cx+3, then A+B+C =
01:03View Solution1) Fragmentation () a) Planaria br 2) Unripe fruits () b) Yeast br 3) Desire () c) Spirogyra br 4) Reproduction () d) Watermelon br
01:42View SolutionIf a,b,c are in A.P .and f(x)=∣∣ ∣ ∣∣x2+x+a+1x2+112x2+x+b−12x2−113x2+x+c−23x2−21∣∣ ∣ ∣∣ then f'(x) is
08:16View SolutionWrite the coefficient of x2 in each of the following br> (i)15−3x+2x2
(ii) 1−x2
(iii) πx2−3x+5
(iv)√2x2+5x−101:38View SolutionWrite the coefficient of x2 in each of the following br> (i)15−3x+2x2
(ii) 1−x2
(iii) πx2−3x+5
(iv)√2x2+5x−103:10View Solution