Attempt any THREE :
Show that beats frequency is equal to frequency difference between two interfering waves.
To show that the beats frequency is equal to the frequency difference between two interfering waves, we can follow these steps:
Step 1: Define the two waves
Let the two waves be represented as:
- Wave 1: y1=Asin(ω1t)
- Wave 2: y2=Asin(ω2t)
Here, A is the amplitude of the waves, ω1 and ω2 are the angular frequencies of the two waves.
Step 2: Write the resultant wave
The resultant wave y when these two waves interfere is given by:
y=y1+y2=Asin(ω1t)+Asin(ω2t)
Step 3: Use the trigonometric identity
We can use the trigonometric identity for the sum of sines:
sina+sinb=2sin(a+b2)cos(a−b2)
Applying this identity, we have:
y=2Asin(ω1+ω22t)cos(ω1−ω22t)
Step 4: Identify the frequencies
The term sin(ω1+ω22t) represents a wave with an average frequency, while the term cos(ω1−ω22t) represents the modulation of the amplitude, which is responsible for the beats.
Step 5: Calculate the beat frequency
The beat frequency f is given by the frequency of the cosine term:
f=ω1−ω22π
Since ω=2πf, we can express the beat frequency in terms of the original frequencies:
f=(2πf1−2πf2)2π=f1−f2
Conclusion
Thus, we have shown that the beat frequency is equal to the frequency difference between the two interfering waves:
f=|f1−f2|
Let the two waves be represented as:
- Wave 1: y1=Asin(ω1t)
- Wave 2: y2=Asin(ω2t)
The resultant wave y when these two waves interfere is given by:
y=y1+y2=Asin(ω1t)+Asin(ω2t)
We can use the trigonometric identity for the sum of sines:
sina+sinb=2sin(a+b2)cos(a−b2)
Applying this identity, we have:
y=2Asin(ω1+ω22t)cos(ω1−ω22t)
The term sin(ω1+ω22t) represents a wave with an average frequency, while the term cos(ω1−ω22t) represents the modulation of the amplitude, which is responsible for the beats.
The beat frequency f is given by the frequency of the cosine term:
f=ω1−ω22π
Since ω=2πf, we can express the beat frequency in terms of the original frequencies:
f=(2πf1−2πf2)2π=f1−f2
Thus, we have shown that the beat frequency is equal to the frequency difference between the two interfering waves:
f=|f1−f2|
Topper's Solved these Questions
- JULY 2018BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - JULY 2018EXERCISE - SECTION-II
21 Videos
- JULY 2017BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - JULY 2017EXERCISE - SECTION-I
12 Videos
- MARCH 2015BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2015EXERCISE - SECTION-II
21 Videos
Similar Questions
Why no beats can be heard if the frequencies of the two interfering waves differ by more then ten ?
View SolutionInterference is an important property of light. In the given table, Column I shows the properties of interfering waves, Column II shows the phase difference, and Column III shows the path difference between two interfering waves
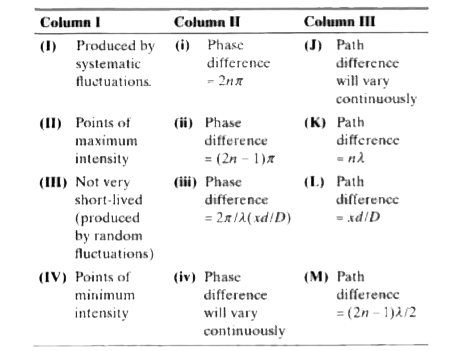
What are the conditions for constructive interference?
View Solution
Knowledge Check
For constructive interference, the phase difference between the two interfering waves is
Aπ2 radianB2 radianCzeroDπ6 radianInterference is an important property of light. In the given table, Column I shows the properties of interfering waves, Column II shows the phase difference, and Column III shows the path difference between two interfering waves

What are the conditions for sustained interference?A(II) (ii) (J)B(III) (iii) (L)C(IV) (i) (L)D(II) (iii) (M)Interference is an important property of light. In the given table, Column I shows the properties of interfering waves, Column II shows the phase difference, and Column III shows the path difference between two interfering waves
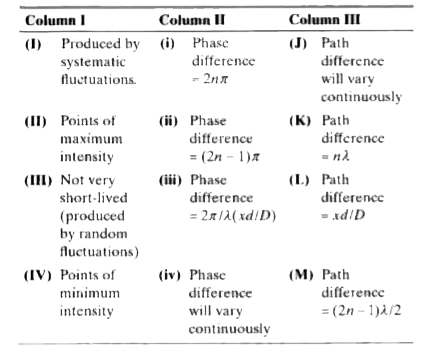
What are the conditions for destructive interference?A(I) (ii) (J)B(IV) (ii) (M)C(II) (iii) (L)D(I) (i) (M)
The relationship between phase difference Δϕ and the path difference Δr between two interfering waves is given by: (λ = wavelength)
View SolutionFrequency of matter wave is equal to
View SolutionWhen two waves with same frequency and constant phase differenc interfere,
View SolutionIn Young's double slit experiment, The locus of the point P lying in a plane with a constant path difference between two interfering waves is
View SolutionIf path difference between two interfering waves is zero, then the point will be
View Solution
