In a LR circuit of 3 mH dinductance and $4 Ω$ resistance, emf $E = 4 \cos 1000 t$ volt is applied. The amplitude of current is

$0.8 Å$

$\frac{4}{7} Å$

$1.0 Å$

$\frac{4}{\sqrt{7}} Å$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

An e.m.f. $E = 4 cos (1000 t)$ volt is applied to an $L R$ circuit of inductance $3 m H$ and resistance $4 o h m$ . The amplitude of current in the circuit is
A $\frac{4}{\sqrt{7}} A$
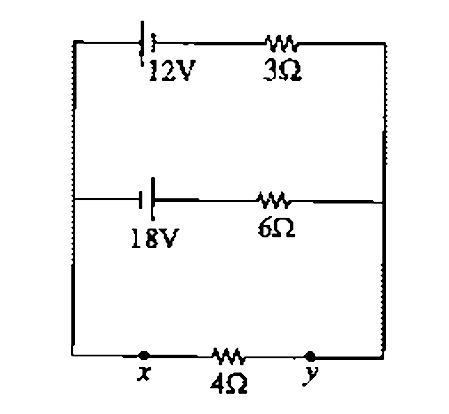
B $1.0 A$
C $\frac{4}{7} A$
D $0.8 A$
In the circuit shown the currents in $4 Ω$ and $8 Ω$ resistances are

A $2.5 A, 0 A$
B $5 A, 0 A$
C $2.5 A, \frac{40}{3} A$
D $5 A, \frac{80}{3} A$
In ac circuit contains a pure capacitor, across which an ac emf
$e = 100 sin (1000 t)$ , volt
is applied. If the peak value of the current is 200 mA, then the value of the capacitor is
A $2 μ$
B $20 μ F$
C $5 μ F$
D $500 μ F$