Level 1 from ARIHANT PHYSICS Chapter 6 GEOMETRICAL OPTICS for Class 12
| Class 12 | PHYSICS |
| Chapter | GEOMETRICAL OPTICS |
| ARIHANT PHYSICS | Level 1 |
| Medium | English |
| Academic Year | 2023-2024 |
Question 2
Light travels in a straight line. This principle is illustrated in a pinhole camera. In this simple device the image of an object is formed on a photographic plate by light passing through a small hole.
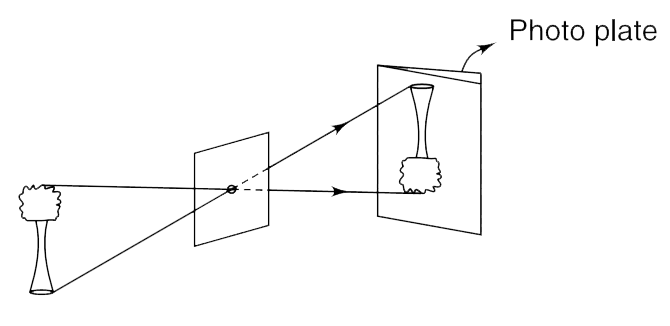
In one experiment, 5 cm high image of a tree was obtained on a photo plate placed at a distance of 15 cm from the pin hole. Actual height of the tree is 20 m.
(a) Find the distance of the pinhole from the tree.
(b) How is the size of image affected if the photo plate is moved away from the pinhole?
(c) What will happen if a large hole is made in place of a pin hole? View SolutionQuestion 3
A point object O is kept in front of a plane mirror AB having length L = 2 m. The line AOM makes an angle θ=60° with the mirror. An observer is walking along the line XMX' (perpendicular to AOM). Find the length of his path along XMX' in which he can see the image of the object. Given AO=d=1m and AM=2d.
]View SolutionQuestion 5
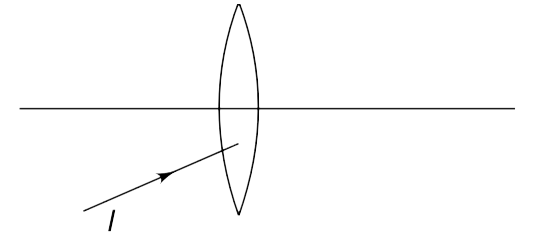
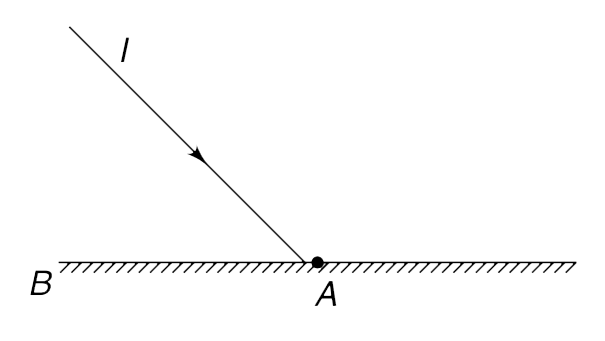
I is a ray incident on a plane mirror. Keeping the incident ray fixed, the mirror is rotated by an angle θ about an axis passing through A perpendicular to the plane of the Fig. show that the refracted ray rotates through an angle 2θ. Does your answer differ if the mirror is rotated about an axis passing through B?
 View Solution
View SolutionQuestion 6
The Fig. shows a device used to measure small twist in a thread. A plane mirror is suspended from a twist free thread. A light ray striking the mirror is reflected on to a screen placed at a distance D=1m from the mirror.
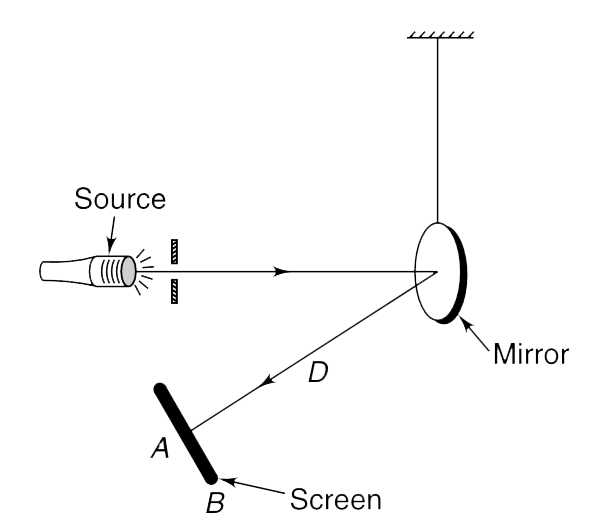
As the thread is twisted by an angle θ (so that mirror rotates by θ), the light spot on the screen moves from A to B such that AB=0.5cm. Find θ. View SolutionQuestion 7
While looking at her face in a mirror, Hema notes that her face is highly magnified when she is close to the mirror. As she backs away from the mirror, her image first gets blurry, then disappears when she is at a distance of 45 cm from the mirror. Explain the happenings? What will happen if she moves beyond 45 cm distance from the mirror?View SolutionQuestion 8
We know that parallel light rays which are inclined to the principal axis of a spherical mirror, after reflection converge at a point in the focal plane of the mirror. With this knowledge explain how you will trace the reflected ray for incident ray PQ shown in the Fig. F is focus and C is centre of curvature of the mirror.
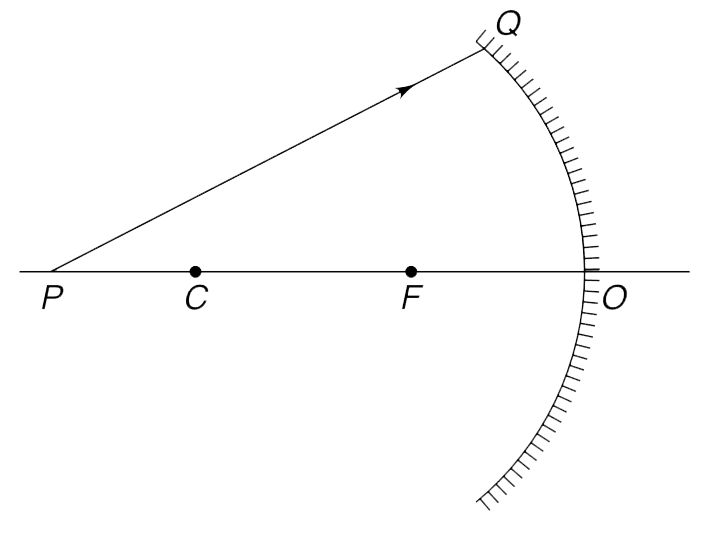 View Solution
View SolutionQuestion 9
The inner surface of the wall of a sphere is perfectly reflecting. Radius of the sphere is R. A point source S is placed at a distance R/2 from the centre of the sphere. Consider the reflection of light from the farthest wall followed by reflection from the nearest wall. Where is the image of the source? Consider paraxial rays only.View SolutionQuestion 10
A concave mirror forms a real image, on a screen of thrice the linear dimension of a real object placed on its principal axis. The mirror is moved by 10 cm along its principal axis and once again a sharp image of the object is obtained on the screen. This time the image is twice as large as the object. Find the focal length of the mirror.View SolutionQuestion 12
A point object O is placed at a distance of 60 cm from a concave mirror of radius of curvature 80 cm.
(a) At what distance from the concave mirror should a plane mirror be kept so that rays converge at O itself after getting reflected from the concave mirror and then from the plane mirror?
(b) Will the position of the point where the rays meet change if they are first reflected from the plane mirror? View SolutionQuestion 15
A small insect starts walking away from a concave mirror along its principal axis. At a point (P) 20 cm from the mirror the image flips upside down. (a) What can you say about the size of the image at the instant it flips upside down – it is very large, very small or of the size similar to the insect? (b) Find the distance of the insect from point (P) where its image is thrice as large as the insect. View SolutionQuestion 16
A piece of metal is cut from a hollow metal sphere of radius R and is polished on both sides. A boy looks at the metal piece and finds his image 13 cm behind the metal piece. His friend flips the mirror, keeping its position unchanged and now the boy finds his image to be 52 cm behind the mirror. Find R.View SolutionQuestion 17
The aperture diameter of a spherical mirror is D=ηR where η is a positive number less than 2 and R is radius of curvature of the mirror. Consider a wide parallel beam of light incident on the mirror parallel to its principal axis.
(a) Find minimum value of h for which marginal rays start getting reflected twice.
(b) Find minimum value of h for which marginal rays undergo three reflections.View SolutionQuestion 18
A source of laser (S), a receiver (R) and a fixed mir- ror (F) – all lie on an arc of a circle of radius R=0.5km. The distance between the source and the receiver is d=0.5m. At the centre of the circle there is a small mirror M which is rotating with angular speed ω (see figure). Find smallest value of ω is if it is seen that the source shoots a laser pulse which gets reflected at M, then gets reflected at F and finally gets reflected at M to be received by the receiver.
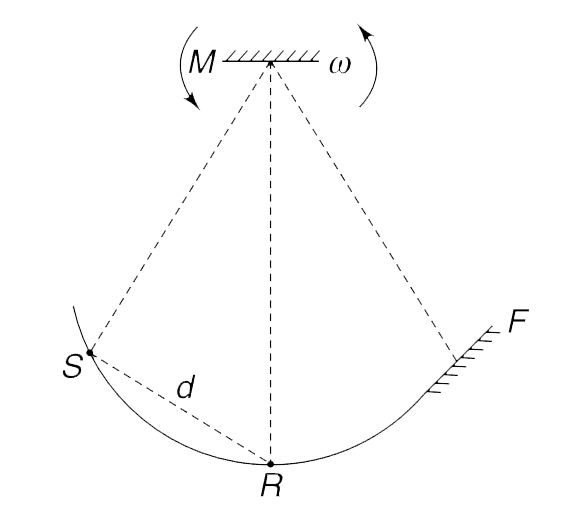 View Solution
View SolutionQuestion 19
A real object is kept at a distance a from the focus of a concave mirror on the principal axis. A real image is formed at a distance b from the focus. Plot the variation of b with a. If b versus a graph is given to you, how will you find the focal length of the mirror? ExplainView SolutionQuestion 20
A light ray enters horizontally into a vertical cylinder through a small hole at A. The ray is initially travelling along a chord (AB) whose length is (√5+12)R where R is the radius of the cylinder. After how many reflections on the inner wall of the cylinder the light ray will be incident at point A ? [Given cos36∘=√5+14]
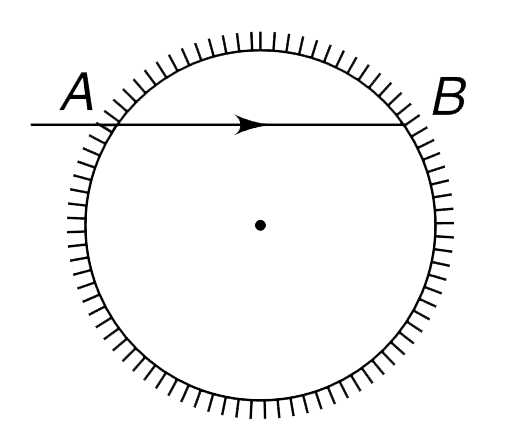 View Solution
View SolutionQuestion 26
A glass block of refractive index μ=1.5 has an L cross section with both arms identical. A light ray enters the block from left at an angle of incidence of 45°, as shown in the figure. If the block was absent the ray would pass through the point P. Calculate the angle at which the ray will emerge from the bottom face after refraction through the block.
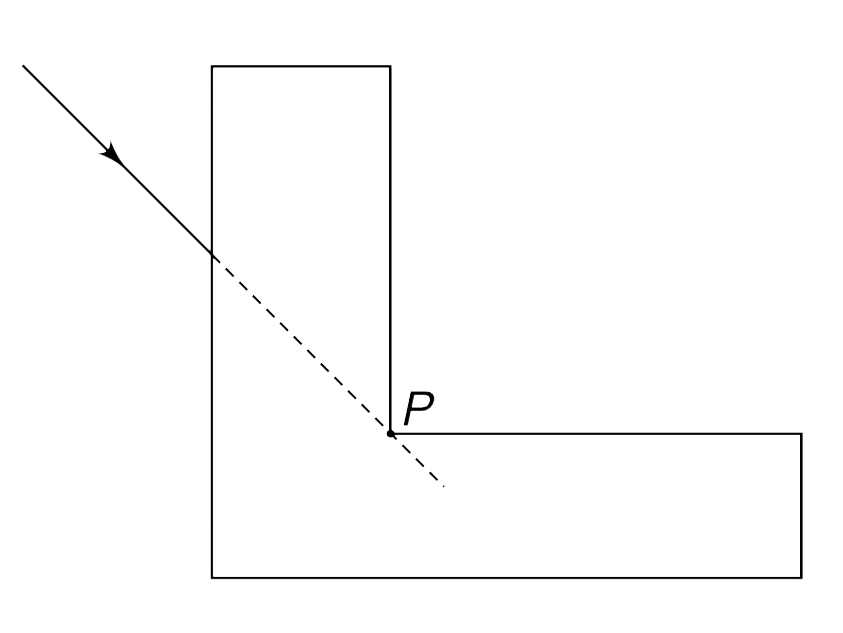 View Solution
View SolutionQuestion 28
A ray of light travelling in air is incident on a composite transparent slab at an angle of incidence i=45°. The composite slab consist of 100 parallel faced slices of equal thickness. The refractive index of the nth slice (counting from the one on which light is incident) is given by μn=1.0+0.01n. The medium on the other side of the slab has refractive index of √2 . Calculate the angular deviation suffered by the ray as it comes out of the slab.View SolutionQuestion 30
A large transparent cube (refractive index = 1.5) has a small air bubble inside it. When a coin (diameter 2 cm) is placed symmetrically above the bubble on the top surface of the cube, the bubble cannot be seen by looking down into the cube at any angle. However, when a smaller coin (diameter 1.5 cm) is placed directly over it, the bubble can be seen by looking down into the cube. What is the range of the possible depths d of the air bubble beneath the top surface ?
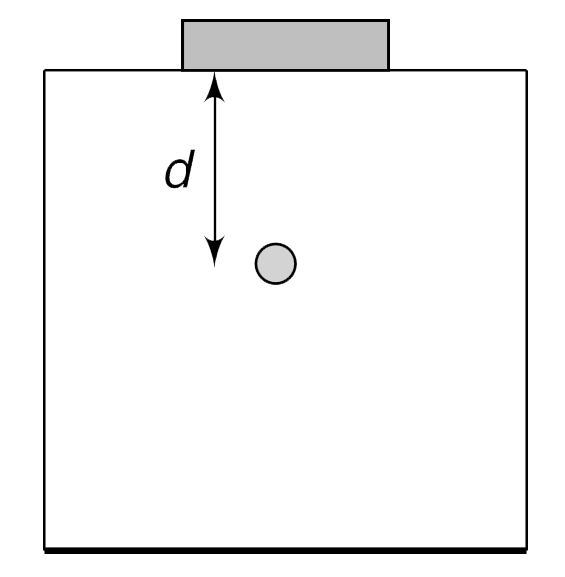 View Solution
View SolutionQuestion 31
A travelling microscope can move vertically along a scale. It is focused at a mark O on the table and the reading on the vertical scale isr1. Now a glass slab is placed over mark O and the microscope has to be moved up to bring the mark in focus again. This time the scale reads r2. Lycopodium powder is spread over the top of the glass slab and the microscope is moved up once again to bring the powder particles in sharp focus. This time the vertical scale reads r3. Find the refractive index of the material of the glass slab.
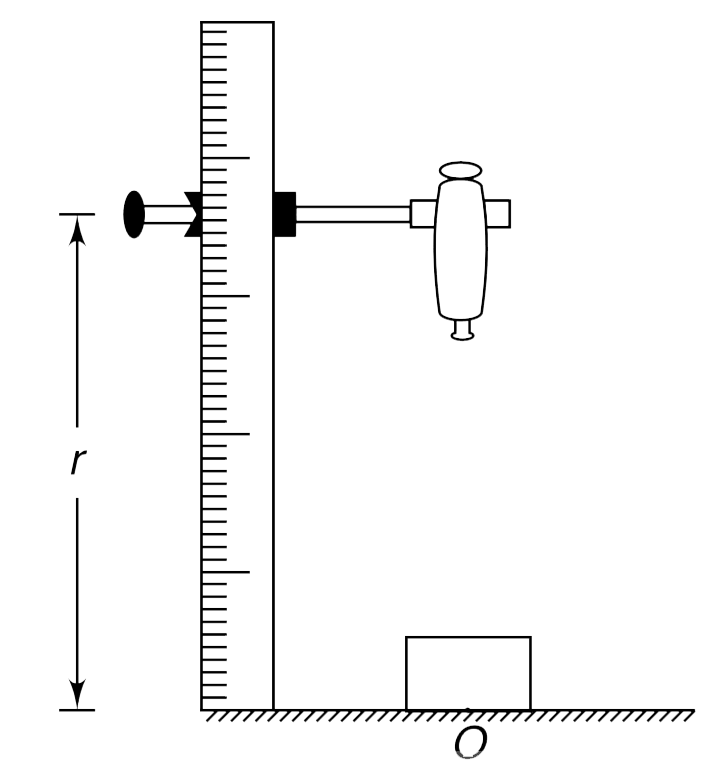 View Solution
View SolutionQuestion 32
A vertical beam of light of cross sectional radius R2 is incident symmetrically on the curved surface of a glass hemisphere of refractive index μ=32. Radius of the hemisphere is R and its base is on a horizontal table. Find the radius of luminous spot formed on the table. sin20∘=13 and sin80∘=0.98View SolutionQuestion 33
A horizontal cylindrical tank is half full of water ( refractive index =43) . The space above the water is filled with a light liquid of unknown refractive index (μ). A small laser source (s) can move along the curved bottom of the cylinder and aims a light beam towards the centre of the cylinder. The time needed by the laser beam to travel from the source to the rim of the cylinder depends on position (θ) of the source as shown in the graph. Find μ, it is given that sinθ0=56.
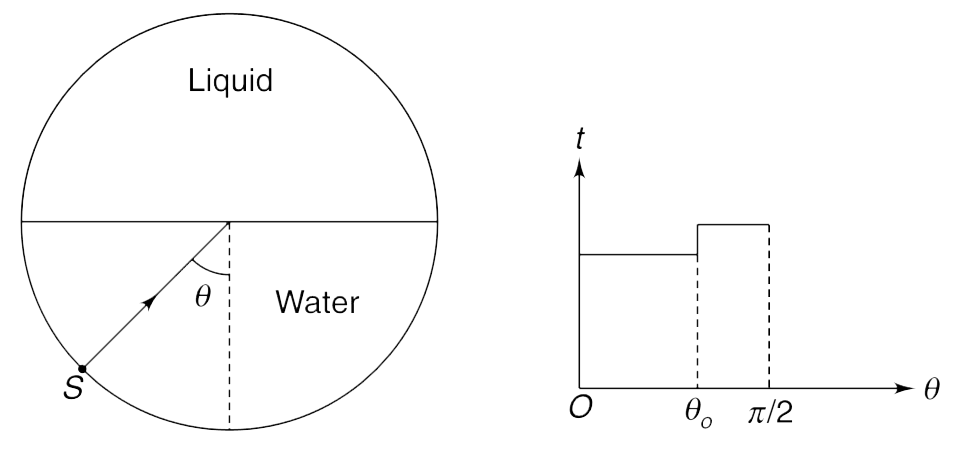 View Solution
View SolutionQuestion 34
A man standing on sea-shore sees an elongated image (shown by dashed line) of a floating object AB. In fact he finds the image to be oscillating due to air turbu- lence. Figure (ii) gives three plots (a, b and c) of height from the water surface vs air temperature. Which one best illustrates the air-temperature condition that can create this image? [Many people have seen sea monsters due to this phenomena!]
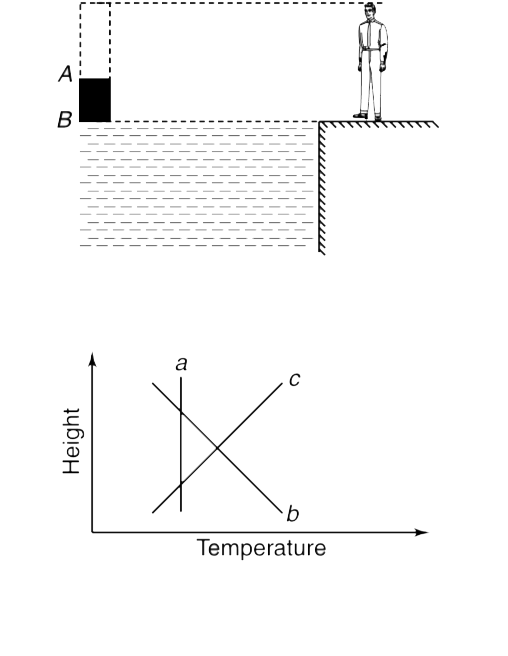 View Solution
View SolutionQuestion 36
A glass slab is placed between an object (O) and an observer (E) with its refracting surfaces AB and CD perpendicular to the line OE. The refractive index of the glass slab changes with distance (y) from the face AB as μ=μ0(1+y). Thickness of the slab is t. Find how much closer (compared to original distance) the object appears to the observer. Consider near normal incidence only.
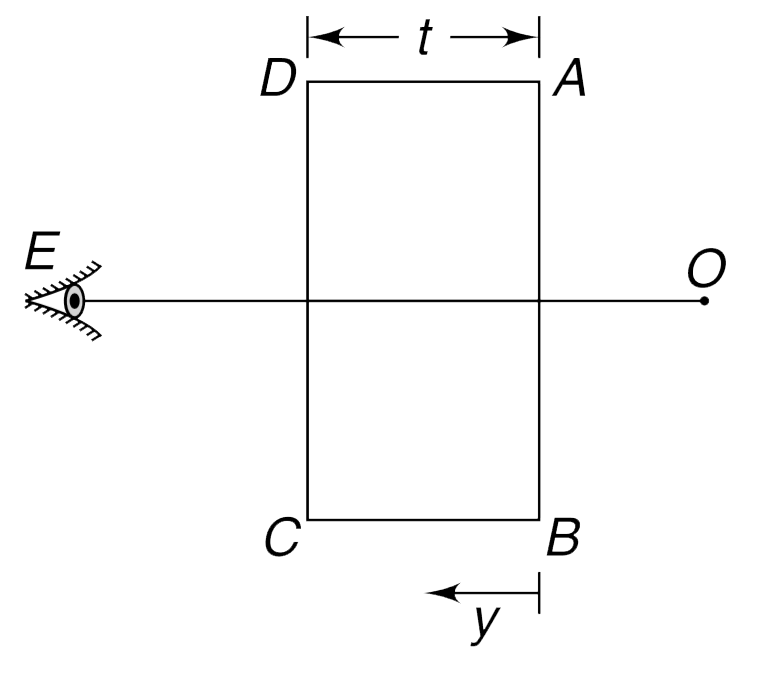 View Solution
View SolutionQuestion 38
An equilateral glass prism can produce a minimum deviation of 30° to the path of an incident ray. A transpar- ent slab of refractive index 1.5 is placed in contact with one of the refracting faces of the prism. Thickness of the slab is 3 cm. Now calculate the minimum possible deviation which can be produced by this prism-slab combination.
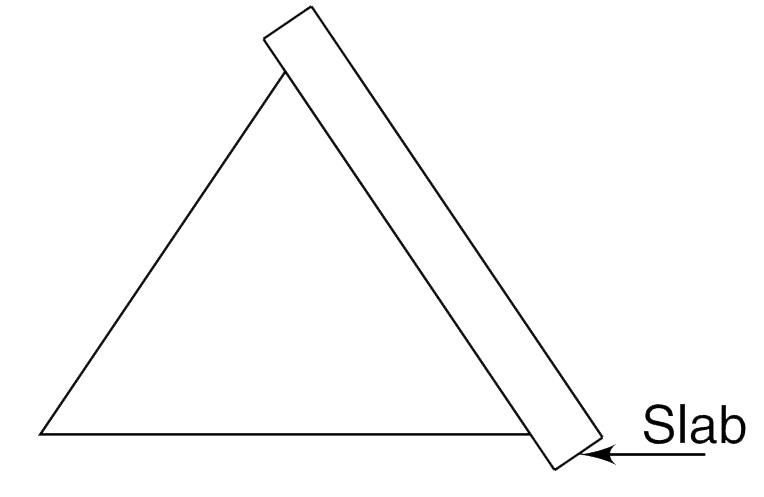 View Solution
View SolutionQuestion 39
A triangular medium has varying refracting index μ=μ0+ax where x is the distance (in cm) along x-axis from origin and m0=4/3. A ray is incident normally on face OA at the mid-point of OA. Find the range of value of a so that light does not escape through face AB when it falls first time on the face AB.
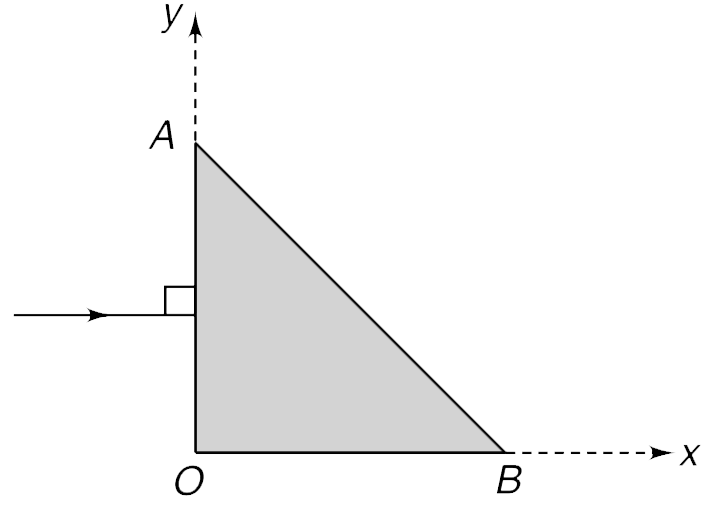 View Solution
View SolutionQuestion 40
Letter F is kept in front of a right triangular psim. The light rays enter perpendicular to the large rectangular face, is reflected twice by small rectangular faces and exits perpendicularly to the large rectangular face (see Fig.). Draw the image of the letter seen by the eye.
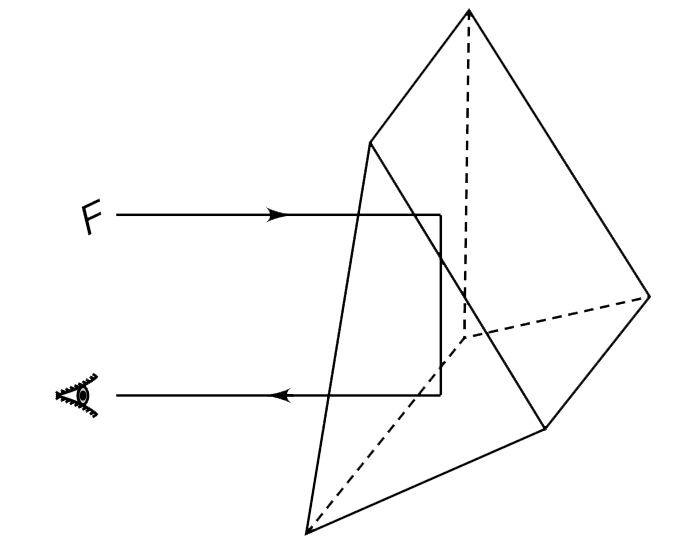 View Solution
View SolutionQuestion 42
Limiting angle of a prism is defined as the largest angle of the prism (A) for which no emergent ray is obtained.
(a) Find the limiting angle (A0) for a glass prism having refractive index μ.
(b) A prism has limiting angle for light of wavelength λ0. Can there be any emergent ray for light of wave- length λ<λ0.View SolutionQuestion 43
An isosceles glass prism has one of its faces silvered. A light ray is incident normally on the other face which is identical in size to the silvered face. The light ray is reflected twice on the same sized faces and emerges through the base of the prism perpendicularly. Find the minimum value of refractive index of the material of the prism.View SolutionQuestion 44
A parallel beam of light falls normally on the first face of a prism of small refracting angle. At the second refracting face it is partly reflected and partly transmitted. The reflected light strikes the first face again and emerges from it making an angle of 4° with the reversed direction of incident beam. The deviation suffered by refracted ray is 1° from original direction of incident ray. Find the refractive index of glass of the prism and the angle of the prism. View SolutionQuestion 48
Object O is placed in front of a plane mirror M. A glass slab S having thickness t = 3 cm and refractive index μ=1.8 is placed between the object and the mirror. The refracting faces of the slab are parallel to the mirror surface. The object is made to move with a velocity of u=2m/s perpendicular to the mirror surface. Find the speed of the image formed after
(a) refraction from the slab followed by reflection from the mirror.
(b) refraction from the slab followed by reflection from the mirror followed by the refraction from the slab.
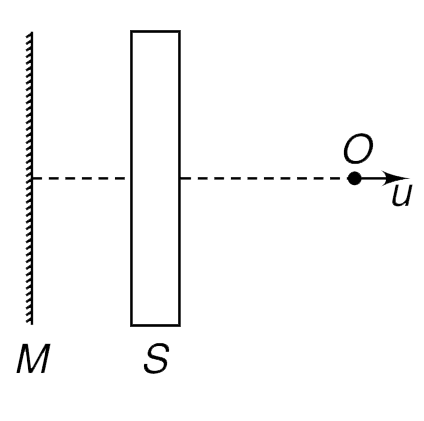 View Solution
View SolutionQuestion 49
A point object O is placed at a distance of 62 cm in front of a concave mirror of focal lengthf=20cm. A glass slab of refractive index μ=32 and thickness 6 cm is inserted between the object and the mirror. Let’s define final image as image formed after the light ray originating from O passes through the slab, gets reflected from the mirror and then again passes through the slab. At what distance d from the mirror, the face AB of the slab can be placed so that the final image is formed inside the slab itself?
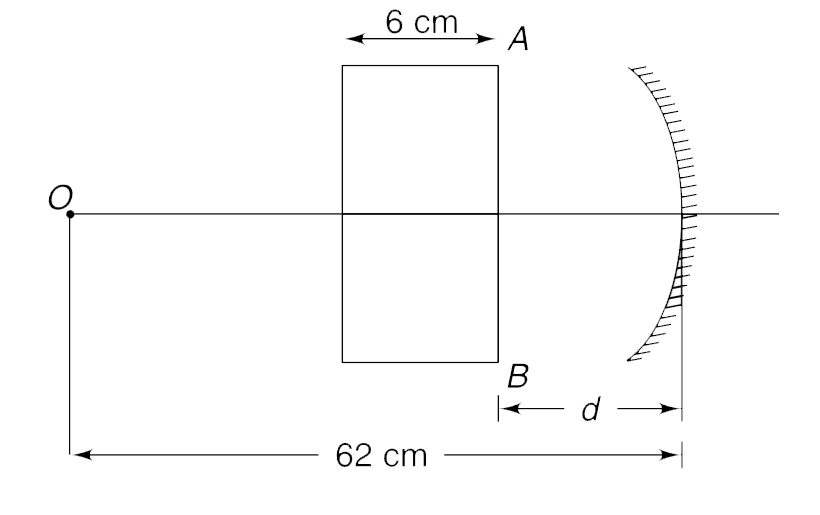 View Solution
View SolutionQuestion 50
A point object (A) is kept at a distance (L) from a convex mirror on its principal axis. A glass slab is inserted between the object and the mirror with its refracting surfaces perpendicular to the principal axis of the mirror. The thickness of the slab is 6 cm and its refractive index is 32. The image formed after refraction through the slab, reflection from the mirror followed by refraction through the slab is a virtual image at a distance of 10 cm from the pole of the mirror (on its principal axis). Consider paraxial rays only and calculate the distance (L) of the object from the mirror. Focal length of the mirror is f=20cm.
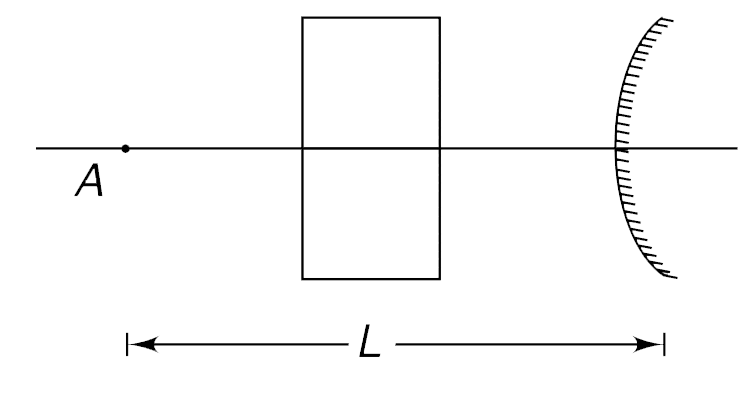 View Solution
View SolutionQuestion 52
An equilateral prism has its faces made of a transparent fiber sheet (having refractive index = 1.25) having thickness of 1 mm. The fibre prism is filled with a liquid of refractive index √2 . Find the deviation of a light ray incident on one face of such a prism at an angle of 45°.
 View Solution
View SolutionQuestion 54
Two thin prisms are combined such that they neither produce any average deviation nor do they cause any dispersion when white light is incident on the combination. Angle of one prism is A=2° and refractive index of its glass for red, yellow and violet lights are 1.49, 1.50 and 1.51 respectively. Find the dispersive power of the glass of the other prism.View SolutionQuestion 56
A concave spherical surface of radius of curvature R=20cm separates two media A and B having refractive indices μA=43 and μB=32respectively. A point object is placed on the principal axis. Find the distance of the object from the surface so that its image is virtual when
(a) the object is in medium A.
(b) the object is in medium B. View SolutionQuestion 60
A region bounding water has air on two sides. Tell the nature (real or virtual) of the image for following cases- (The object is real and lies on the principal axis (see fig) in all cases.)
(a) The object is to the left of surface 1 and the image to be considered is formed after the first refraction.
(b) The object is to the left of surface 1 and the image to be considered is formed after two refractions.
(c) The object is to the right of second surface and the image to be considered is formed after one refraction.
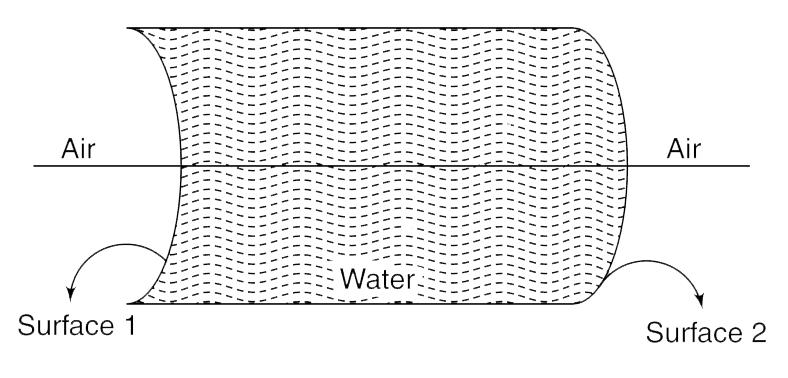 View Solution
View SolutionQuestion 61
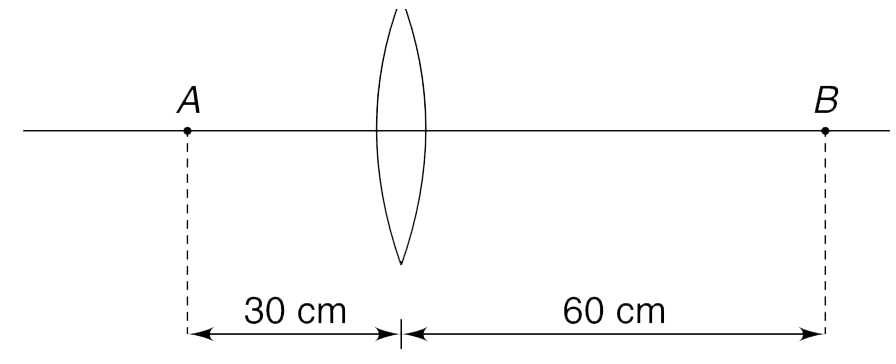
A converging beam of light rays passes through a round opening in a screen. The beam converges at a point A which is at a perpendicular distance of 15 cm from the screen and lies slightly above the central axis of the circular opening. A convex lens of focal length 30 cm is inserted in the opening. At what distance from the screen do the rays converge now ?
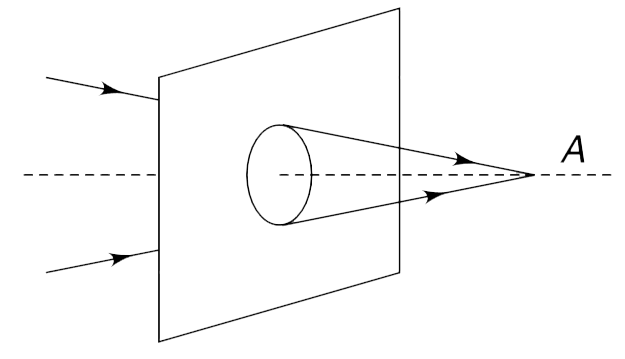 View Solution
View SolutionQuestion 62
In the Figure AB is the principal axis of an optical element (a lens or a mirror). For position 1, 2 and 3 of a real object, the corresponding position of images are 1', 2' and 3' respectively. Size of image at 3' is largest and that at 1' is smallest. Identify the optical element and indicate its position.
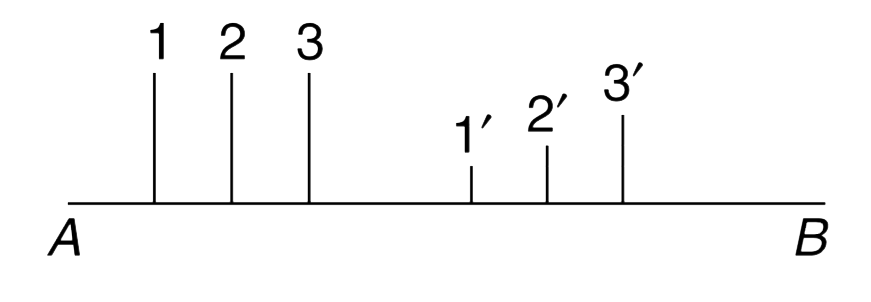 View Solution
View SolutionQuestion 63
A transparent glass slab (G) of thickness 6 cm is held perpendicular to the principal axis of a convex lens (L) as shown in the Figure. The refractive index of the material of the glass is 32 and its nearer face is at a distance 40 cm from the lens. Focal length of the lens is 20 cm. Find the thickness of the glass slab as observed through the lens.
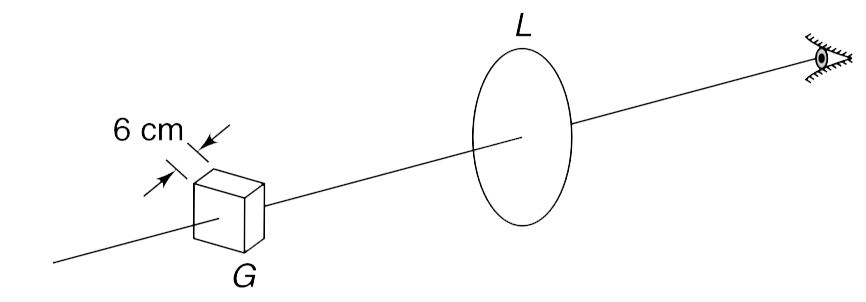 View Solution
View SolutionQuestion 64
An object 240 cm in front of a lens forms a sharp image on a screen 12 cm behind the lens. A glass slab 1 cm thick, having refractive index 1.50 is placed between the lens and the screen with its refracting faces perpendicular to the principal axis of the lens.
(a) By how much distance the object must be moved so as to again cast a sharp image on the screen?
(b) Another identical glass slab is interposed between the object and the lens. How much further the object shall be moved so as to form a sharp image on the screen. View SolutionQuestion 68
A horizontal parallel beam of light passes though a vertical convex lens of focal length 40 cm. Behind the lens there is a plane mirror making an angle θ with the principal axis of the lens. The mirror intersects the principal axis at M. Distance between the optical centre of the lens and point M is OM=20cm. The light beam reflected by the mirror converges at a point P. Distance OP is 20 cm. Find θ.
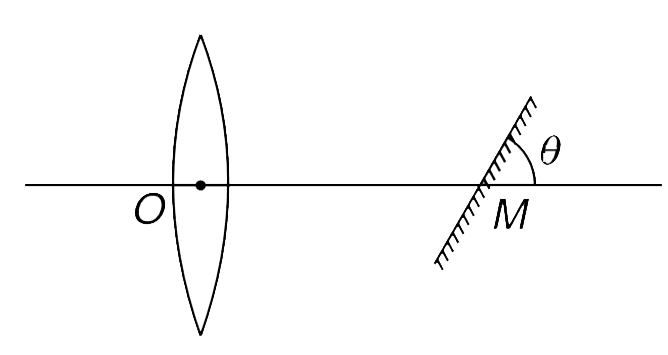 View Solution
View SolutionQuestion 69
A cylindrical tube has a length of 60 cm. Three identical convex lenses, each of focal length f=10 cm are fixed inside the tube, one at each of the ends and one at the centre. One end of the tube is placed 10 cm away from a point source. The device casts an image of the object. How much does the image shift when the tube is moved away from the source by 10 cm. View SolutionQuestion 70
There is an air lens in an extended glass medium. The radius of curvature of both the curved surfaces is R, and refractive index of the glass is 32 . Power of this air lens is P. Find the refractive index of the material to be filled inside the lens so that its power becomes – P.
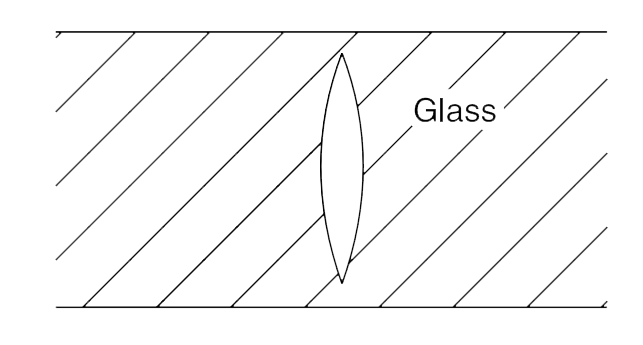 View Solution
View SolutionQuestion 71
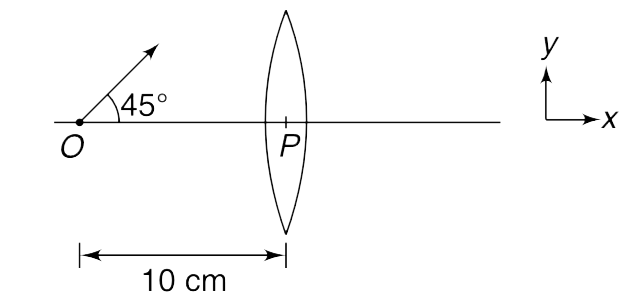
A thin converging lens forms a real image of an object located far away from the lens. The image is formed at A at a distance 4x from the lens and height of the image is h. A thin diverging lens of focal length x is placed at B[PB=2x] and a converging lens of focal length 2x is placed at C[PC=3x]. The principal axes of all lenses coincide. Find the height of the final image formed.
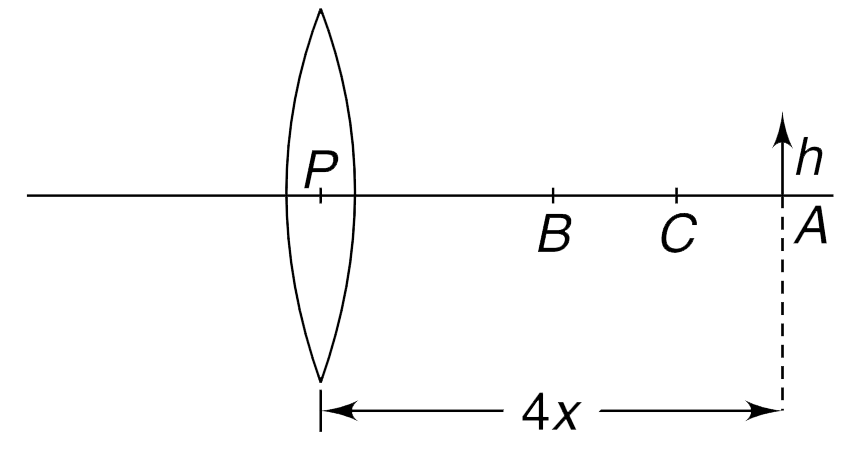 View Solution
View SolutionQuestion 74
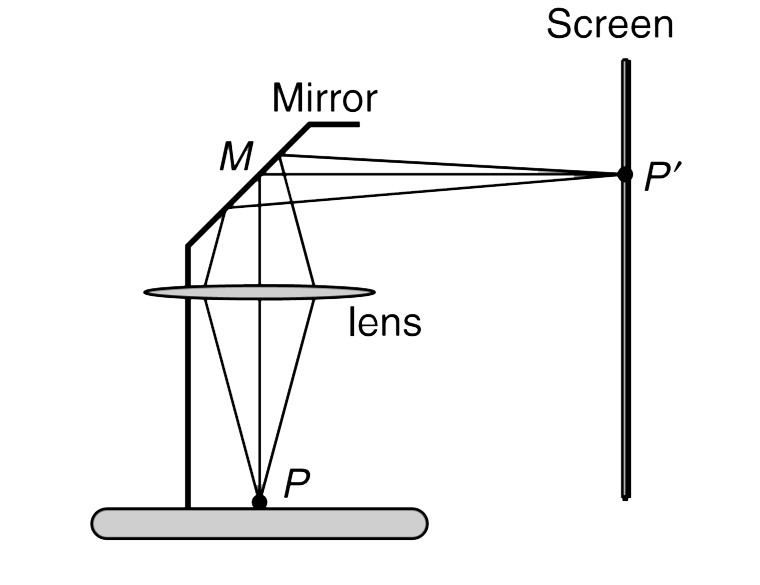
A small object is at the bottom of a container which has water filled up to a height of 20 cm. There is a plane mirror inclined at 45° to the horizontal above the container. A convex lens having focal length 15 cm is at a distance of 50 cm from the mirror. The horizontal principal axis of the lens is at a distance of 45 cm from the bottom of the container. Find the distance of the image (from the lens) of the object as seen by an observer to the left of the lens. Light rays from the object hit the lens only after they are reflected from the mirror. (use μwater=4/3)
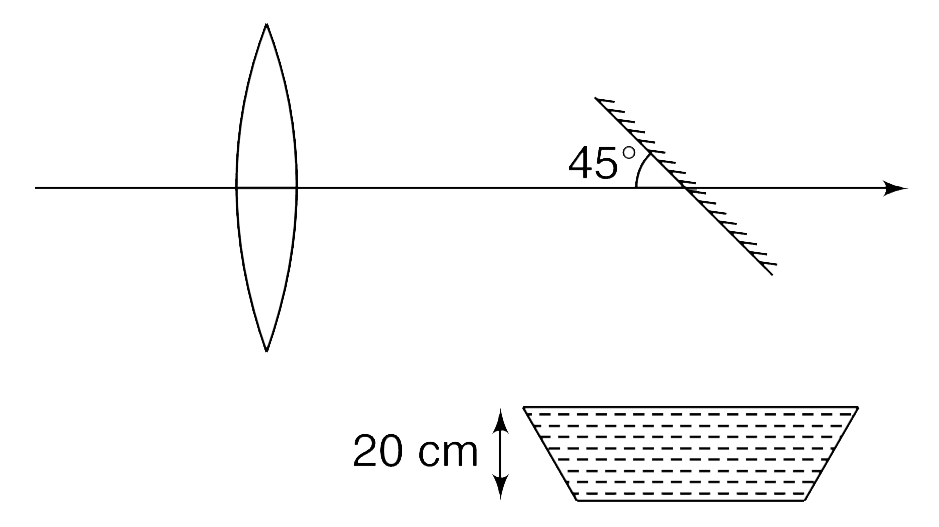 View Solution
View Solution