Exercise from ARIHANT PHYSICS Chapter 6 GRAVITATION for Class 11

Question 1

Question 2

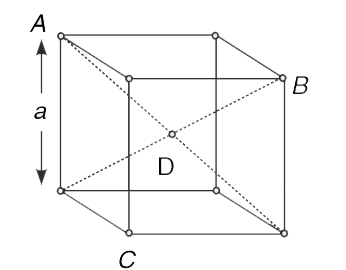
Four identical point masses m each are kept at the vertices A, B, and C of a cube having side length ‘a’ (see figure). Another identical mass is placed at the center point D of the cube. (a) Where will you place a fifth identical mass so that the net gravitational force acting on mass at D becomes zero? (b) Calculate the net gravitational force acting on the mass at D.

Question 3

Question 4

Question 5

Question 6

Question 7

Question 8

Question 9

Question 10

Question 11

Question 12

Question 13

Question 14

Question 15

Question 16

Question 17

Question 18

Question 19

Question 20

Question 21

Question 22

Question 23

Question 24

Question 25

Question 26

Question 27

Question 28

A small asteroid is at a large distance from a planet and its velocity makes an angle phi(cancel(=)0) with line joining the asteroid to the centre of the planet. Prove that such an asteroid can never fall normally on the surface of the planet.

Question 29

Question 30

Imagine a hole drilled along the radius of the earth. A uniform rod of length equal to the radius (R) of the earth is inserted into this hole. Find the distance of centre of gravity f the rod from the centre .

Question 31

Question 32

Question 33

Question 34

A tunnel is dug along a chord of non rotating earth at a distance $d=\frac{R}{2}$ [R = radius of the earth] from its centre. A small block is released in the tunnel from the surface of the arth. The block comes to rest at the centre (C) of the tunnel. Assume that the friction coefficient between the block and the tunnel wall remains constant at $\mu .$
Calculate work done by the friction on the block. Calculate $\mu .$

Question 35

Question 36

Question 37

Question 38

Question 39

Question 40

Assume that there is a tunnel in the shape of a circular arc through the earth. Wall of the tunnel is smooth. A ball of mass m is projected into the tunnel at A with speed v. The all comes out of the tunnel at B and escapes out of the gravity of the earth. Mass and radius of the earth are M and R respectively and radius of the circle shaped tunnel is also . Find minimum possible value of v (call it $v_0$) If the ball is projected into the tunnel with speed $v_0$, calculate the normal force applied by the tunnel wall on the ball when it is closest to the centre of the earth. It is given that the closest distance between the ball and the centre of the earth is $\frac{R}{2}$

Question 41

Question 42

Question 43

Question 44

Question 45

Question 46

Question 47

The astronomical phenomenon when the planet Venus passes directly between the Sun and the earth is known as Venus transit. For two separate persons standing on the arth at points M and N the Venus appears as black dots at points M’ and N’ on the Sun. The orbital period of Venus is close to 220 days. Assuming that both earth and Venus revolve on circular paths and taking distance MN = 1000 km, calculate the distance M’N’ on the surface of the Sun.$[\text{Take}{\left(2.75\right)}^{1/3}=1.4]$

Question 48

Satellite A is following a circular path of radius a around the earth another satellite B follows an elliptical path around the earth. The two satellites have same mechanical energy and their orbits intersect. Find the speed of satellite B at the point where its path intersects with the circular orbit of A. Take mass of earth to be M.

Question 49

Question 50

Question 51

A small asteroid is approaching a planet of mass M and radius R from a large distance. Initially its velocity (u) is along a tangent to the surface of the planet. It fall on the surface making an angle of ${30}^2$ with the vertical. Calculate u.

Question 52

An asteroid was fast approaching the earth. Scientists fired a rocket which hit the asteroid at a distance of 5 R from the centre of the earth (R = radius of the earth). mmediately after the hit the asteroid’s velocity $\left(V_0\right)$ was making an angle of $\theta ={30}^{\circ }$ with the line joining the centre of the earth to the asteroid. The asteroid just grazed past the surface of the earth. Find $V_0$ [Mass of the earth = M]

Question 53

Question 54

Question 55

Question 56

Question 57

Question 58

Question 59

Question 60

Question 61

Question 62

Question 63

Question 64

A near surface earth satellite has cylindrical shape with cross sectional area of$s=0.5M^2$ and mass of M = 10 kg. It encounters dust which has density of $D=1.6XX{10}^{-11}KG/m^s.$ Assume that the dust particles are at rest and they stick to the satellite’s front face on collision. Take mean density of earth to be$\rho =5500kg/m^3$Find the drag force experienced by the satelliten
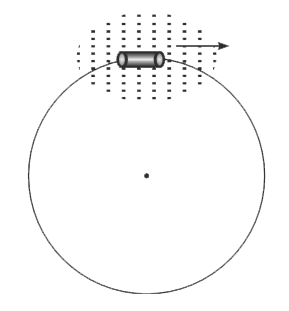If the dust extends throughout the orbit, find the change in velocity and radius of the circular path of the satellite in one revolution.