MOLE CONCEPT for Class 11 CHEMISTRY
INTRODUCTION
Chemistry is the study of the materials that make up the universe and the changes which these materials undergo. The study deals with the composition, structure and properties of matter. These aspects can be best understood in terms of the constituents of matter atoms and molecules. In fact, chemistry is the science of atoms and molecules.
Chemistry is defined as :
The branch of science which deals with the study of composition, structures and properties of matter and the changes which the matter undergoes under different conditions and the laws which govern these changes.
Chemistry plays a central role in science and is often interlinked with other branches of science. It also plays an important role in our daily life.
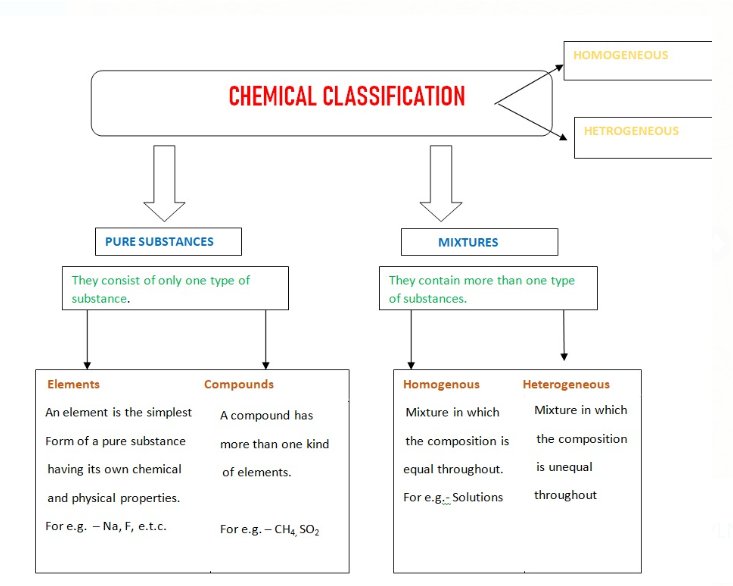
Matter and Its Classification
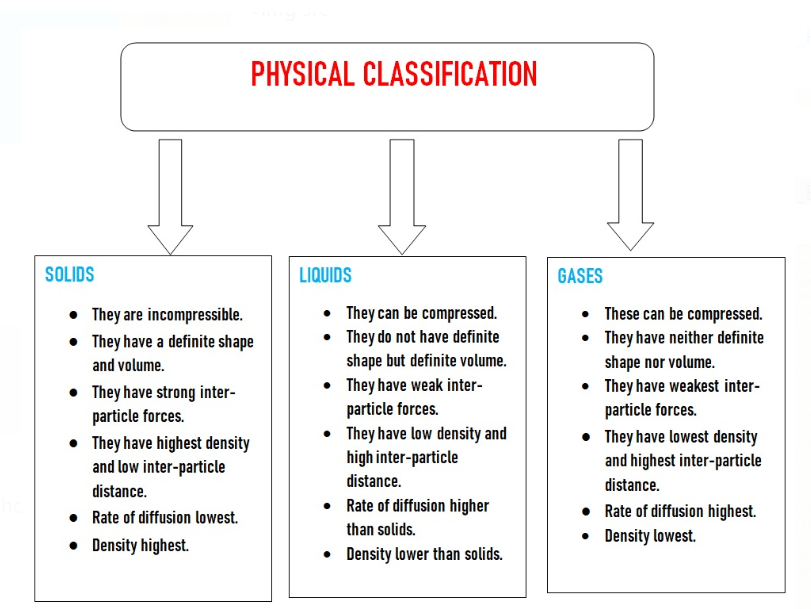
Matter has two kinds of classification :
(i) Physical Classification
(ii) Chemical Classification
The physical classification is on the basis of forces between their particles.
The chemical classification is further divided in the following manner :
Different Types of Masses
Atomic Mass : Atomic masses ka present system Carbon−12 par based hai (Carbon−12 one of the isotopes of carbon hai aur isko hum C12 form meh represent karte hai). Ab kisi element ka atomic mass, wo mass hota hai jo atom of carbon jiska mass 12 hai uss se number of times heavier hota hai.
In other words, one atomic mass wo mass hota hai jo exactly one-twelfth of the mass of one carbon−12 atom ke equal hota hai.
Since mass of 1 atom of C−12=1.9924×10−23 g
1/12th part of the mass of 1 atom =1.9924×10−23g12=1.67×10−24g=16.022×1023g
For example : Mass of an atom of hydrogen =1.6736×10–24 g
Thus, in terms of amu, the mass of hydrogen atom =1.6736×10−24/1.66056×10−24 =1.0078 amu ≈1.0080 amu
Atomic mass of an element ko jab hum grams meh express karte hai tab hum isko gram atomic mass bolte hai.
= mass of NA atoms = mass of 6.023×1023 atoms
Molecular Mass : Molecular mass meh hum ek molecule ke sabhi elements ke atomic masses ka sum karte hai. Isko obtained karne ke liye hum each element ke number ko inke atomic masses se multiply karte hai, further inn sabko add karte hai. For example
Molecular mass of Sulphur Dioxide
=32 u +2×16 u =64 u
(Here we have written 2 because there are 2 atoms of oxygen).
Molar mass of Ferric Oxide
=2×56 u +3×16 u =160 u
Molecular mass of the substance ko jab hum grams meh expressed karte hai tab hum isse gram molecular mass bolte hai.
= mass of NA molecules = mass of 6.023×1023 molecules
For example : The gram molecular mass of H2SO4 is 98 gram and Molecular mass of H2SO4 is 98 u
Average Atomic Mass : Jab hum kisi element ke isotopes ko inki natural abundance se multiply kar inka sum karte hai, tab isse hum average atomic mass bolte hai.
.png)
(This Formula is Valid for percentage Abundance)
For Example : Chlorine has two isotopes of atomic mass units 34.97 and 36.97. The relative abundances of these two isotopes are 0.755 and 0.245 respectively. Find the average atomic mass of chlorine.
Solution : Average atomic mass =34.97×0.755+36.97×0.245=35.46
Ab periodic table of elements meh jo different elements ke atomic masses mentioned hote hai wo actually inke average atomic masses ko represent karte hai.
Formula Mass : In compounds jaise sodium chloride meh ek Na+ ion ko 6 Cl− ions surround karte hai aur vice versa. Ab formula jaise NaCl ko formula mass calculate karne ke liye use kiya jata hai instead of molecular mass kyunki solid state meh sodium chloride ek single entity ki tarah exist nahi karta hai.
Thus, sodium chloride ke formula mass ke liye hum atomic mass of sodium + atomic mass of chlorine consider karte hai.
=23.0 u +35.5 u =58.5 u
Also, jab hum formula mass ko gram meh express karte hai tab isse hum gram formula mass bolte hai.
Different Types of Masses
Atomic Mass : The present system of atomic masses is based on Carbon−12 (Carbon−12 is one of the isotopes of carbon and can be represented as C12). The atomic mass of an element is the number of times an atom of that element is heavier than an atom of carbon taken as 12.
One atomic mass unit is defined as a mass exactly equal to one-twelfth of the mass of one Carbon−12 atoms
Since mass of 1 atom of C−12=1.9924×10−23 g
1/12th part of the mass of 1 atom =1.9924×10−23g12=1.67×10−24g=16.022×1023 g
For example : Mass of an atom of hydrogen =1.6736×10–24 g
Thus, in terms of amu, the mass of hydrogen atom =1.6736×10−24/1.66056×10−24=1.0078 amu ≈1.008 amu
When atomic mass of an element is expressed in grams then it is known as gram atomic mass
= mass of NA atoms = mass of 6.023×1023 atoms
Molecular Mass : Molecular mass is the sum of atomic masses of the elements present in a molecule. It is obtained by multiplying the atomic mass of each element by the number of its atoms and adding them together. For example
Molecular mass of Sulphur Dioxide
(Here we have written 2 because there are 2 atoms of oxygen).
Molar mass of Ferric Oxide
=2×56 u +3×16 u =160 u
When molecular mass of the substance is expressed in grams then it is known as gram molecular mass.
= mass of NA molecules = mass of 6.023×1023 molecules
For example : The gram molecular mass of H2SO4 is 98 gram and Molecular mass of H2SO4 is 98 u.
Average Atomic Mass : The average atomic mass of an element is the sum of the masses of its isotopes, each multiplied by its natural abundance.
.png)
(This Formulae is Valid for percentage Abundance)
For Example : Chlorine has two isotopes of atomic mass units 34.97 and 36.97. The relative abundances of these two isotopes are 0.755 and 0.245 respectively. Find the average atomic mass of chlorine.
Solution : Average atomic mass =34.97×0.755+36.97×0.245=35.46
In the periodic table of elements, the atomic masses mentioned for different elements actually represent their average atomic masses.
Formula Mass : In Compounds like sodium chloride, one Na+ ion is surrounded by six Cl– ion and vice-versa. The formula, such as NaCl, is used to calculate the formula mass instead of molecular mass as in the solid state sodium chloride does not exist as a single entity.
Thus, the formula mass of sodium chloride is atomic mass of sodium + atomic mass of chlorine
=23.0 u +35.5 u =58.5 u
When formula mass of the substance is expressed in grams then it is known as gram formula mass.
Mole Concept : In terms of mass, number of particles, volume
Ab microscopic level (i.e, atoms, molecules, particles, electrons, ions, etc.,) par entities ko count karne ke liye hum mole ko use karte hai.
Mole (symbol mol) seventh base quantity for the amount of the substance hai.
One mole of a substance wo amount hota hai jisme utne hi particles or entities present hote hai jitne atoms exactly 12 g C12 isotope meh hote hai.
Or ek mole of substance meh Avogadro number (6.022×1023) of particles hote hai.
Ab hume mole concept samajhne ke liye Avogadro’s number ke basic ko samajhna hoga.
Avogadro’s no. : Element ke 1 gram atom meh number of atoms or substance ke 1 gram molecule meh number of molecules ko hum Avogadro’s number bolte hai.
Note : Accordingly one cannot say one mole of hydrogen, yahan ye specify karna jaruri hai whether its one mole of hydrogen atom or one mole of hydrogen molecule.
Ab hum molar mass ko in terms of mole define kar sakte hai - One mole of a substance ke mass in grams ko hum molar mass bolte hai.
Ab mole ko hum various ways meh define kar sakte hai :
(a) In terms of Mass : Jab hum given mass of substance ko iske atomic or molecular mass se divide karte hai toh ye hume uss substance ke no. of moles deta hai.
For example : 1 mole of C= Atomic mass of Carbon =12 g of Carbon
1 mole of Sulphur = Atomic mass of Sulphur =32 g of Sulphur
1 mole of CH4= Molecular mass of CH4
= Atomic mass of C+ Atomic mass of Hydrogen ×4
=12 g +4 g =16 g
(b) In terms of Number of Particles : Yahan particles meh ions, electrons, atoms, molecules involved hote hai. Ab agar substance atomic ho tab mole wo amount of substance hota hai jisme Avogadro’s number (6.022×1023) of atoms hote hai or jab substance molecular ho tab mole wo amount of substance hota hai jisme Avogadro's number (6.022×1023) of molecules hote hai.
For example : 1 mol of hydrogen atoms =6.022×1023 atoms
1 mol of water molecules =6.022×1023 water molecules
(c) In terms of Volume : Ab gases ke case meh ek mole wo amount of gas hota hai jisme 22.4 litres of volume hoti hai at STP.
For example : 1 Mole of CO2 gas =22.4 litres of carbon dioxide gas at STP
1 Mole of oxygen gas =22.4 litres of oxygen at STP
Note : STP (Standard Temperature and Pressure) :
When Temperature =0∘C or 273.15 K, Pressure =1 atm, Molar Volume =22.4 L or
When Temperature =0∘C or 273.15 K, Pressure =1 bar, Molar Volume =22.7 L
Importance of Avogadro's Number and Mole Concept : Chemical calculations meh Avogadro’s number aur mole concept number of ways meh help karte hai.
1. Isse hum mass of a single atom of an element or a single molecule of a substance ko calculate karne ke liye use karte hai.
2. Isse hum number of atoms or molecules in a given mass of the substance ko calculate karne ke liye use karte hai.
3. Also, isse hum moles from mass, volume or number of particles ko calculate karne ke liye bhi use karte hai.
Mole Concept : In terms of mass, number of particles, volume
We use the idea of the mole to count entities at the microscopic level (i.e., atoms, molecules, particles, electrons, ions, etc).
Mole (symbol, mol) is the seventh base quantity for the amount of a substance.
One mole is the amount of a substance that contains as many particles or entities as there are atoms in exactly 12 g (or 0.012 kg) of the 12C isotope.
Or A mole of a substance contains Avogadro number (6.022×1023) of particles.
We need to understand the basics of Avogadro’s number for the mole concept.
Avogadro's no. : It is defined as the number of atoms present in 1 gram atom of element or number of molecules present in 1 gram molecule of the substance.
Note : One cannot just say one mole of hydrogen it needs to be specified that whether it’s one mole of a hydrogen atom or one mole of a Hydrogen molecule.
We can define molar mass in terms of moles as - The mass of one mole of a substance in grams is called its molar mass.
A mole can be defined in many ways :
(a) In terms of Mass : No. of moles of a substance is equal to the given mass of substance divided by its atomic or molecular mass.
For example : 1 mole of C= Atomic mass of Carbon =12 g of Carbon
1 mole of Sulphur = Atomic mass of Sulphur =32 g of Sulphur
1 mole of CH4= Molecular mass of CH4
= Atomic mass of C+ Atomic mass of Hydrogen ×4
=12 g +4 g =16 g
(b) In terms of Number of Particles : Particles include ions, electrons, atoms, molecules. A mole is defined as that amount of the substance which contains Avogadro's number (6.022×1023) of atom if the substance is atomic or Avogadro's number (6.022×1023) of molecules (the substance is molecular)
For example, 1 mol of hydrogen atoms =6.022×1023 atoms
1 mol of water molecules =6.022×1023 water molecules
(c) In terms of Volume : In case of gases, a mole is defined as that amount of the gas which has a volume of 22.4 litres at STP.
For example : 1 Mole of CO2 gas =22.4 litres of carbon dioxide gas at STP
1 Mole of oxygen gas =22.4 litres of oxygen at STP
Note : STP (Standard Temperature and Pressure) :
When Temperature =0∘C or 273.15 K, Pressure =1 atm, Molar Volume =22.4 L or
When Temperature =0∘C or 273.15 K, Pressure =1 bar, Molar Volume =22.7 L
Importance of Avogadro's Number and Mole Concept : Avogadro's number and mole concept help in the chemical calculation in a number of ways as follows :
1. In the calculation of the mass of single atom of an element or a single molecule of a substance.
2. In the calculation of the number of atoms or molecules in a given mass of the substance.
3. In the calculation of moles from mass, volume or number of particles.
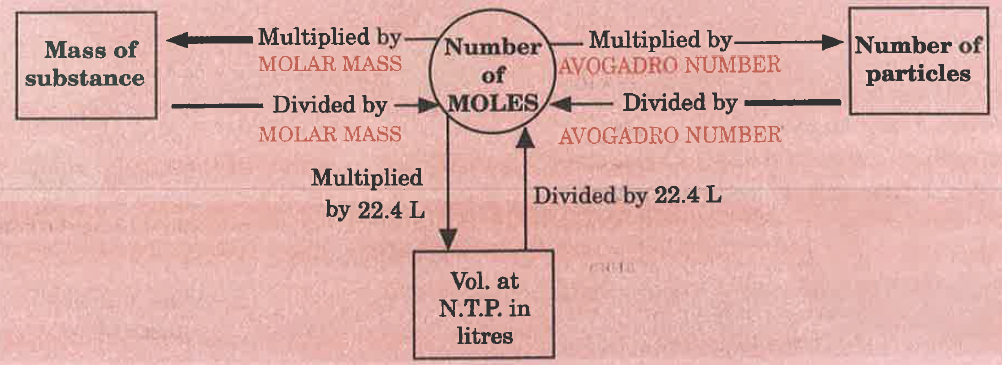
Hello Points!!!
The scheme for calculation of moles, mass, number of particles as summarised below:
EXAMPLE 1
Silver is a very precious metal and is used in Jewellery. One million atoms of silver weigh 1.79×10−16g. Calculate the atomic mass of silver.
Atomic mass of an element is the mass of 6.022×1023 atoms.
Now, 106 atoms of silver weigh =1.79×10−16g
6.022×1023 atoms of silver weigh =1.79×10−16×6.022×1023106=107.8g
Atomic mass of silver =107.8
EXAMPLE 2
An element X has the following isotopic composition :
200X:90%,199X:8.0%,202X:2.0%
The weight average atomic mass of the naturally occurring element X is closest to
Average isotopic mass of X
Average Atomic Mass =∑(Relative abundance of the elements×Molar mass of elements)100
X=200×90+199×8+202×2100
=18000+1592+404100=19996100
=199.96 amu or ≈200 amu
EXAMPLE 3
Given that the abundances of isotopes 54Fe,56Fe, and 57Fe are 5%, 90%, and 5 % respectively. The atomic mass of iron is : [JEE 2009]
Average atomic mass =∑(Relative abundance of the elements×Molar mass of elements)100
=0.05×54+0.9×56+0.05×57
=55.95.
EXAMPLE 4
How many moles of lead (II) chloride will be formed from a reaction between 6.5g of PbO and 3.2g of HCl ? [AIPMT 2008]
According to balanced equation,
PbO+2HCl(aq.)→PbCl2+H2O(l)1 mol2 mol1 mol2 mol
Calculating moles of reactants :
nPbO=6.5g223.2gmol−1=0.029mol
nHCl=3.2g36.5gmol−1=0.087mol
Calculating moles of product formed from reactions:
0.029molPbO×1molPbCl21molPbO=0.029mol PbCl2
0.087molHCl×1molPbCl22molHCl=0.44mol PbCl2
Thus, PbO is the limiting reactant and 0.029mol−PbCl2 will be formed.
EXAMPLE 5
Calculate
(a) Mass of 2.5 gram atoms of magnesium,
(b) Gram atoms in 1.4 grams of nitrogen (Atomic mass : Mg=24,N=14)
(a) 1 gram atom of Mg=24g
2.5 gram atoms of Mg=24×2.5=60 g
(b) 1 gram atom of N=14 g ,
14g of N=1 gram atom
1.4 g of N=114×1.4=0.1 gram atom
EXAMPLE 6
Calculate the mass of
(i) 2.5 gram molecules of H2S (ii) 3.6 gram molecules of glucose (C6H12O6)
Number of Moles = mass of substancemolar mass of substance
Since 1 gram molecule =1 mole
(i) Molar mass of H2S=2×1+32=34 g
2.5 gram molecules of H2S=34×2.5=85 g
(ii) Molecular mass of glucose =12×6+1×12+16×6=180 g
3.6 gram molecules of glucose =180×3.6=648g
EXAMPLE 7
How many moles of electrons weigh one kilogram? [JEE 2018]
1 mol of electrons weight =9.108×10−31×6.022×1023kg
or 1 kg of electrons =19.108×6.022×108 or 0.0182×108 mol
EXAMPLE 8
In three moles of ethane(C2H6), calculate the following:
(i) Number of moles of carbon atoms.
(ii) Number of moles of hydrogen atoms.
(iii) Number of molecules of ethane.
(i) 1 mole of C2H6 contains 2 moles of carbon atoms.
∴ Number of moles of carbon atoms in 3 moles of C2H6=2×3=6
(ii) 1 mole of C2H6 contains 6 moles of hydrogen atoms.
∴ Number of moles of carbon atoms in 3 moles of C2H6=3×6=18
(iii) 1 mole of C2H6 contains 6.023×1023 molecules of ethane.
∴ Number of molecules in 3 moles of C2H6.
=3×6.023×1023=18.069×1023
EXAMPLE 9
Nitrogen atom has atomic number 7 And oxygen has atomic number 8. Calculate the total number of electrons in nitrate ion.
Formula of nitrate ion =NO−3
Number of electron in NO−3= Electrons in N+3× electron in O+1 (due to negative charge) =7+3×8+1=32
EXAMPLE 10
The number of electrons, protons and neutrons in a species are equal to 10,8 and 8 respectively. Assign the proper symbol to the species.
Electrons =10, Protons =8, Neutrons =8
Atomic number = No. of protons =8
Mass number = No. of protons + No. of neutrons =8+8=16
As the species has two electrons more than protons, it has two units of −ve charge. Hence, the species is 168O2−.
Hello Points!!!
.PNG)
Laws of Chemical Combination1) Law of Conservation of Mass (Lavoisier – `1774`)
In any physical or chemical change, mass can neither be created nor be destroyed.
It means :
This relationship holds good when reactants are completely converted into products.
In case the reacting material are not completely consumed the relationship will be :
Limitation : In nuclear reactions, some mass of reactant is converted into energy, so mass of reactant is always less than that of product.
EXAMPLE 11
1.7 gram of silver nitrate dissolved in 100 gram of water is taken 0.585 gram of sodium chloride dissolved in 100 gram of water is added to it and chemical reaction occurs 1.435 gm of AgCl and 0.85 gm NaNO3 are formed. Show that these results illustrate the law of conservation of mass.
Total mass before chemical change = mass of AgNO3+ Mass of NaCl+ Mass of water =1.70+0.585+200=202.285 gram
Total mass after the chemical reaction = mass of AgCl+ Mass of NaNO3+ Mass of water =1.435+0.85+200=202.285 gram
Thus, in the given reaction
Total mass of reactants = Total mass of the products.
2) Law of Constant Composition : [Proust `1799`]
A chemical compound always contains the same element combined together in fixed proportion by mass.
Limitations : In case of isotopes, ratio is not fixed.
e.g.

EXAMPLE 12
1.08 gram of Cu wire was allowed to react with nitric acid. The resulting solution was dried and ignited when 1.35 gram of copper oxide was obtained. In another experiment 2.3 gram of copper oxide was heated in the presence of Hydrogen yielding 1.84 gram of copper. Show that the above data are in accordance with the law of constant composition ?
Case −I
1.35 gram CuO contains →1.08 gram Cu.
100 gram CuO contains →80 gram Cu
%Cu in CuO=80%
%O in CuO=20%
Case −II
2.30 gram CuO contains →1.84 gram Cu.
100 gram CuO contains →80 gram Cu.
%Cu in CuO=80%
%O in CuO=20%
Both samples have the same composition & hence the data are in accordance with the law of constant composition.
EXAMPLE 13
1.375 gram of cupric oxide was reduced by heating in a current of hydrogen and the weight of copper that remained was 1.098 gram. In another experiment, 1.179 gram of Cu was dissolved in the nitric acid and the resulting copper nitrate is converted into cupric oxide by ignition. The weight of cupric oxide formed was 1.476 gram. Show that these results illustrate the law of constant composition.
First case :
Copper oxide taken =1.375g
Copper left =1.098g
∴ Oxygen present =1.357g−1.098=0.277g
Hence, % of O2 in CuO=0.277×1001.357=20.14
Second case :
Copper taken =1.179g
Copper oxide formed =1.476g
∴ Oxygen present =1.476g−1.179=0.297g
Hence, % of O2 in CuO=0.297×1001.476=20.41
Hence, percentage of oxygen is same in both of the above cases, so the law of constant composition is proved.
3) Law of Multiple Proportion: [Dalton `1806`]
When two elements combine to form two or more compounds, the different masses of one element which combine with a fixed mass of the other element, bear a simple ratio to one another.
EXAMPLE 14
Two compounds each containing only tin and oxygen had the following composition.

Show that these data illustrate the law of multiple proportion ?
In compound A
21.23 parts of oxygen combine with 78.77 parts of tin.
1 part of oxygen combined with 78.7721.23=3.7 parts of Sn.
In compound B
11.88 parts of oxygen combine with 88.12 parts of tin.
1 part of oxygen combined with 88.1211.88=7.4 parts of tin.
Thus, the mass of Tin in compound A and B which combine with a fixed mass of oxygen are in the ratio 3.7:7.4 or 1:2. This is a simple ratio. Hence the data illustrate the law of multiple proportion.
EXAMPLE 15
Carbon and oxygen are known to form two compounds. The carbon content in one of these is 42.9% while in the other it is 27.3%. Show that these data are in agreement with the law of multiple proportion.
In a compound of C and O
C=42.9,O=57.1
∴ Rate of C:O::42.957.1=0.751
In other compound of C and O
C=27.3,O=72.7
∴ Ratio of C:O::27.372.7=0.376
Thus, the ratio of amounts of C reacting with same mass of O=0.751:0.376=2:1
This is in agreement with law of multiple proportions.
4) Law of Reciprocal Proportion : [Richter `1794`]
When two different elements combine with the same mass of a third element, the ratio in which they do so will be the same or simple multiple if both directly combined with each other.
EXAMPLE 16
The % composition of NH3,H2O and N2O3 is as given below :
NH3→82.35%N and 17.65%H.
H2O→88.9%O and 11.1%H.
N2O3→63.15%O and 36.85%N.
On the basis of above data prove the law of reciprocal proportion ?
(i) For NH3 1−part of hydrogen reacts with =82.3517.65=4.67 part N.
(ii) For H2O 1−part of hydrogen reacts with =88.9011.10=8.01 part O.
Thus, the ratio N:O=4.67:8.01=1:1.71
(iii) For N2O3:N and O reacts with each other N:O=36.85:63.15=1:1.71
Because the two ratios are the same, thus the law of reciprocal proportion is proved.
5) Gay-Lussac’s Law of Gaseous Volumes [Gay-Lussac – `1808`]
When gases are combined or produced in a chemical reaction, they do so in a simple ratio by volume provided all the gases are at same temperature and pressure.
Empirical & Molecular Formula
Ek compound meh various atoms ki simplest whole number ratio ko Empirical formula represent karta hai whereas a molecule of a compound meh different types of atoms ke exact number ko Molecular formula represent karte hai.
Molecular Formula =n× Empirical Formula
Calculation of Empirical Formula
Step 1) Sabse pehle mass percent ko grams meh convert karne ke liye hum ek compound meh 100 g consider karte hai aur given mass percentage, elements ke masses ko grams meh represent karta hai.
Step 2) Then number of moles ko calculate karne ke liye hum each element ki percentage (mass in g) ko inke atomic mass se divide karte hai. Jo hume as a result various elements ke number of moles deta hai.
Moles of elements = Given mass / molecular mass
Step 3) Ab simplest molar ratio calculate karne ke liye hum moles ko jo step 2 meh mile hai unhe smallest quotient or the least value from the values obtained for each element se divide karte hai. Ye hume then simplest molar ratio deta hai.
Step 4) Ab step 3 meh jo hum simplest molar ratio calculate karte hai wo generally whole numbers hote hai. Lekin agar aisa nahi hota tab hum (a) isse nearest whole number value tak raise karte hai or (b) hum yahan sabhi simplest atomic ratios ko ek suitable integer se multiply kar dete hai.
Step 5) Ab empirical formula likhne ke liye hum various elements ke symbols ko pehle side by side likhte hai. Then step 3 meh jo simplest whole number ratio milti hai usse hum lower right hand corner of each symbol likhte hai.
As a result ye hume Empirical formula deta hai.
Empirical & Molecular Formula
An Empirical formula represents the simplest whole-number ratio of various atoms present in a compound, whereas, the Molecular formula shows the exact number of different types of atoms present in a molecule of a compound.
Molecular Formulae =n× Empirical Formulae
Calculation of Empirical Formula
Step 1) To convert the mass per cent into grams. Considering 100 g of the compound, the given mass percentages represent the masses of the elements in grams.
Step 2) To calculate the number of moles. Divide the percentage (mass in g) of each element by its atomic mass. This gives the number of moles of the various elements
Moles of elements = Given mass / Molecular Mass
Step 3) To calculate the simplest molar ratio. Divide the moles obtained in step 2 by the smallest quotient or the least value from the values obtained for each element. This gives the simplest molar ratio.
Step 4) To calculate the simplest whole-number ratio. The simplest molar ratio as calculated in step 3 is generally whole numbers.
If not, then (a) raise the values to the nearest whole number, or (b) multiply all the simplest atomic ratios by a suitable integer.
Step 5) To write the empirical formula. Write the symbols of the various elements side by side. Now insert the simplest whole-number ratio of each element as obtained in step 3 at the lower right-hand corner of each symbol.
This will give us the Empirical Formulae.
EXAMPLE 17
A compound contains 4.07% hydrogen, 24.27% carbon and 71.65% chlorine. Its molecular mass is 98.96. What are its empirical and molecular formulae ?
nH=4.07g1.008gmol−1=4.04
nC=24.27g12.01gmol−1=2.02
nCl=71.65g31.45gmol−1=2.2
Dividing by the smallest number, we get
4.042.02:2.022.02:2.22.02 =2:1:1
∴EF is CH2Cl and EFM is (12.01+2×1.008+35.45)
=49.48u
n=MFMEFM=98.96u49.48u=2
∴MF=2×EF=2(CH2Cl)=C2H4Cl2
EXAMPLE 18
2.746 g of a compound gave on analysis 1.94 g of silver, 0.268 g of sulphur, 0.538 g of oxygen. Calculate the empirical formula of the compound (At. masses : Ag=108, S=32, O=16).
Calculation of Empirical Formula 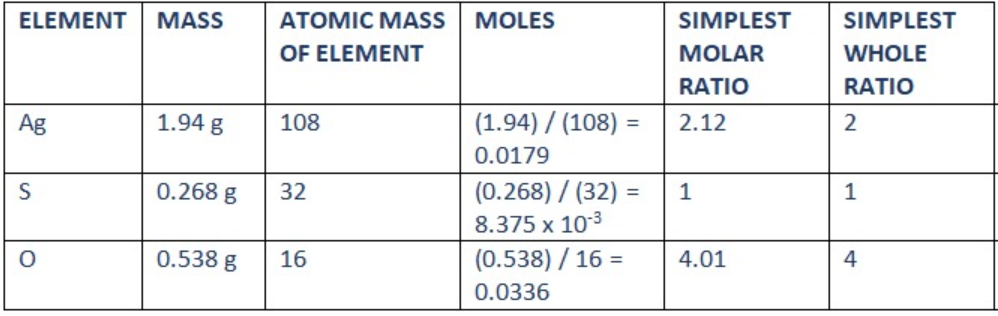
The Empirical Formulae is Ag2SO4
EXAMPLE 19
A gaseous hydrocarbon gives upon combustion, 0.72 g of water and 3.08 g of CO2. The empirical formula of the hydrocarbon is [JEE MAINS 2013]
18g H2O contain 2g H.
∴0.72g H2O contain 0.08gH
44g CO2 contain 12g C.
∴3.08g CO2 contain 0.84g C.
∴C:H=0.8412:0.081=0.07:0.08=7:8
∴ Empirical formula=C7H8
Vapour Density
Some times in numericals molecular mass of volatile substance is not given, instead vapour density is given. Vapour density can be defined as
or V.D.=Mgas2
Mgas=2×V.D.
EXAMPLE 20
A compound of nitrogen and oxygen was found to contain 7:16 by mass N and O respectively. Calculate molecular formula of the compound if V.D. is 46 ?
Let mass of N=7 K gram
Mass of O=16 K gram
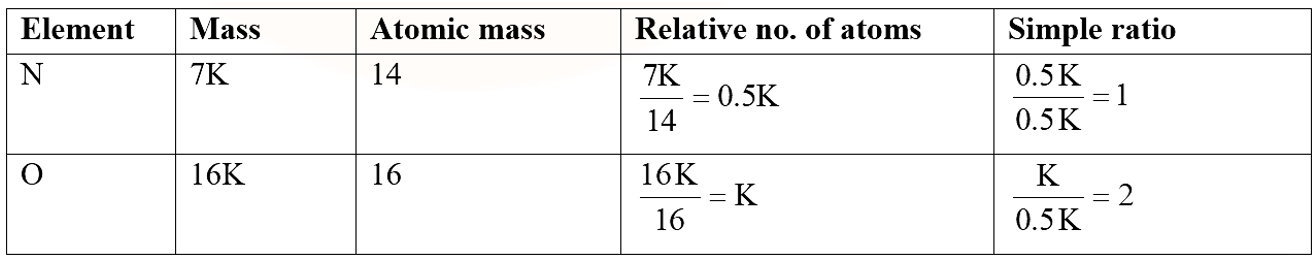
N:O=1:2
Empirical formula =NO2
(Empirical formula)n= molecular formula
n=M. Massempirical formula mass=2×V.D46=2×4646=2
Molecular formula =(NO2)2=N2O4
Stoichiometry & Stoichiometric Calculations
Ab balanced chemical equation ke coefficients ko hum Stoichiometric Coefficients bolte hai. Iske alawa reactant aur product ke relationship ko in terms of moles, masses, volume ko hum Stoichiometry bolte hai.
Ab hum inn terms ko following cases ke through study karenge :
Note : Steps used in calculation
(i) Sabse pehle hum balanced chemical equation likhenge.
(ii) Ab hum reactants aur products ke formulae ke niche inke relative number of moles or relative masses (gram atomic or molecular masses) ko likhte hai.
(iii) In case of a gaseous substance, hum inke formula ke niche 22.4 Litres at STP likhte hai in place of 1 mole.
(iv) Then hum required calculations ke liye unitary method apply karte hai.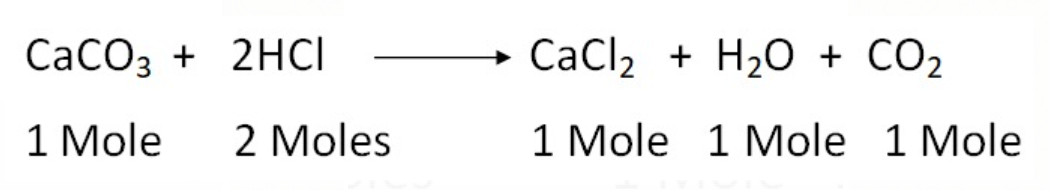
Mole − Mole relationship : Iss case meh moles of one product or reactant given hota hai aur moles of other hume calculate karna hota hai.
Ab given reaction meh, jab 1 mole of calcium carbonate aur 2 moles of Hydrochloric acid react karte hai tab ye hume 1 mole Calcium Chloride, 1 mole of water aur 1 mole of carbon dioxide dete hai.
So, by unitary method 1 mole of HCl, 0.5 mole of CaCO3 ke sath react karta hai.
(a) Mass − Mass relationship : Iss case meh mass of one product or reactant given hota hai aur mass of other hume calculate karna hota hai.
(b) Mass − Volume relationship : Yahan Mass / volume of one of the product or reactant given hota hai aur mass / volume of other hume calculate karna hota hai.
(c) Volume − Volume relationship : Iss case meh bhi volume of one of the product or reactant given hoti hai aur volume of other hume calculate karni hoti hai.
Limiting Reagent
Ab many cases meh mixture ke substances exactly same amount meh present nahi hote jitna balanced chemical equation meh required hota hai. Inn situations meh ek reactant dusre ki respect meh excess amount meh hota hai. Ab jo reactant lesser amount meh hota hai wo after sometime consumed ho jata hai, jiske wajah se tab further reaction nahi hoti hai even though other reactant present hota hai. Thus,
the reactant which gets completely consumed in a reaction and limits the amount of product formed is called a limiting reagent.
1) Hence limiting reagent wo reagent hota hai jo reaction meh pehle hi consumed ho jata hai.
2) Ab jo product formed hota hai usse hum limiting reagent se hi determine karte hai aur isko hum maximum product formed b bolte hai.
3) The other reactant jo rah jata hai wo excess reagent hota hai.
Identification of Limiting Reagent
Step 1: Sabse pehle hum ek balanced chemical reaction likhte hai.
Step 2 : Then hum reactant ke number of moles likhte hai.
Step 3 : Further hum moles of the reactant ko inke respective stoichiometric coefficients se divide karte hai. Ab isme se jo least value hoti hai wo hume limiting reagent deti hai.
For Example :
24 g C reacts with 64 g O2
i.e., 2 mole C reacts with 2 mole O2
Moles of CStoichiometry of C=21=2 Moles of O2Stoichiometry ofO2=20.5=4
Since 2 is lesser than 4
∴ C is Limiting Reagent and will decide the amount of CO formed.
As 1 mole of C gives 1 mole of CO
∴ 2 mole of C gives 2 mole of CO.
Stoichiometry & Stoichiometric Calculations
The coefficients of the balanced chemical equation are called Stoichiometric Coefficients and the relationship between reactant and product in terms of moles, masses, volume is Stoichiometry.
We can understand these terms by the following cases
Note : Steps used in calculation
(i) Write down the balanced chemical equation.
(ii) Write the relative number of moles or the relative masses (gram atomic or molecular masses) of the reactants and the products below their formulae.
(iii) In case of a gaseous substance,write down 22.4 Litres at STP below the formula in place of 1 mole
(iv) Apply unitary method to make the required calculations.
Mole - Mole relationship : Moles of one product or reactant is given and moles of other needs to be calculated
In the given reaction, 1 mole of calcium carbonate reacts with 2 moles of Hydrochloric acid to give 1 mole Calcium Chloride, 1 mole of water and 1 mole of carbon dioxide.
So, 1 mole of HCl will react with 0.5 mole of CaCO3 (unitary method)
(a) Mass - Mass relationship : Mass of one reactant or product is given and mass of other needs to calculated.
(b) Mass - Volume relationship : Mass / volume of one of the product or reactant is given and mass/volume of other is to be found.
(c) Volume - Volume relationship : Volume of the product or reactant is given and volume of other is to be found.
Limiting Reagent
When in a reaction if one of the reactant is not present in amounts as required by a balanced chemical reaction and is in the excess amount over the other the reactant which is present in lesser amount is consumed and after sometime no further reaction takes place whatever be the amount of other reactant present. Hence,
the reactant which is completely consumed, limits the amount of product formed and is therefore called a limiting reagent.
1) Hence limiting reagent is a reagent that is consumed earlier in reaction.
2) The product formed is determined by limiting reagent, it is also known as the maximum product formed.
3) The other reactant left is excess reagent.
Identification of Limiting Reagent
Step 1 : Write a balanced chemical reaction.
Step 2 : Write the moles of reactant.
Step 3 : Divide the moles of reactant with their respective stoichiometric coefficients the least value will give limiting reagent.
For Example :
24 g C reacts with 64 g O2
i.e., 2 mole C reacts with 2 mole O2
Moles of CStoichiometry of C=21=2 Moles of O2Stoichiometry ofO2=20.5=4
Since 2 is lesser than 4
∴ C is Limiting Reagent and will decide the amount of CO formed.
As 1 mole of C gives 1 mole of CO
∴ 2 mole of C gives 2 mole of CO.
EXAMPLE 21
How many moles of iron can be made from Fe2O3 by the use of 16 mol of carbon monoxide in the following reaction :
Fe2O3+3CO→2Fe+3CO2
The balanced equation is :
3 mol of CO are used to make =2 mol Fe
16 mol of CO are used to make =23×16=10.67 mol
EXAMPLE 22
Oxygen is prepared by the catalytic decomposition of potassium chlorate (KClO3). Decomposition of potassium chlorate gives potassium chloride (KCl) and oxygen (O2). If 2.4 mol of oxygen is needed for an experiment, how many grams of potassium chlorate must be decomposed ?
The balanced equation is
3 mol of O2 is produced by decomposition of KClO3=245 g
2.4 mol of O2 will produce by the decomposition of KClO3=245×2.43=196.0g
Percentage Yield
In general, when a reaction is carried out in the laboratory we do not obtain the theoretical amount of product. The amount of product that is actually obtained is called the actual yield. Knowing the actual yield and theoretical yield, the % yield can be calculated by the following formula.
EXAMPLE 23
For the reaction
1.12 gram of CaO is reacted with excess of hydrochloric acid and 1.85 gm CaCl2 is formed. What is the % yield of the reaction ?
56 gm CaO will produce 111 gm CaCl2
1.12 gram of CaO will produce →11156×1.12=2.22 gm
Thus, Theoretical yield =2.22 gm
Actual yield =1.85 gm
% yield =1.852.22×100=83.33%
Percentage Purity
Depending upon the mass of the product, the equivalent amount of reactant present can be determined with the help of a given chemical equation. Knowing the actual amount of the reactant taken and the amount calculated with the help of a chemical equation, the purity can be determined, as
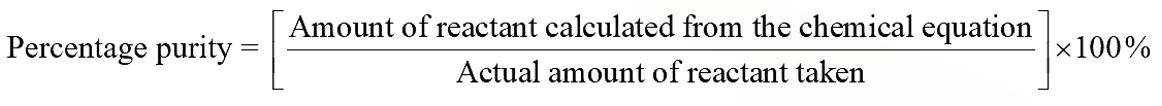
EXAMPLE 24
Calculate the amount of (CaO) in kg that can be produced by heating 200 kg limestone that is 90% pure CaCO3.
Mass of Pure CaCO3=200×90100=180 kg
100180=56x⇒x=100.8 kg
Principle of Atom Conservation (POAC)
The principle of conservation of mass, expressed in the concepts of atomic theory, means the conservation of atoms. And if atoms are conserved, moles of atoms shall also be conserved. This is known as the principle of atom conservation. This principle is in fact the basis of the mole concept.
In order to solve problems of nearly all stoichiometric calculations, let us first see how this principle works. Choose an example,
Apply the principle of atom conservation (POAC) for K atoms.
Moles of K atoms in reactant = mole of K atoms in products
Moles of K atoms in KClO3= moles of K atoms in KCl.
Now, since 1 molecule of KClO3 contains 1 atom of K
or 1 mole of KClO3 contains 1 mole of K, similarly, 1 mole of KCl contains 1 mole of K
Thus, mole of K atoms in KClO3=1× moles of KClO3
and Mole of K atoms in KCl=1× moles of KCl
∴ mole of KClO3= mole of KCl
or =wt. of KClO3 in gmol wt. of KClO3=wt. of KCl in gmol wt. of KCl
The above equation gives the weight relationship between KClO3 and KCl which is important in stoichiometric calculations.
Again, applying the principle of atom conservation for O atoms,
Moles of O in KClO3= moles of O in O2
But since 1 mole of KClO3 contains 3 moles of O and 1 mole of O2 contains 2 moles of O,
Thus, mole of O in KClO3 = 3× moles of KClO3
Moles of O in O2=2× moles of O2
∴ 3× moles of KClO3=2× moles of O2
3×wt. of KClO3mol wt. of KClO3=2×wt. of O2mol wt. of O2
Mole of O2 may also be expressed in volume.
EXAMPLE 25
All carbon atoms present in KH3(C2O4)2.2H2O weighing 254 gm is converted to CO2 . How many gram of CO2 were obtained ?
Apply P.O.A.C. on carbon atom
4× mole of KH3(C2O4)2.2H2O=1× mole of CO2
∴ Mass of CO2=4×44=176 gram
Concentration Terms
A solution is a homogeneous mixture of two or more substances, the composition of which may vary within limits. “A solution is a special kind of mixture in which substances are intermixed so intimately that they cannot be observed as separate components”. The substance which is to be dissolved is called solute while the medium in which the solute is dissolved to get a homogeneous mixture is called the solvent. A solution is termed as binary and ternary if it consists of two and three components respectively.
Methods of Expressing Concentration of Solution
Concentration of solution is the amount of solute dissolved in a known amount of the solvent or solution. The concentration of solution can be expressed in various ways as discussed below :
1) Percentage : It refers to the amount of the solute per 100 parts of the solution. It can also be called as parts per hundred (pph). It can be expressed by any of following four methods :
(i) Weight by weight percentage (%w/w) =Wt. of Solute (g)Wt. of Solution (g)×100
e.g., 10% Na2CO3 solution (w/w) means 10 g of Na2CO3 is dissolved in 100 g of the solution (It means 10 g Na2CO3 is dissolved in 90 g of solvent).
(ii) Weight by volume percent (%w/v) =Wt. of Solute (g)Volume of Solution(cm3)×100
e.g., 10% Na2CO3 (w/v) means 10 g Na2CO3 is dissolved in 100 cm3 of solution.
(iii) Volume by volume percent (%v/v) =Volume of Solute(cm3)Volume of Solution(cm3)×100
e.g., 10% ethanol (v/v) means 10 cm3 of ethanol dissolved in 100 cm3 of solution.
(iv) Volume by weight percent (%v/w) =Volume of SoluteWt. of Solution×100
e.g., 10% ethanol (v/w) means 10 cm3 of ethanol dissolved in 100 g of solution.
2) Parts per million (ppm) and parts per billion (ppb) : When a solute is present in very small quantity, it is convenient to express the concentration in parts per million and parts per billion. It is the number of parts of solute per million (106) or per billion (109) parts of solution. It is independent of the temperature.
ppb =Mass of solute componentTotal Mass of solution×109
3) Normality (N) : It is defined as the number of gram equivalents of a solute present per litre of the solution. Unit of normality is gram equivalents litre−1. Normality changes with temperature since it involves volume. When a solution is diluted x times, its normality also decreases by x times. Concentration in terms of normality is generally expressed as,
N= Normal solution; 5N= Penta normal, 10N= Deca normal;
N/2= semi normal; N/10= Deci normal; N/5= Penti normal
N/100 or 0.01N= centinormal, N/1000 or 0.001= millinormal.
Mathematically normality can be calculated by following formulas,
(i) Normality
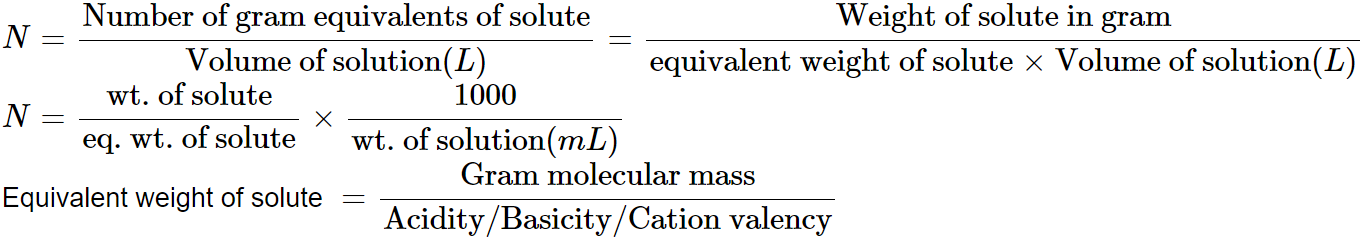
Acidity = No. of replaceable OH− in a molecule of a base
For example : Acidity of NaOH=1
Basicity = No. of replaceable H+ in a molecule of an acid
For example : Basicity of H2SO4=2
(ii) If volume V1 and normality N1 is so changed that new normality is N2 and volume is V2 then,
(iii) When two solutions of the same solute and solvent are mixed then normality of mixture (N) is
4) Molarity (M) : Molarity of a solution is the number of moles of the solute per litre of solution (or number of millimoles per mL of solution). Unit of molarity is mol/litre or mol/dm3. For example, a molar (1M) solution of sugar means a solution containing 1 mole of sugar per litre of the solution. Solutions in terms of molarity is generally expressed as,
1M= One molar solution, 2M= Molarity is two, or 0.5M= Semimolar solution,
M10 or 0.1M= Decimolar solution, M100 or 0.01M= Centimolar solution
M1000 or 0.001M= Millimolar solution
Mathematically, molarity can be calculated by following formulas :
(i)
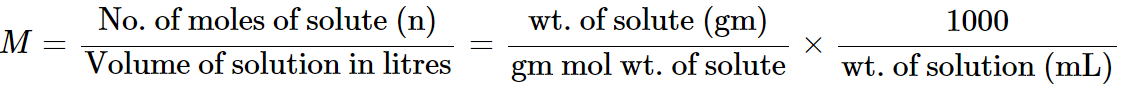
(ii) If molarity and volume of the solution are changed from M1,V1 to M2,V2. Then,
(iii) In balanced chemical equation, if n1 moles of reactant−1 react with n2 moles of reactant−2. Then,
M1V1n1=M2V2n2
(iv) If two solutions of the same solute are mixed then molarity (M) of resulting solution.
5) Formality (F) : Formality of solution may be defined as the number of gram formula units of the ionic solute dissolved per litre of the solution. It is represented by F. Commonly, the term formality is used to express the concentration of the ionic solids which do not exist as molecules but exist as network of ions. A solution containing one gram formula mass of solute per litre of the solution has formality equal to one and is called Formal solution. It may be mentioned here that the formality of a solution changes with change in temperature.
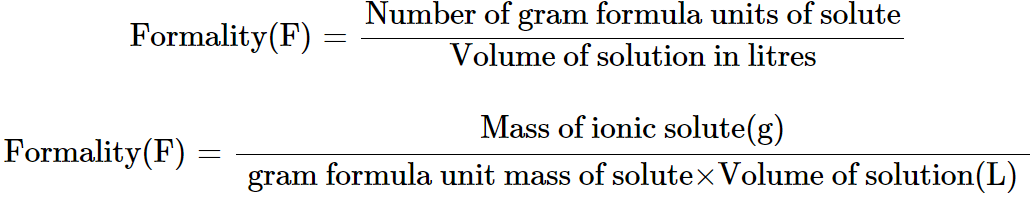
6) Molality (m) : It is the number of moles of the solute per 1000 g of the solvent. Unit of molality is mol/kg. For example, a 0.2 molal (0.2 m) solution of glucose means a solution obtained by dissolving 0.2 mole of glucose in 1000 gm of water. Molality (m) does not depend on temperature since it involves measurement of weight of liquids.
Mathematically molality can be calculated by following formulas,
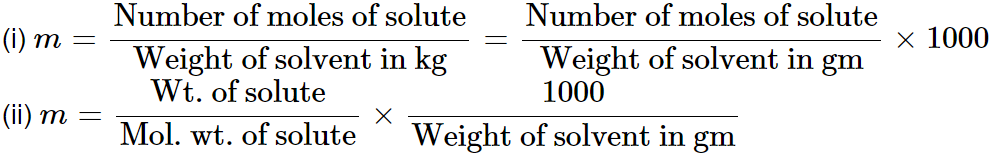
7) Mole fraction (χ) : Mole fraction may be defined as the ratio of the number of moles of one component to the total number of moles of all the components (solvent and solute) present in the solution. It is denoted by the letter χ. It may be noted that the mole fraction is independent of the temperature. Mole fraction is dimensionless. If a solution contains the components A and B and suppose that WA gram of A and WB gram of B are present in it.
Number of moles of A is given by, nA=WAMA and the number of moles of B is given by, nB=WBMB
where MA and MB are molecular mass of A and B respectively.
Total number of moles of A and B=nA+nB
Mole fraction of A, χA=nAnA+nB
Mole fraction of B, χB=nBnA+nB
The sum of mole fractions of all the components in the solution is always one.
χA+χB=nAnA+nB+nBnA+nB=1
χA+χB=1
Thus, if we know the mole fraction of one component of a binary solution, the mole fraction of the other can be calculated.
8) Mass Fraction : Mass fraction of a component in a solution is the mass of the component divided by the total mass of the solution. For a solution containing wA gm of A and wB gm of B.
Mass fraction of A=wAwA+wB
Mass fraction of B=wBwA+wB
Note : It may be noted that molality, mole fraction, mass fraction etc. are preferred to molarity, normality, formality etc. Because the former involve the weights of the solute and solvent whereas later involve volumes of solutions. Temperature has no effect on weights but it has significant effect on volumes.
EXAMPLE 26
Concentrated nitric acid used as laboratory reagent is usually 69% by mass of nitric acid. Calculate the volume of the solution which contains 23 g nitric acid. The density of concentrated acid is 1.41 g cm−3.
Given HNO3 is 69% by mass;
Density of HNO3=1.41 g cm−3
Thus (i) 69 g HNO3 is present in conc. HNO3=100 g
23 g HNO3 is present in conc. HNO3=10069×23=33.33 g
(ii) Volume of solution required =MassDensity=33.331.41=23.64 mL
EXAMPLE 27
Calculate the parts per million of SO2 gas in 250 mL water (density 1 g cm−3) containing 5×10–4 g of SO2 gas.
Mass of SO2 gas =5×10–4 g;
Mass of H2O= Volume × Density =250 cm3×1 g cm−3=250 g
∴ Parts per million of SO2 gas =5×10−4250g×106=2
EXAMPLE 28
EXAMPLE 29
A bottle of commercial sulphuric acid (density 1.787 g mL−1) is labelled as 86% by weight. What is the molarity of acid ?
(i) Molarity of H2SO4=Wt. of H2SO4 in 1L solutionmol. wt. of H2SO4
But wt. of given H2SO4 per litre =86100×1.787×1000=1536.82 g
Hence molarity of H2SO4=1536.8298=15.68 mol L−1
EXAMPLE 30
What will be the formality of KNO3 solution having strength equal to 2.02 g per litre ?
Strength of KNO3=2.02 g L−1
And g formula weight of KNO3=101 g
∴ Formality of KNO3=0.02 F
EXAMPLE 31
The density of a 3 M Na2S2O3 solution is 1.25 g mL−1. Calculate percentage by mass of Na2S2O3 and molalities of Na+ and S2O2−3 ions.
(i) Total mass of Na2S2O3 solution = Vol of solution × Density of solution
Total mass of Na2S2O3 solution =1000 mL ×1.25 g mL−1=1250 g
Wt. of 3 M Na2S2O3 in 1000 mL solution =3× Mol. wt of Na2S2O3 (158)=474 g
[Mol. wt. of Na2S2O3=158 g mol−1]
∴ % of Na2S2O3=Wt. of Na2S2O3Total wt. of solution×100=4741250×100=37.92%
(ii) To find molality of Na+ ions
3 M Na2S2O3 contain 6 moles of Na+ ions in one litre
∴ Molality of Na+ ions =6×1000wt. of solvent(1250−474=776g)=6×1000776=7.73 m
(iii) To find molality of S2O2–3
3 M Na2S2O3 contain 3 moles of S2O2–3 ions in one litre
Molality of S2O2–3=3×1000wt. of solvent=776g=3.865 m
EXAMPLE 32
Find out the masses of acid and water required to prepare 1 mole of CH3COOH solution of 0.3 mole fraction of CH3COOH.
χCH3COOH=0.3
χH2O=1−0.3=0.7
Wt. of CH3COOH=χCH3COOH× mol. wt. (CH3COOH)=0.3×60=18 g
Wt. of water =χH2O× mol. wt. (H2O)=0.7×18=12.6 g
EXAMPLE 34
Calculate the percentage composition of various elements in :
Sodium carbonate, Na2CO3
Given that the relative atomic masses of O=16, Na=23 and C=12.
Relative molecular mass of Na2CO3
=23×2+12+16×3
=46+12+48=106 amu
Since 106 g of Na2CO3 contains 46 g of sodium, 12 g of carbon, 48 g of oxygen
Thus, % of Na=No. of parts by mass of NaMolar mass of Na2CO3
=46106×100%
=43.4%
% of C=No. of parts by mass of CMolar mass of Na2CO3
=12106×100%
=11.32%
% of O=No. of parts by mass of OMolar mass of Na2CO3
=48106×100%
=45.28%
EXAMPLE 35
Calculate the mass per cent of calcium, phosphorus and oxygen in calcium phosphate Ca3(PO4)2.
Molecular mass of Ca3(PO4)2
=3×40+2(31+4×16)=310
Mass of Ca=3×40=120
Mass percentage of Ca=120310×100=38.71%
Mass of P=2×31=62
Mass percentage of P=62310×100=20%
Mass of O=8×16=128
Mass percentage of O=128310×100=41.29%
EXAMPLE 36
Mixing or Dilution of Solutions
20 mL N/2 HCl, 60 mL N/10 H2SO4 and 150 mL N/5 HNO3 are mixed together. Calculate the normality of the [H+] in final solution.
N1V1+N2V2+N3V3=NV
(12×20)+(110×60)+(15×150)=N(230)
10+6+30=N(230)
46=N(230)
N=0.2
Mixing of Acid & Base Solutions
In case of mixing of acid and base, calculate equivalent or milliequivalent of acid and base separately, subtract the lower value from higher value, which gives the number of equivalent in the final solution.
EXAMPLE 37
50 mL N/2 HCl, 50 mL N/5 H2SO4 and 200 ml N/10 NaOH are mixed. What will be normality of [H−] ions ?
meq of acids =(50×12)+(50×15)=25+10=35
meq of base =(200×110)=20
After mixing meq of acid =35−20=15
∴ Normality of [H−] ion, N=15300=0.05 Ans.
Some Typical Concentration TermsStrength of `H_2O_2` Solution :
The strength of H2O2 is aqueous solution is expressed in the following two ways :
(i) Percentage strength : The mass of H2O2 present in 100 mL of the aqueous solution is termed as percentage strength. For example, a 25% solution (w/v) of H2O2 means that 25 grams of H2O2 are present in 100 mL of the solution.
(ii) Volume strength : Strength of the sample of H2O2 is generally expressed in terms of the volume of oxygen at 0∘C and 1 atm that one volume of the sample of hydrogen peroxide gives on heating. The commercial samples are marked as '10 volume', '15 volume' or '20 volume'. 10 volume means that one volume of the sample of hydrogen peroxide gives 10 volumes of oxygen at 0∘C and 1 atm. 1 mL of a 10 volume solution of H2O2 will liberate 10 mL of oxygen at 0∘C and 1 atm.
EXAMPLE 38
Find the volume strength of 1.6 M H2O2 solution.
Strength of the solution = Molarity × mol. mass =1.6×34=54.4 g L−1
∴ Volume strength =17.92 V
Relationship between Different Concentration Terms
1) N=M×n factor
2) M=md1+mM2/1000
3) m=1000×x2x1M1
4) m=1000dx2x1M1+x2M2
5) d=M(1m+M21000)
6) Volume strength of H2O2=5.6×N=5.6×Percentile strengthEq. wt. of H2O2(17)×10
7) Volume strength of H2O2=11.2×M=11.2×Percentile strengthMol. wt. of H2O2(34)×10
8) In oleum labelled as (100+x)%
% of free SO3=(80×x18)(w/w)
where N= Normality
M= Molarity
m= molality
d= density of solution
M2= Molecular mass of solute
x2= Mole fraction of solute
x1= Mole fraction of solvent
M1= Molecular mass of solvent
d= Density of solution.
SUMMARY
1) Law of conservation of mass (Lavoisier in 1774) : During any physical or chemical change, the total mass of the products formed is equal to the total mass of reactants consumed.
2) Law of constant composition (Proust in 1798) : A chemical compound always contains same elements combined together in same proportion by maps.
3) Law of multiple proportions (John Dalton in 1803) : When two elements combine together to form two or more than two compounds then the masses of one of the elements that combine with fixed mass of the other bear a simple whole number ratio to one another.
4) Law of reciprocal proportions (Richter in 1792) : When two different elements combine separately with a fixed mass of a third element, then the ratio of their masses in which they do so is either the same or some whole number multiple of the ratio in which they combine with each other.
5) Gay Lussac's law (Gay Lussac in 1808) : When gases react with each other they do so in volumes which bear a simple whole number ratio to one another and to the volume of the products, if they are also gases, provided all volumes are measured under similar condition of temperature and pressure.
6) Avogadro's law : Equal volume of all gases under similar conditions of temperature and pressure contain equal number of molecules.
7) The smallest particle of an element that takes part in chemical reactions is atom.
8) The smallest particle of a substance that has independent existence is molecule.
9) One twelveth (1/12) of the mass of an atom of carbon (C−12) is atomic mass unit. It is equal to 1.66×10−27 kg.
10) Atomic mass is the average relative mass of an atom of element as compared with mass of a carbon atom (C−12) taken as 12 .a.m.u.
11) Molecular mass is the average relative mass of a molecule of the substance as compared with mass of a carbon atom (C−12) taken as 12 a.m.u.
12) Atomic mass expressed in grams is gram atomic mass and molecular mass expressed in grams is gram molecular mass.
13) One mole is 6.022×1023 specified particles.
13) Volume occupied by one mole of a gaseous substance is called gram molecular volume (G.M.V.). Its value is 22.4 L at N. T.P.
14) Mass percentage composition of a compound gives the mass of each element expressed as percentage of the total mass.
15) Empirical formula is the formula which gives the simplest whole number ratio of atoms of different elements present in the molecule of a compound.
16) Molecular formula is the formula which gives the actual number of atoms present in the molecule of the compound. It is whole number multiple of empirical formula.
17) Molarity is the number of moles of solute dissolved per litre of solution. It is expressed as mol dm−3 or M. Molarity changes with temperature because volume of the solution changes with temperature.
18) Molality : It is defined as the no. of moles of solute present in 1 kg of solvent. It is represented by m and it is expressed as mol/kg.
19) Limiting reactant is the reactant which is completely consumed in the reaction.