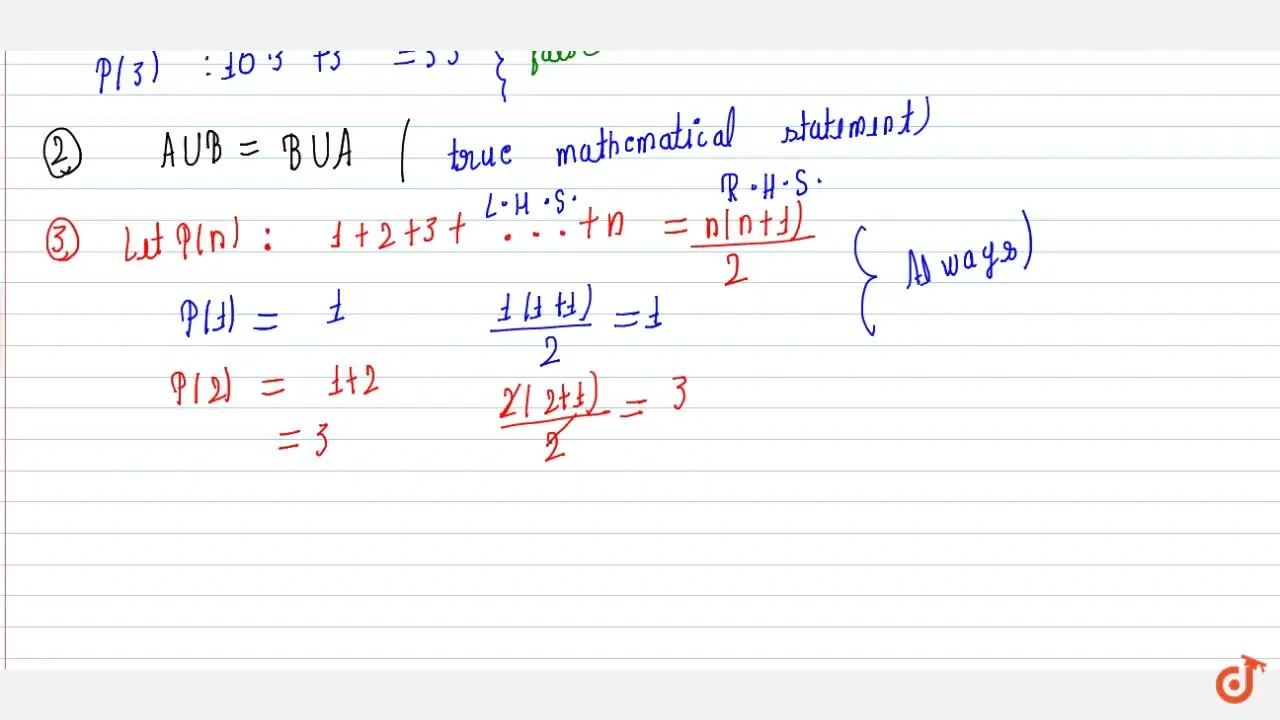
What is Mathematical statements? Explain with the example.
Step by step video & image solution for What is Mathematical statements? Explain with the example. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
What are the technical amendments ?
Explain with the examples.View SolutionWhat are isotones? Give examples.
View SolutionWhat is sublimation? Give three examples of substances which sublime on heating.
View SolutionWhat is smelting? Explain with an example.
View SolutionWhat is smelting? Explain with an example.
View SolutionWhat is Tyndall effect? Explain with an example.
View SolutionWhat is a chemical change? Explain with an example.
View SolutionWhat is atmospheric refraction? Explain with an example.
View SolutionWhat is a tropic movement? Explain with an example.
View SolutionWhat is a biopatent? Give any two examples.
OR
What is biopatent? Explain it with a suitable example.View Solution