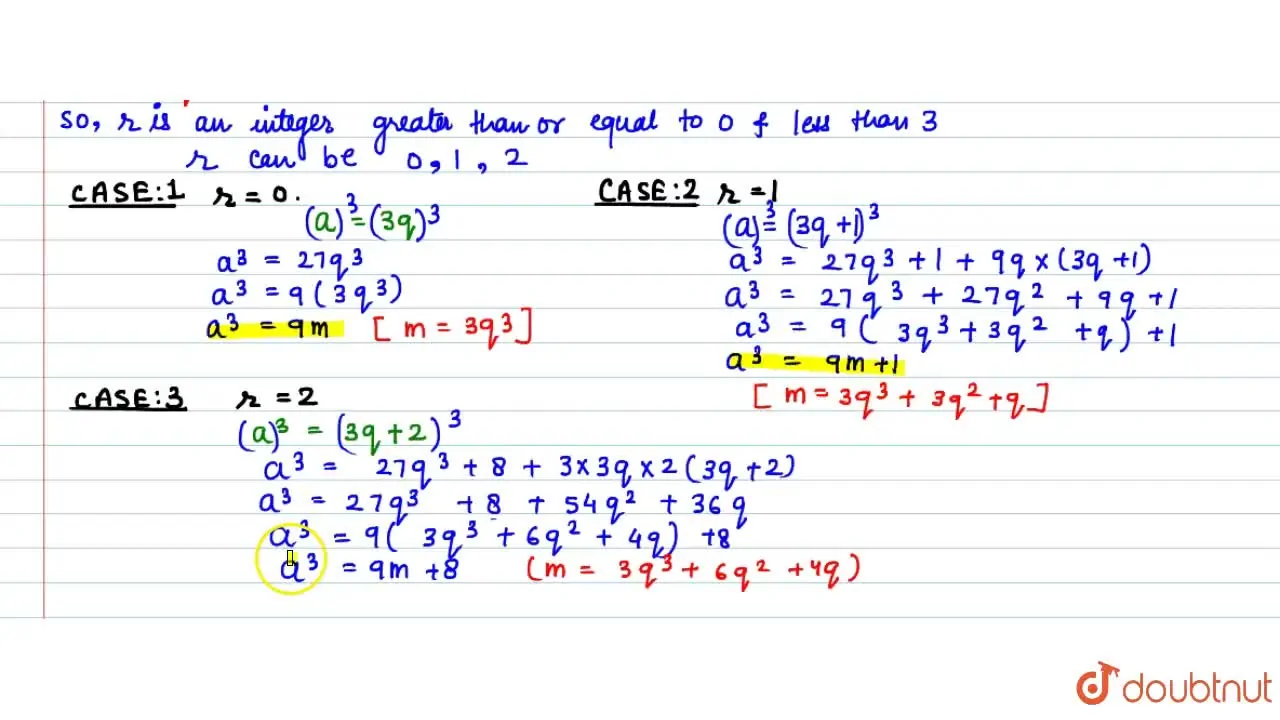
Use Euclid's division lemma to show that the cube of any positive integer is of the form 9m, 9m+1or 9m+8.
a is an integer & b=3
a=3p+r where r=(0,1,2)
case1 : a=3p+0
a3=(3p)3
=27p3=9(3p3)+0;m=3p3
a3=9m
case 2 : a=3p+1
a3=(3p+1)3
=27p3+1+27p2+9p
=9(3p3+3p2+p)+1
=9m+1 where (m=3p3+3p2+p)
case 3 : a=3p+2
a3=(3p+2)2
=27p3+8+27p2+9p
=9(3p3+3p2+p)+8
=9m+8 wherem=3p3+3p2+p
hence proved
Related Playlists
- BOOK - NCERTCHAPTER - REAL NUMBERSEXERCISE - EXERCISE 1.33videos
REAL NUMBERS
- BOOK - NCERTCHAPTER - REAL NUMBERSEXERCISE - Exercise 1.43videos
REAL NUMBERS
- BOOK - NCERTCHAPTER - QUADRATIC EQUATIONSEXERCISE - EXERCISE 4.26videos
QUADRATIC EQUATIONS
- BOOK - NCERTCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - SOLVED EXAMPLES7videos
SOME APPLICATIONS OF TRIGONOMETRY
Similar Questions
Use Euclids division Lemma to show that the cube of any positive integer is either of the form 9m, 9m+1 or, 9m+8 for some integer m .
View SolutionUsing Eulids division lemma, show that the cube of any positive integer is of the form 9m , or ( 9m+1) or ( 9m+8) for some integer m.
View SolutionUse Euclid's division lemma to show that the square of any positive integer is either of the form 3m or 3m + 1 for some integer m.
View SolutionUse Euclid's division lemma to show that the square of any positive integer is either of the form 3mor 3m+1for some integer m.[Hint: Let x be any positive integer then it is of the form 3q, 3q+1or 3q+2Now square each of these and show that they can be rewritten in the form 3m or 3m+1].
View SolutionUsing Eulid's divison lemma, show that the square of any positive interger is either of the form 3m or ( 3m+1) for some integer m. |
View SolutionShow that the square of any positive integer is of the form 3m or, 3m+1 for some integer m .
View SolutionShow that cube of any positive integer is of the form 4m, 4m+1 or 4m+3, for some integer m.
View SolutionProof that the square of any positive integer is of the form of 4m or 4m+1 for some integer m.
View SolutionShow that the square of any positive integer is either of the form 4m or 4m+1 for some integer m.
View SolutionShow that square of any positive integer is of the form of 5m,5m+1,5m−1 for some integer m.
View Solution