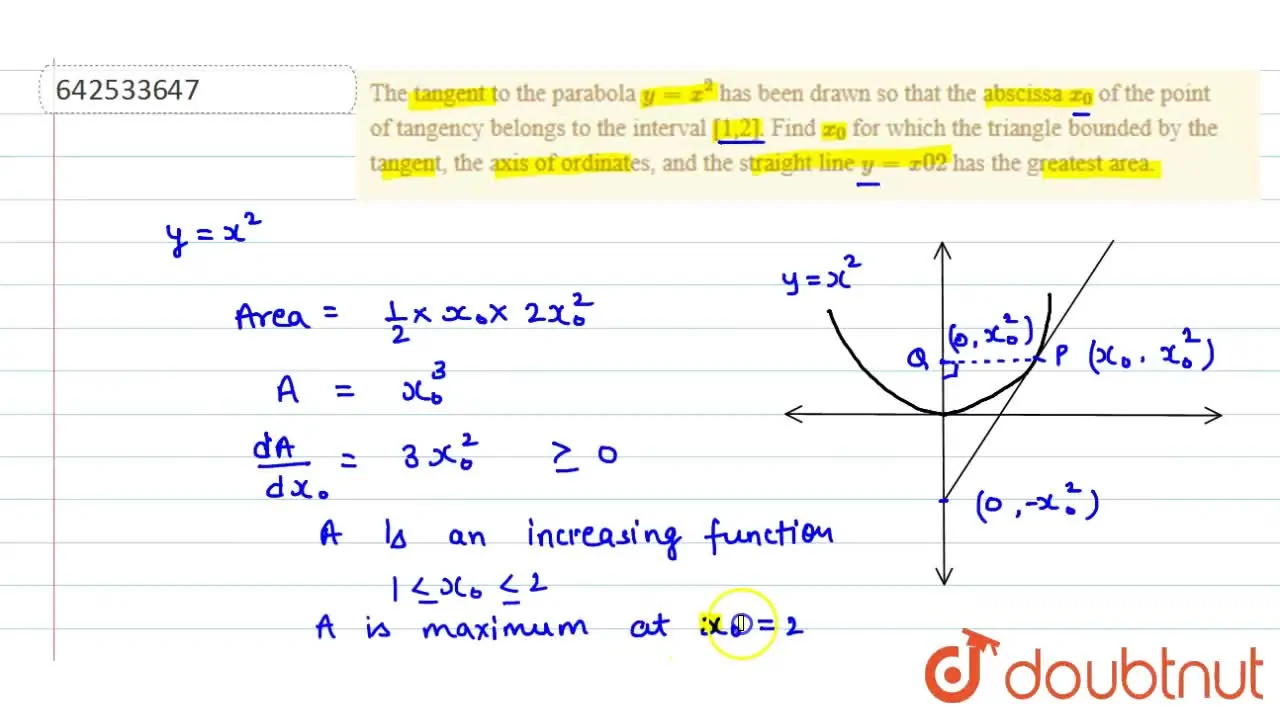
The tangent to the parabola y=x2 has been drawn so that the abscissa x0 of the point of tangency belongs to the interval [1,2]. Find x0 for which the triangle bounded by the tangent, the axis of ordinates, and the straight line y=x02 has the greatest area.
Step by step video & image solution for The tangent to the parabola y=x^2 has been drawn so that the abscissa x_0 of the point of tangency belongs to the interval [1,2]. Find x_0 for which the triangle bounded by the tangent, the axis of ordinates, and the straight line y=x0 2 has the greatest area. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Find the area bounded by the line y=x, the x-axis and the ordinates x=−1 and x=2
View SolutionFind the area bounded by the line y=x, the x-axis and the ordinates x=−1 and x=2
View SolutionA point chanrge of magnitude q=4.5nC is moving with speed v=3.6×107ms−1 parallel to the x-axis along the line y=3m. Find the magnetic field at the origin produced by this charge when the charge is at the point x=−4m, y=3m, as shown in Fig.
 View Solution
View SolutionFind the area bounded by the parabola y=2−x2 and the straight line y+x=0.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area of the triangle formed by the straight lines y=2x,x=0 and y=2 by integration.
View SolutionFind the area of the region bounded by the line y = 2x, X - axis and ordinate x = 2.
View SolutionFind the area of the region bounded by the parabola y2=4ax, its axis and two ordinates x=a and x=2a.
View Solution