The sum of the perimeter of a circle and square is k, where k is some constant. Prove that the sum of their areas is least when the side of square is double the radius of the circle.
Let x be the radius of the circle and y be the side of the square
Given: Sum of the perimeter of the circle and the square is k.
∴2π(radius)+4(side)=k
2πx+4y=k
y=k−2πx4
Now we want to minimize the sum of areas
Let the sum of the areas of the square and circle be A
A=πx2+y2
A=πx2+(k−2πx4)2
Differentiating wrt x,
dAdx=π(2x)+1162(k−2πx)(−2π)
=2πx−π(k−2πx)4
=2πx(4+π)−πk4
Putting dAdx=0
2πx(4+π)−πk4=0
2πx(4+π)=πk
x=k8+2π
d2Adx2=2π(4+π)4>0
Since A''>0, thus A is minimum at x=k8+2π
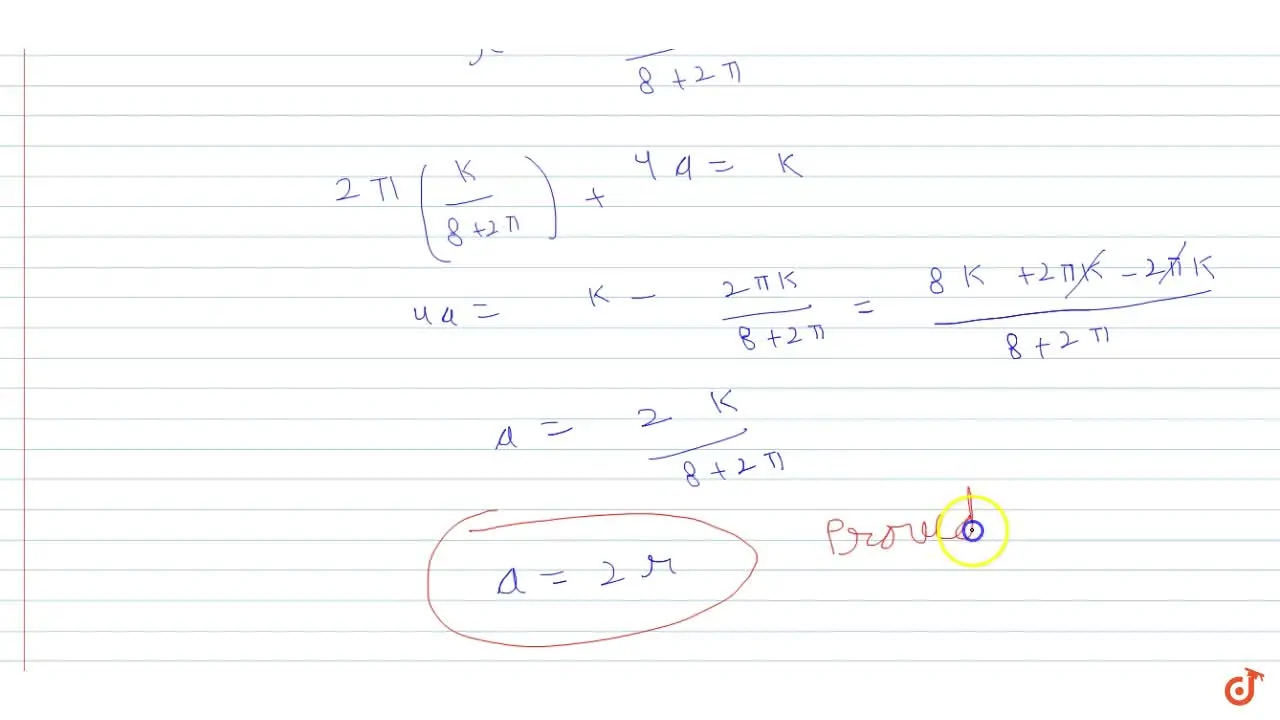
We need to prove that sum of the areas is least when the side of the square is double the radius of the circle.
We know that y=k−2πx4andx=k8+2π
∴y=k−2π(k8+2π)4
y=k4(1−π4+π)
y=k4(4+π−π4+π)
y=k4+π=2(k8+2π)
Hence, the sum of the areas is least when the side of the square is double the radius of the circle.
Related Playlists
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.49videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.529videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.327videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
Similar Questions
The sum of the perimeters of a circle and a square is k , where k is some constant. Prove that the sum of their areas is least when the side of the square is double the radius of the circle.
View SolutionThe sum of perimeter of a square and circumference of a circle is given. Prove that the sum of their areas will be minimum when the side of square is equal to the diameter of the circle.
View SolutionGiven the sum of the perimeters of a square and a circle, show that the sum of their areas is least when one side of the square is equal to diameter of the circle.
View SolutionA wire of length l is cut into two parts. One part is bent into a circle and the other into a square. Prove that the sum of the areas of the circle and the square is the least, if the radius of the circle is half of the side of the square.
View SolutionThe area of a square is equal to the area of a circle. What is the ratio between the side of the square and the radius of the circle?
View SolutionThe areas of a circle and a square are same. The ratio of the side of the square to the radius of the circle is
View SolutionA circle and a square have same area. The ratio of the side of the square to the radius of the circle will be :
View SolutionA circle and a square have same area. The ratio of the side of the square to the radius of the circle will be :
View SolutionThe areas of a circle and a square are same. The ratio of the side of the square to the radius of the circle is
View SolutionIf the perimeter of a circle and a square are equal, then what is the ratio of the area of the circle to that of the square ?
View Solution