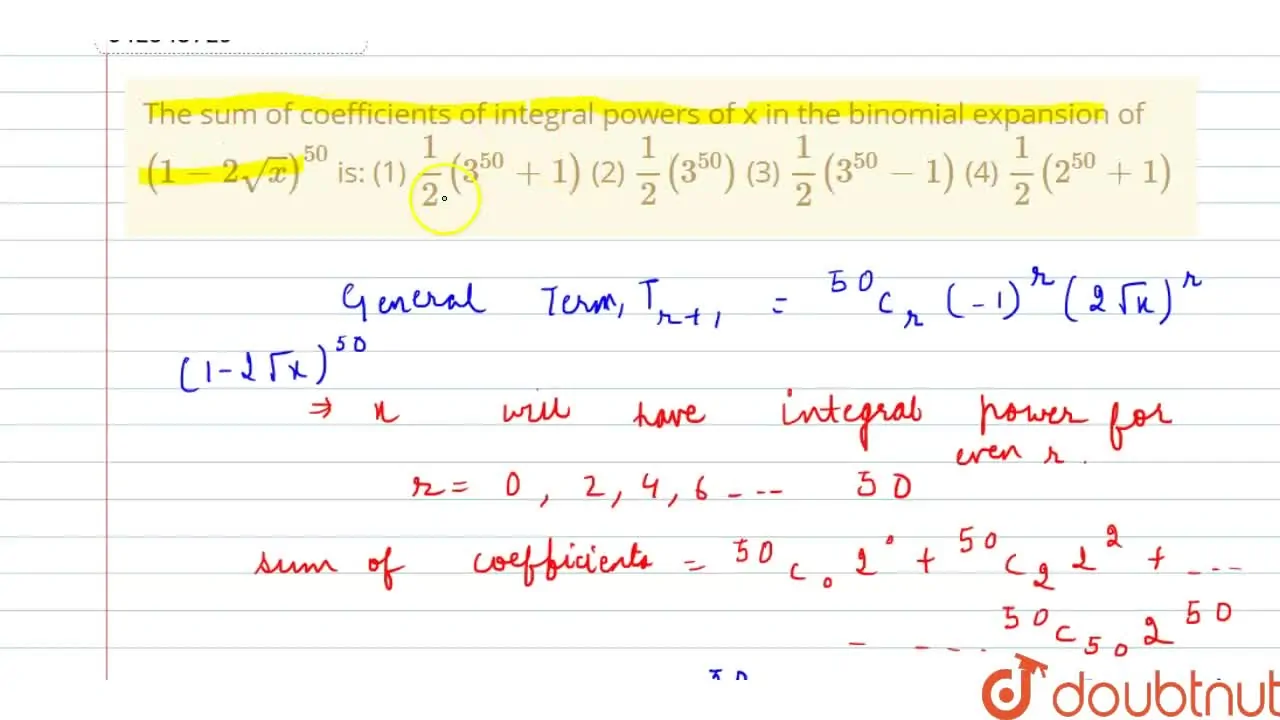
The sum of coefficients of integral powers of x in the binomial expansion of (1−2√x)50 is: (1) 12(350+1) (2) 12(350) (3) 12(350−1) (4) 12(250+1)
12(350+1)
12(350)
12(350−1)
12(210+1)
The correct Answer is:A
(1−2√x)50=C0−C12√x+C2(2√x)2−....+C50+(2√x)50
(1+2√x)50=C0+C1(2√x)+C2(2√x)2+......+C50(2√x)50
Putting x=1, we get
∴350+12=C0+C2(2)2+....
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Multiple Correct Answer4videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Numerical25videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Comprehension Type2videos
AREA
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE7videos
CIRCLE
Similar Questions
Cofficient of x12 in the expansion of (1+x2)50(x+1x)−10
View SolutionThe number of terms with integral coefficients in the expansion of (1713+3512)600 is (A) 100 (B) 50 (C) 150 (D) 101
View SolutionThe coefficient of xn in the expansion of (1+x)2(1−x)3, is
View SolutionThe sum of the coefficients in the expansion of (1−x+x2−x3)n ,is
View SolutionFind the coefficient of x50 in the expansion of (1+x)101×(1−x+x2)100.
View SolutionThe coefficient of x5 in the expansion of (1+x1!+x22!+x33!+x44!+x55!)2 is
View SolutionThe coefficient of x1274 in the expansion of (x+1)(x−2)2(x+3)3(x−4)4…(x+49)49(x−50)50 is
View SolutionFind the largest term in the expansion of (3+2x)50,wherex=1/5.
View SolutionIf |x|<1, then find the coefficient of xn in the expansion of (1+2x+3x2+4x3+)1/2.
View SolutionFind coeff. of x25 in the expansion of
50∑k=0(−1)k50Ck(2x−3)50−k(2−x)k.View Solution