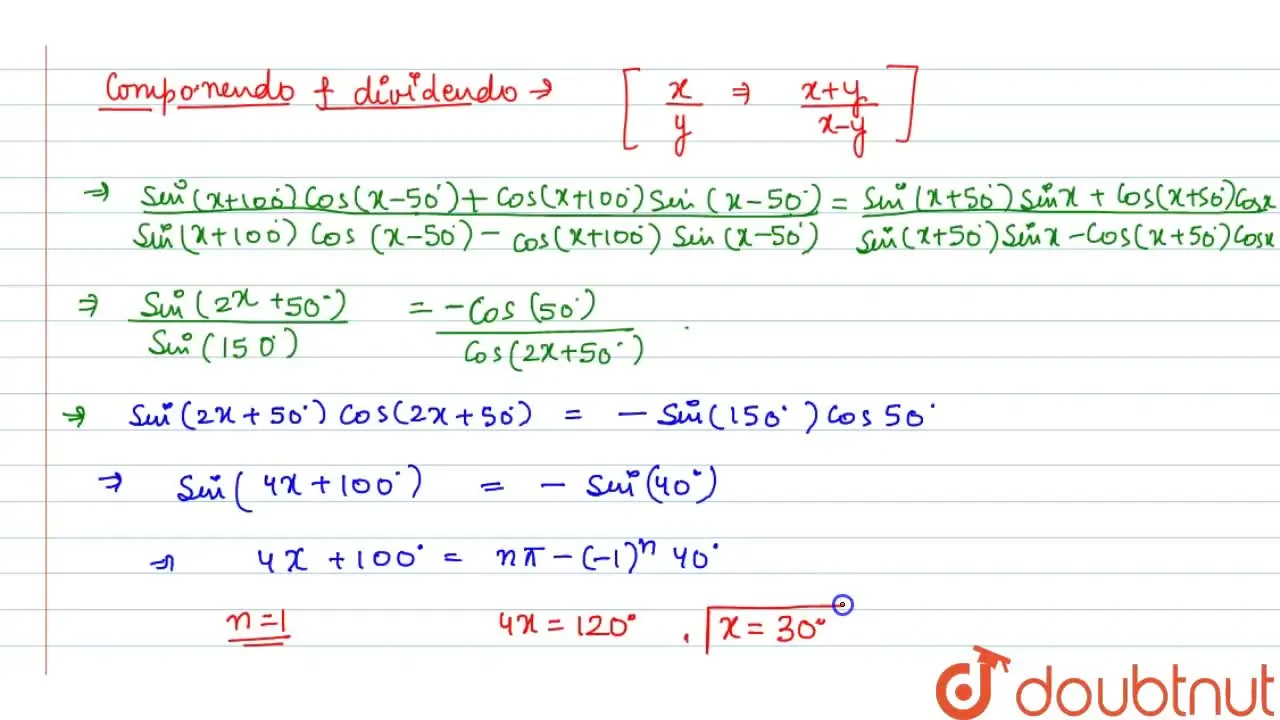
The smallest positive value of x (in degrees) for which tan(x+100∘)=tan(x+50∘).tanx.tan(x−50∘) is
The correct Answer is:x=30∘
Step by step video & image solution for The smallest positive value of x (in degrees) for which tan(x+100^@)=tan(x+50^@).tanx.tan(x-50^@) is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Solved Examples : Single Option Correct Type Questions2videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise For Session 110videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)12videos
TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)9videos
VECTOR ALGEBRA
Similar Questions
The smallest positive values of x and y which satisfy tan(x−y)=1,sec(x+y)=2√3, are
View SolutionFind the set of values of x for which tan3x−tan2x1+tan3x.tan2x=1.
View SolutionFind the set of values of x for which tan3x−tan2x1+tan3xtan2x = 1
View Solution∫tanx.tan2x.tan3xdx=
View SolutionThe value of tan20∘+2tan50∘−tan70∘, is
View Solutiontan 5x tan3x tan2x=
View SolutionThe value of x that satisfies tan−1(tan−3)=tan2x is
View SolutionThe general solution of the equation tanx+tan2x+tanx.tan2x=1 is
View SolutionThe value of tan10∘tan50∘tan70∘ is
View SolutionFind (in each case, given below) the value of x, if :
tanx=tan60∘−tan30∘1+tan60∘tan30∘View Solution