The pyramid in Figure composed of a square base of area 16 and four isoceles triangles, in which each base angle measures 60∘. What is the volume of the pyramid ?
7.39
9.24
15.08
21.33
The correct Answer is:C
The sides of the pyramid are actually equilateral (the base angles are each 60∘, which means the last angle is also 60∘). To find the volume of a pyramid, you need the area of the base, which is given here as 16, and you need the height, which you have to figure out. Imagine a triangle that includes the height and one of the lateral edges :
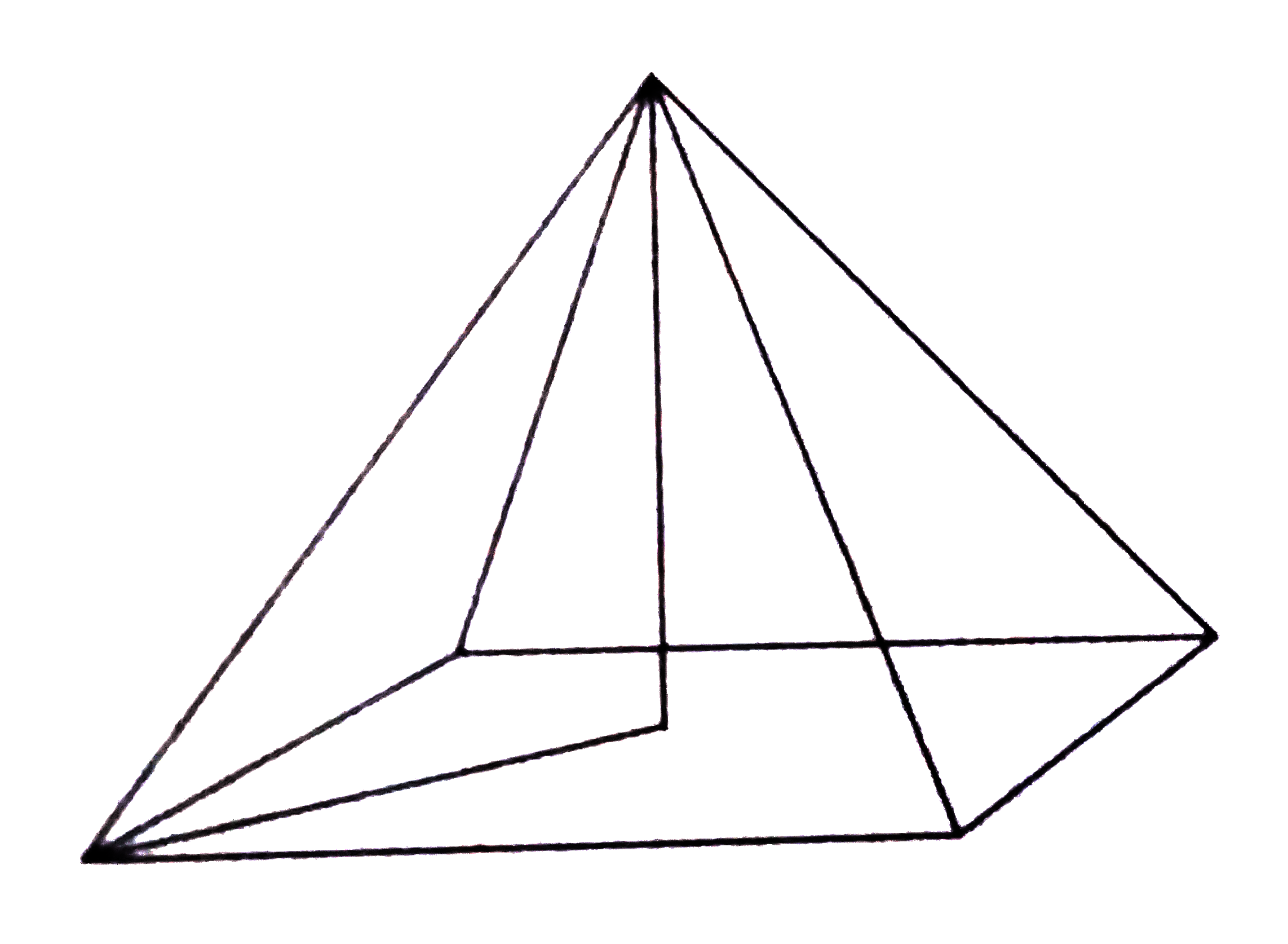
This triangle is a right triangle. The hypotenuse is the same as a side of one of the equilateral triangles, which is the same as a side of the square, which is the square root of 16, or 4 :
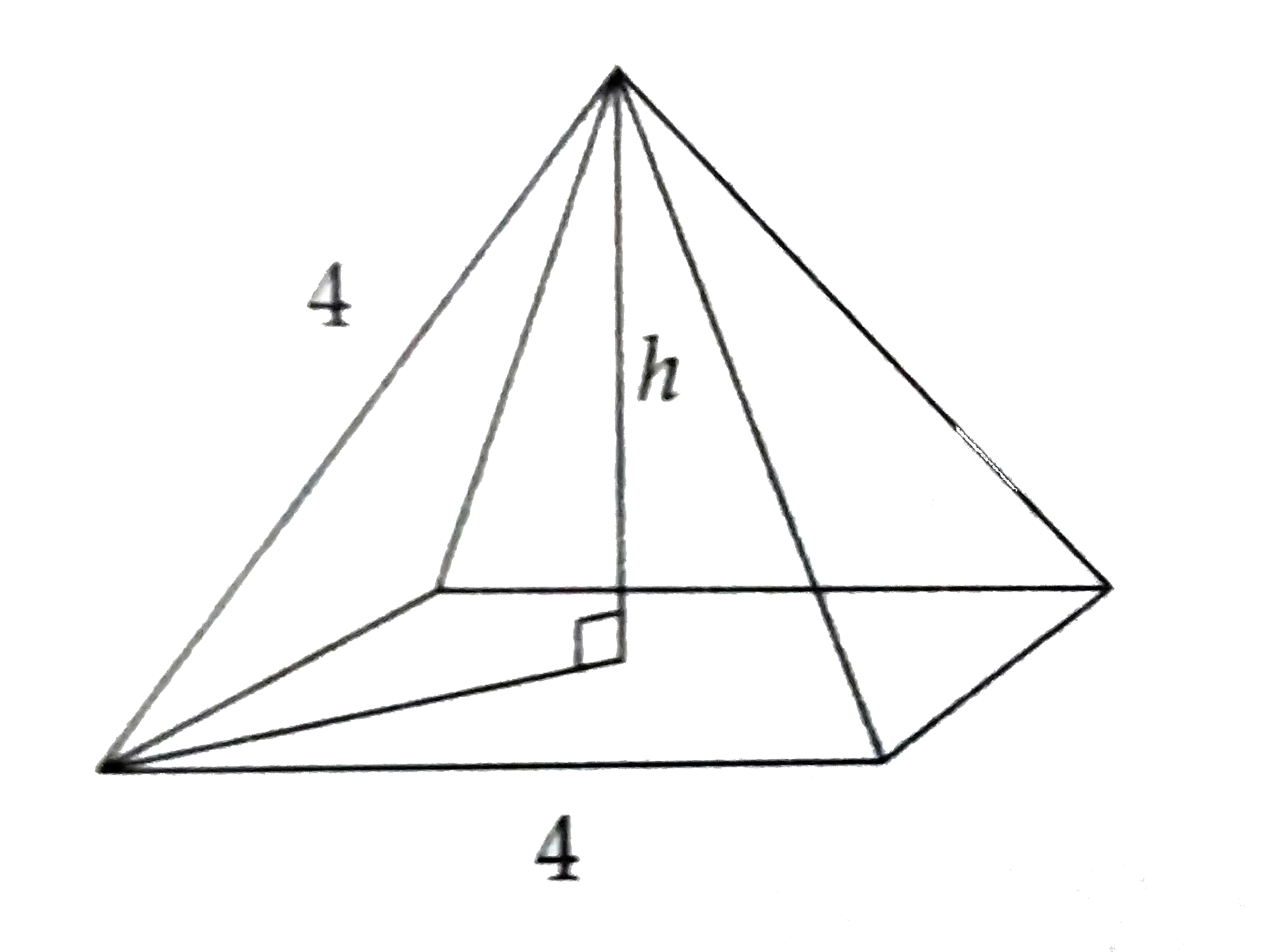
One of the legs of this triangle is half of a diagonal of the square base - that is, half of 4√2, or 2√2.
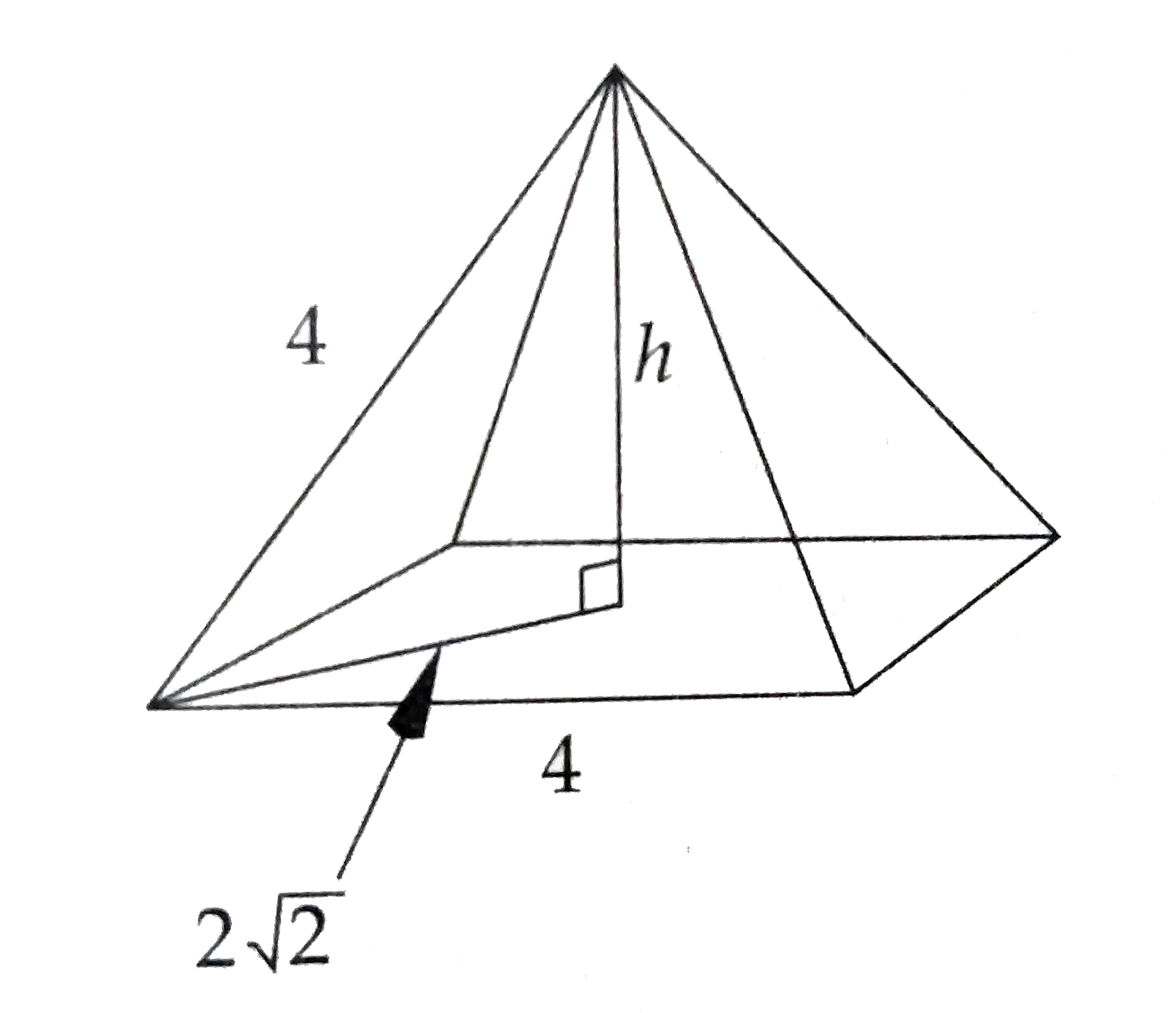
Now you can use the Pythagorean theorem to find the height :
(2√2)2+h2=16
8+h2=16
h2=8
h=√8=2√2
Finally, you have what you need to use the volume formula :
Volume =13Bh=13(16)(2√2)=32√23≈15.08
Related Playlists
- BOOK - KAPLANCHAPTER - SOLID GEOMETRY EXERCISE - SOLID GEOMETRY FOLLOW - UP TEST5videos
SOLID GEOMETRY
- BOOK - KAPLANCHAPTER - SIMILARITY, CONGRUENCE, AND PROOFSEXERCISE - Multiple Choice Question10videos
SIMILARITY, CONGRUENCE, AND PROOFS
- BOOK - KAPLANCHAPTER - SYSTEM OF LINEAR EQUATION EXERCISE - Multiple Choice Question20videos
SYSTEM OF LINEAR EQUATION
Similar Questions
In an isosceles triangle, each base angle is four times its vertical angle. Find each angle of the triangle.
View SolutionFind the area of an isosceles triangle with perimeter 36 cm and base 16 cm.
View SolutionFind the area of an isosceles triangle which has base 12 cm and equal sides are 10 cm each.
View SolutionIf one of the base angles of an isosceles triangle measures 30∘, find the measures of the remaining two angles ?
View SolutionAn isosceles triangle has base 20cm and each of the two equal sides measures 18 cm. What is its perimeter?
View SolutionFind the area of a figure formed by a square of side 8 cm and an isosceles triangle with base as one side of the square and perimeter as 18 cm.
View SolutionThe vertical angle of an isosceles triangle is twice the sum if its base angles. Find each angle of the triangle.
View SolutionA pyramid is a solids figure, the base of which is
View SolutionConstruct on isosceles ΔABC with base BC=3 cm and each base angle measuring 75∘.
View SolutionThe base of an isosceles triangle is 24 cm and its area is 60cm2Find its perimeter.
View Solution


