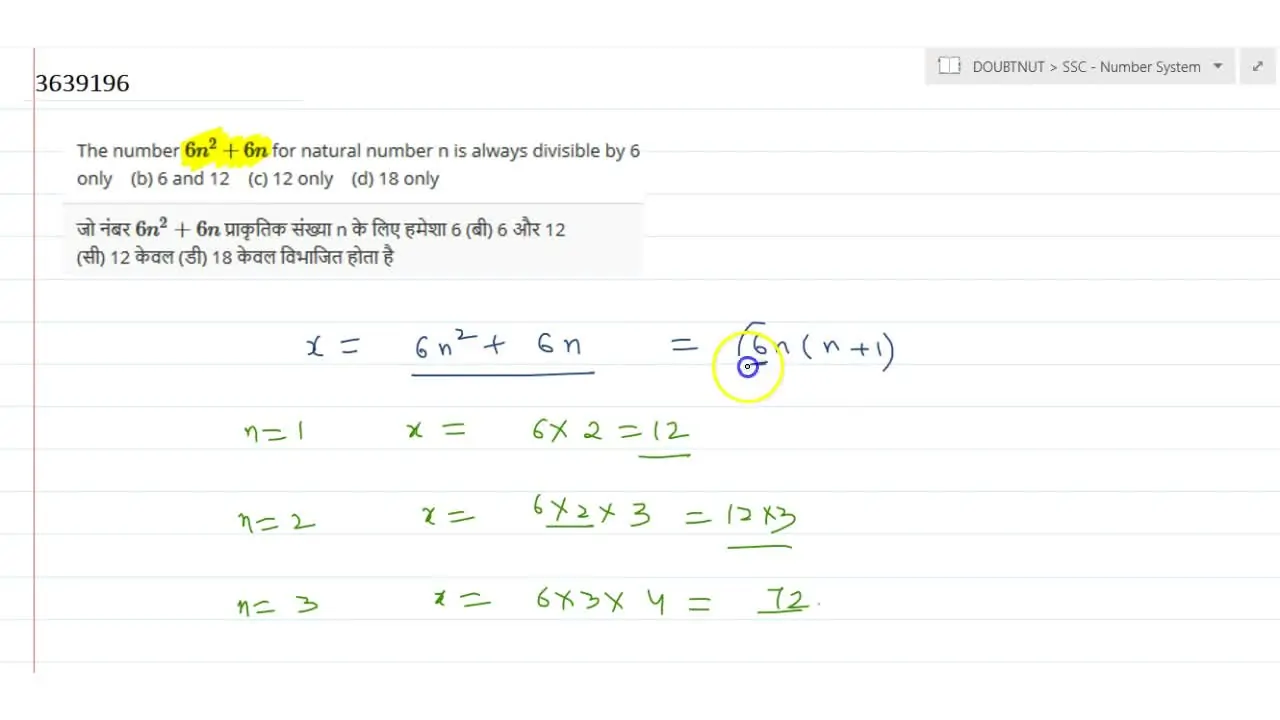
The number 6n2+6n for natural number n is always divisible by 6 only (b) 6 and 12 (c) 12 only (d) 18 only
Step by step video & image solution for The number 6n^2+6n for natural number n is always divisible by 6 only (b) 6 and 12 (c) 12 only (d) 18 only by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
The product of n consecutive natural number is always divisible by
View SolutionThe product of n consecutive natural numbers is always divisible by
View SolutionThe product of the predecessor and successor of an odd natural number is always divisible by 2 (b) 4 (c) 6 (d) 8
View SolutionThe expression 26n−42n where n is a natural number is always divisible by
View SolutionA 6-digit number is formed by repeating a 3-digit number: for example, 256256 or 678678 etc. Any number of this form is always exactly divisible by 7 only (b) 11 only (c) 13 only (d) 1001
View SolutionThe expression 26n−42n, where n is a natural number is always divisible by
View SolutionIf n is a natural number, then 92n−42n is always divisible by 5 (b) 13 (c) both 5 and 13 (d) none of these
View SolutionIf n be any natural number then by which largest number (n3−n) is always divisible? 3 (b) 6 (c) 12 (d) 18
View SolutionIf n is a whole number greater than 1, then n2(n2−1) is always divisible by 8 (b) 10 (c) 12 (d) 16
View SolutionIf p is a prime number greater than 3, then (p2−1) is always divisible by (a) 6 but not 12 (b) 12 but not 24 (c) 24 (d) None of these
View Solution