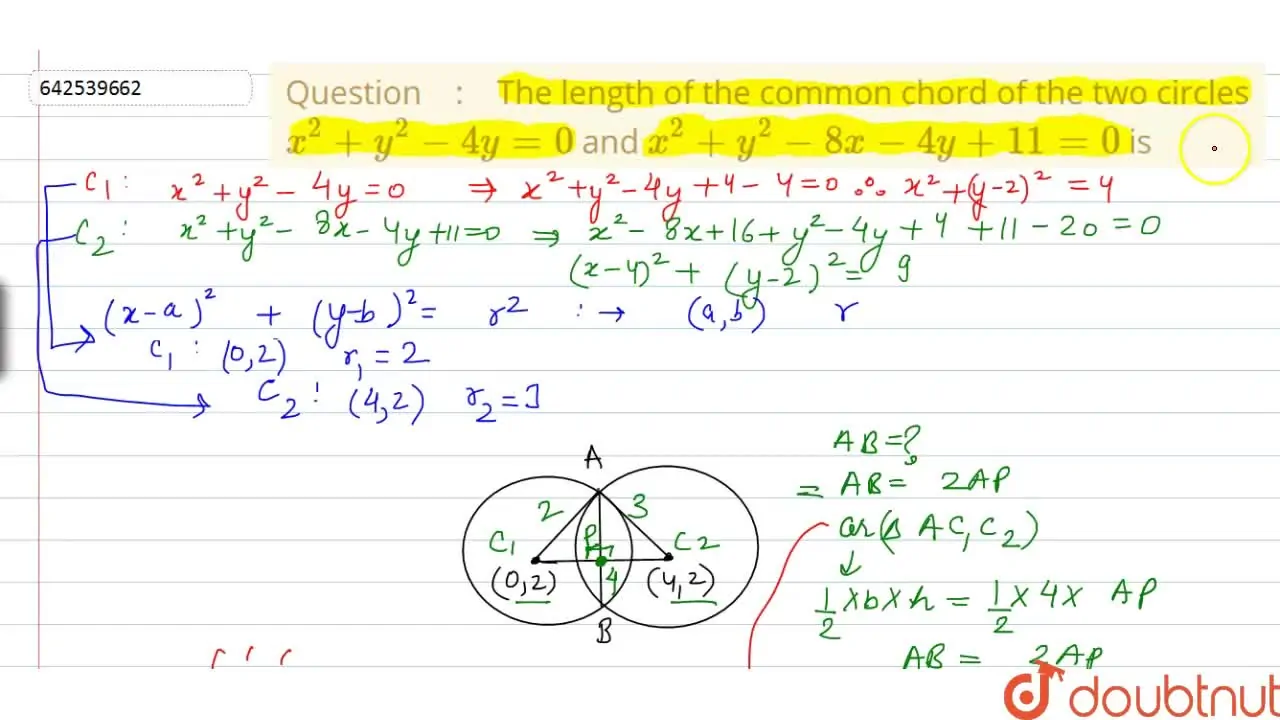
The length of the common chord of the two circles x2+y2−4y=0 and x2+y2−8x−4y+11=0 is
2√11
3√2
6√3
8√2
The correct Answer is:C
Step by step video & image solution for The length of the common chord of the two circles x^2+y^2-4y=0 and x^2+y^2-8x-4y+11=0 is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 6 (Ellipse)3videos
JEE 2019
- BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 7 (Hyperbola)7videos
JEE 2019
- BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 4 (Circle)10videos
JEE 2019
- BOOK - CENGAGE ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Archives (Numerical Value type)2videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - LIMITSEXERCISE - Comprehension Type4videos
LIMITS
Similar Questions
Find the length of the common chord of the circles x2+y2+2x+6y=0 and x2+y2−4x−2y−6=0
View SolutionThe length of the common chord of the circles x2+y2−2x−1=0 and x2+y2+4y−1=0, is
View SolutionThe length of the common chord of the circles x2+y2+ax+by+c=0 and x2+y2+bx+ay+c=0 is
View SolutionFind the equation and length of the common chord of the two circles
S=x2+y2+3x+5y+4=0 and S=x2+y2+5x+3y+4=0View SolutionIf the length of the common chord of two circles x2+y2+8x+1=0 and x2+y2+2μy−1=0 is 2√6 , then the values of μ are ±2 (b) ±3 (c) ±4 (d) none of these
View SolutionCommon chord of the circles x2+y2−4x−6y+9=0,x2+y2−6x−4y+4=0 is
View SolutionShow that the common chord of the circles x2+y2−6x−4y+9=0 and x2+y2−8x−6y+23=0 is the diameter of the second circle and also find its length.
View SolutionThe two circles x2+y2−5=0 and x2+y2−2x−4y−15=0
View SolutionThe equation of the circle described on the common chord of the circles x2+y2−4x+5=0andx2+y2+8y+7=0 as a diameter, is
View SolutionThe length of the common chord of the circles (x−6)2+(y−4)2=4,(x−4)2+(y−6)2=4 is
View Solution