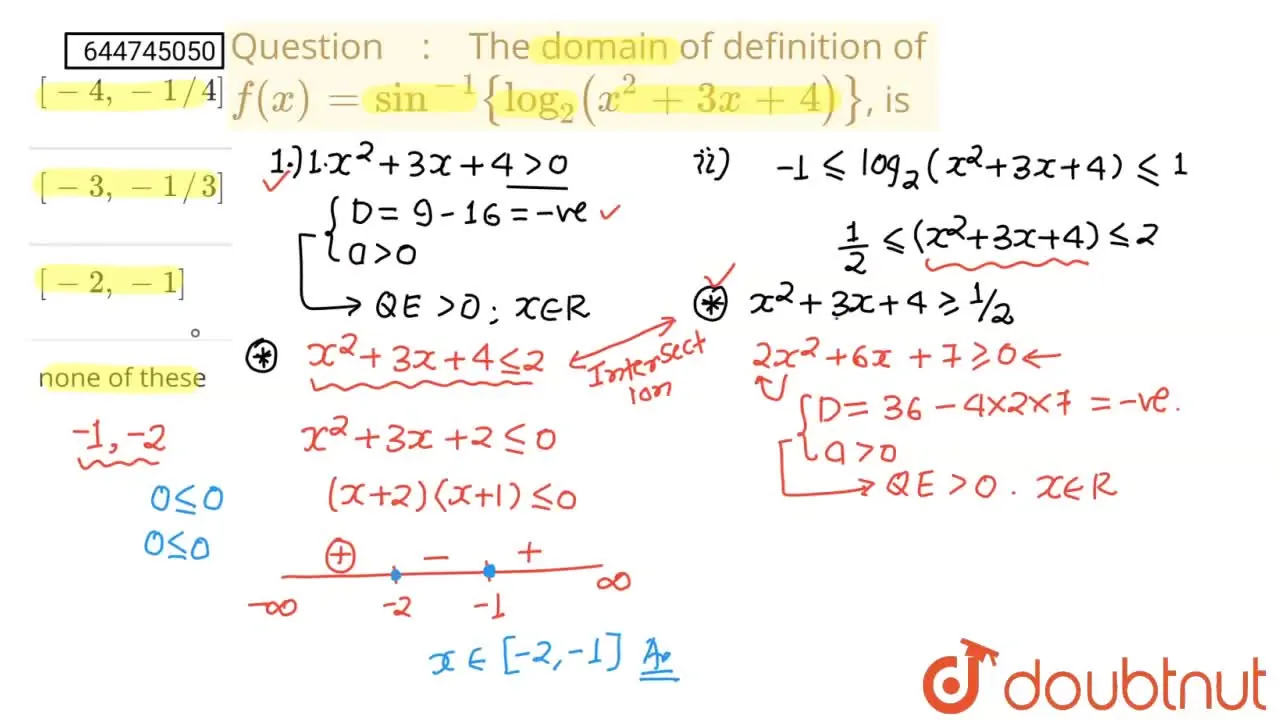
The domain of definition of , is
A
B
C
D
none of these
Video Solution
Text Solution
The correct Answer is:C
f(x) is denfined, if
rArr x in [-2,-1]
|
Updated on:21/07/2023
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - REAL FUNCTIONS EXERCISE - Section II - Assertion Reason Type8videos
REAL FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - REAL FUNCTIONS EXERCISE - Exercise94videos
REAL FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - REAL FUNCTIONS EXERCISE - Chapter Test58videos
REAL FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMEXERCISE - Chapter Test55videos
PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEM
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SCALAR AND VECTOR PRODUCTS OF THREE VECTORS EXERCISE - Exercise63videos
SCALAR AND VECTOR PRODUCTS OF THREE VECTORS
Similar Questions
The domain of definition of f(X) = sin−1(−x2) is
View SolutionThe domain of definition of f(x)=√ecos−1(log4x2)is
View SolutionThe domain of definition of the function
f(x)=sin−1{log2(x22)}, isView SolutionThe domain of definition of f(x)=log3|logex|, is
View SolutionThe domain of the function f(x)=sin−1log3(x3)) is
View SolutionFind the domain of definition of f(x)=cos−1(x2−4) .
View SolutionThe domain of definition of f(x)=log2(log3(log4x)), is
View SolutionThe domain of definition of f(x)= ⎷sec−1{1−|x|2} is
View SolutionFind the domain of the function : f(x)=sin−1((log)2x)
View SolutionFind the domain of the function : f(x)=sin−1((log)2x)
View Solution