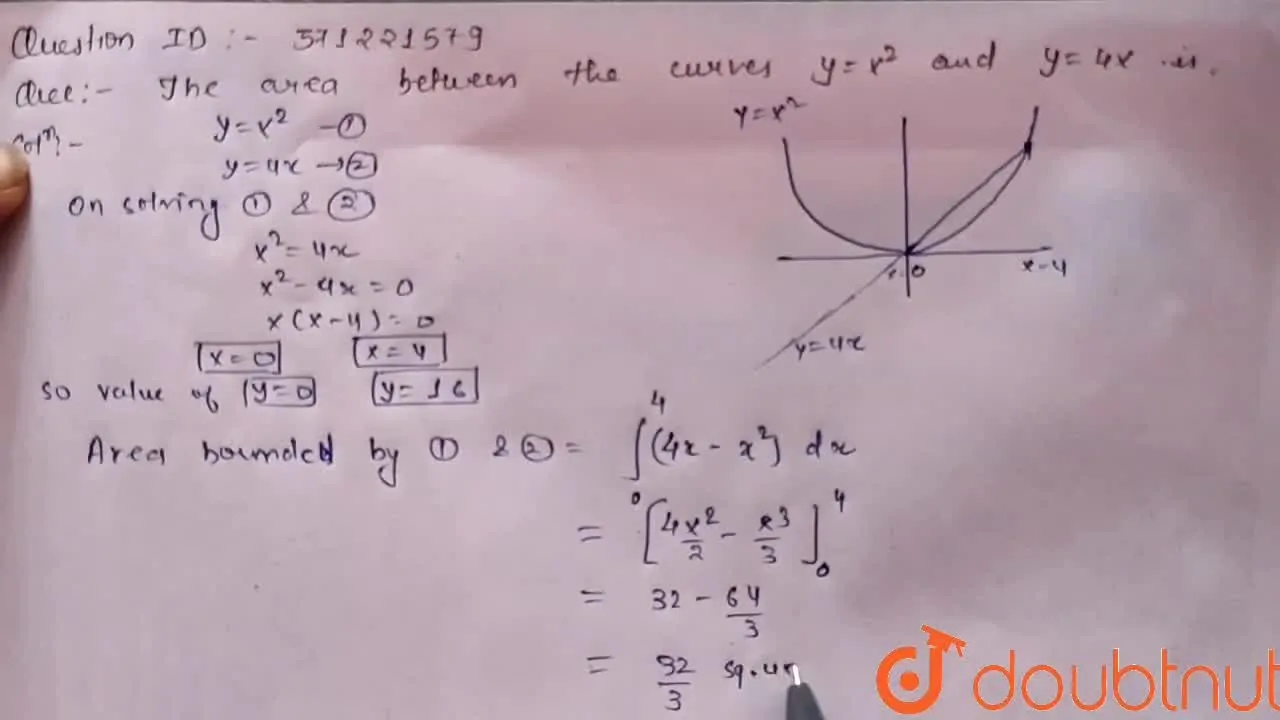
the area between the curves y=x2 and y=4x is
Given curves are x2=yandy=4x
Intersection points are (0,0)and(4,16)
Required Area =∫40(4x−x2)dx
[4x22−x33]40
[32−643]
=323sq unit
Related Playlists
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - SOLVED EXAMPLES15videos
APPLICATION OF INTEGRALS
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Exercise 8.113videos
APPLICATION OF INTEGRALS
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - SOLVED EXAMPLES51videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5.717videos
CONTINUITY AND DIFFERENTIABILITY
Similar Questions
Find the area between the curves y=xand y=x2.
View SolutionThe angle between the curves y=x2 and y=4−x2 is
View SolutionFind the area between the curve y=x and y=x2
View SolutionThe area between the curves y=x3 and y=x+|x| is equal to
View SolutionThe area between the curves y=x2 and y=21+x2 is equal to
View SolutionThe ratio of the areas between the curves y=cosxandy=cos2x and x-axis from x=0 to x=π3 is
View SolutionUsing integration , find the area bounded between the curve y=x2andy=−|x|+2
View SolutionShow that the area enclosed between the curves y = x and y=x3 is 12 sq unit.
View SolutionFind the area enclosed between the curves
y=4x2 and y=x2+3View SolutionIf A is the area lying between the curve y=sinxand x-axis between x=0 andx=π/2 . Area of the region between the curve y=sin2xandx-axis in the same interval is given by
View Solution