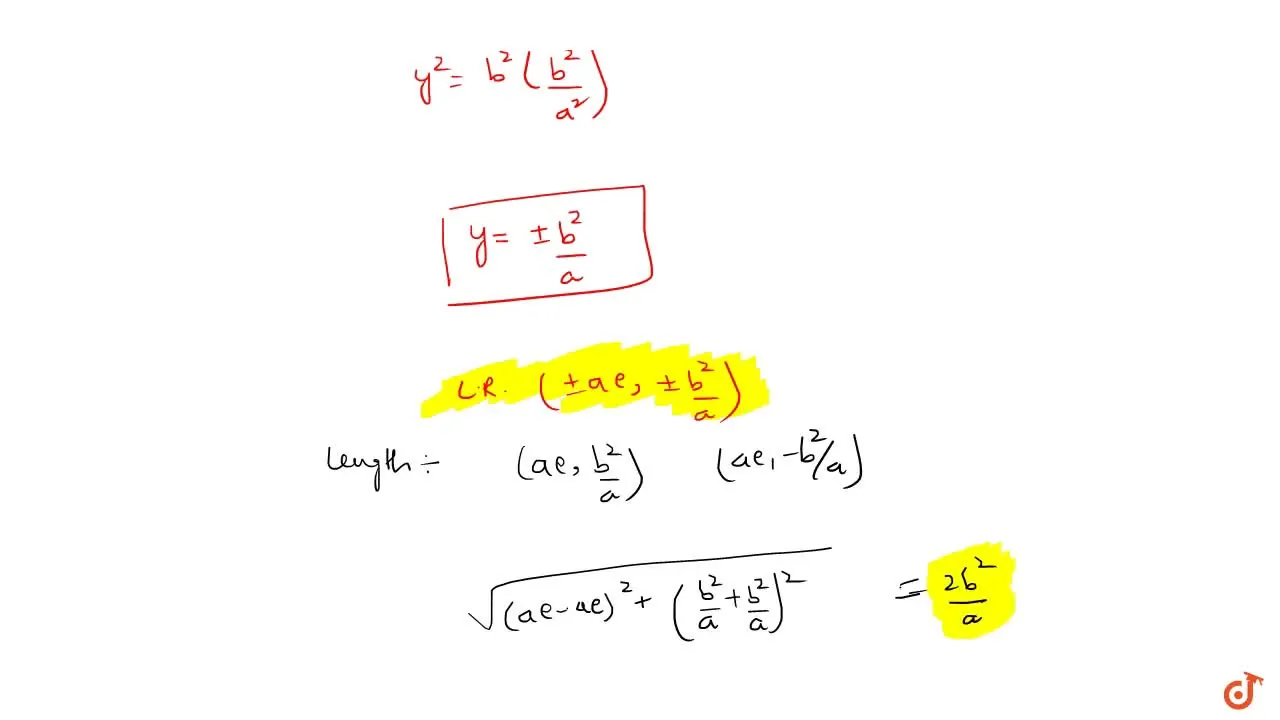
Some important terms of ellipse :- Ordinate; Double ordinate ;latus rectum.
Step by step video & image solution for Some important terms of ellipse :- Ordinate; Double ordinate ;latus rectum. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
" If the distance between the foci of an ellipse is half the length of its latus rectum,then the eccentricity of the ellipse is: "
View SolutionIf the distance between the foci of an ellipse is half the length of its latus rectum,then the eccentricity of the ellipse is
View SolutionIf the distance between the foci of an ellipse is 8 and length of latus rectum is 185, then the eccentricity of ellipse is:
View SolutionIf the distance between the foci of an ellipse is 8 and length of latus rectum is 185, then the eccentricity of ellipse is:
View SolutionThe triangle formed by the tangents to a parabola y2=4ax at the ends of the latus rectum and the double ordinate through the focus is
View SolutionThe eccentricity of the ellipse is 25 and the distance between the foci is 10. Find the length of the latus rectum of the ellipse.
View SolutionEquation of an ellipse whose axes are parallel to co-ordinate axes and center is (h,k)
View SolutionLet p be a point on the parabola y2=4ax then the abscissa of p ,the ordinates of p and the latus rectum are in
View SolutionFind the equation of the parabola whose vertex is (2,3) and the equation of latus rectum is x=4. Find the co-ordinates of the point of intersection of this parabola with its latus rectum.
View SolutionThe locus of the point of trisection of all the double ordinates of the parabola y2=lx is a parabola whose latus rectum is - (A) l9 (B) 2l9 (C) 4l9 (D) l36
View Solution