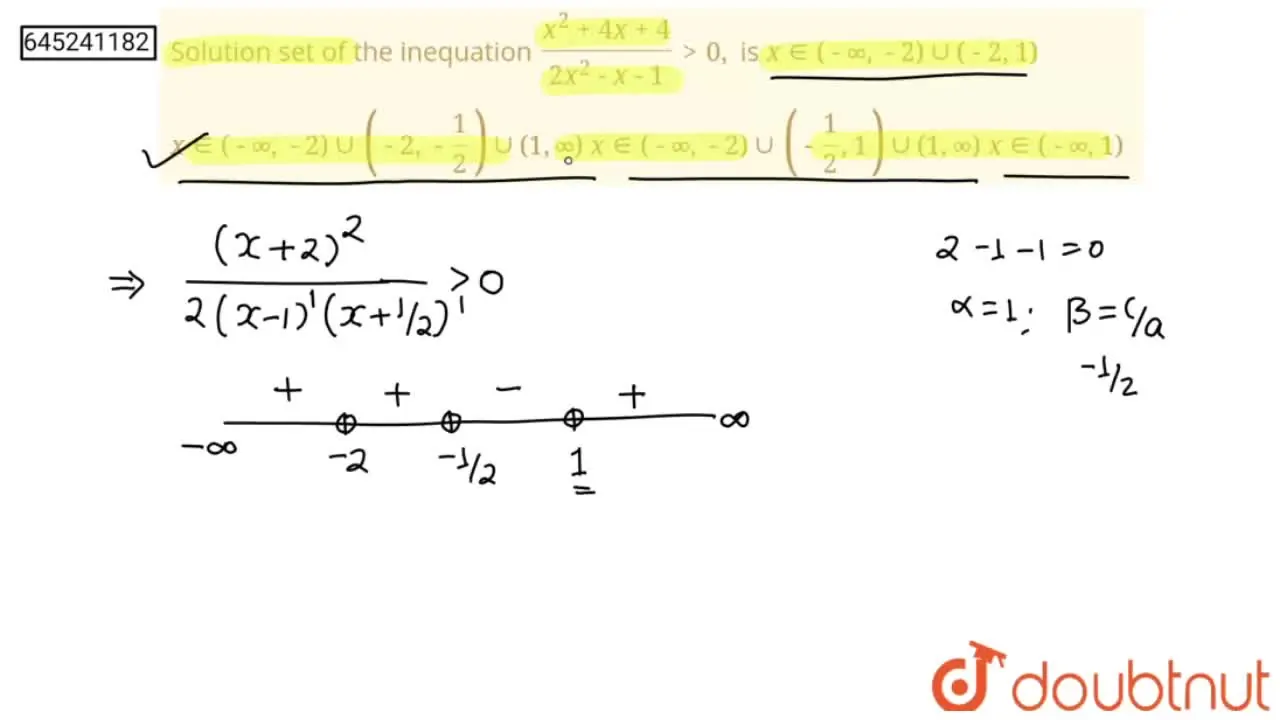
Solution set of the inequation x2+4x+42x2−x−1>0, is x∈(−∞,−2)∪(−2,1) x∈(−∞,−2)∪(−2,−12)∪(1,∞) x∈(−∞,−2)∪(−12,1)∪(1,∞) x∈(−∞,1)
Step by step video & image solution for Solution set of the inequation (x^2+4x+4)/(2x^2-x-1)>0, is x in (-oo,-2)uu(-2,1) x in (-oo,-2)uu(-2,-1/2)uu(1,oo) x in (-oo,-2)uu(-1/2,1)uu(1,oo) x in (-oo,1) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Solution set of the inequation, x2−5x+6x2+x+1<0 is x∈(−∞,2) (b) x∈(2,3) x∈(−∞,2)∪(3,∞) (d) x∈(3,∞)
View SolutionComplete solution set of the inequation (x2−1)(x2−6x+8)≥0 is
(a) x∈[−1,1]∪[2,4]
(b) x∈(−∞,−1)∪[1,2]∪[4,∞]
(c) x∈[−1,2]∪[4,∞]
(d) none of theseView SolutionSolution set of the inequality (log)0.8((log)6x2+xx+4)<0 is (−4,−3) (b) (−3,4)∪(8,∞) (−3,∞) (d) (−4,−3)∪(8,∞)
View SolutionSolution set of the inequality (log)0.8((log)6x2+xx+4)<0 is
View SolutionThe solution set of the inequality max {1−x2,|x−1|}<1 is (−∞,0)∪(1,∞) (b) (−∞,0)∪(2,∞) (0,2) (d) (0,2)
View SolutionThe domain of the function f(x)=√log(|x|−1)(x2+4x+4) is (a) (−3,−1)∪(1,2) (b)(−2,−1)∪(2,∞) (c)(−∞,−3)∪(−2,−1)∪(2,∞) (d)none of these
View SolutionSolution set of the inequality sin−1(sin(2x2+3x2+1))≤π−52 is- a.(−∞, 1)∪(1, ∞) b. [−1, 1] c.(−1, 1) d. (−∞,−1]∪[1, ∞)
View SolutionIf solution set of Inequality (x2+x−2)(x2+x−16)≥−40 is xε(−∞,−4]∪[a,b]∪[c,∞) then a+b−c is
View SolutionIf |x−1|>5, then a. x∈(−4,6) b. x∈[−4,6] c. x∈(−∞,−4)∪(6,∞) d. x∈(−∞,−4)∪[6,∞)
View SolutionSolution set of inequality (ex−1)(2x−3)(x2+x+2)(sinx−2)(x+1)2x≤0 is [32,∞) b. (−∞,−1)∪[32,∞) c. [−1,0)∪[32,∞) d. R−{0,−1}
View Solution