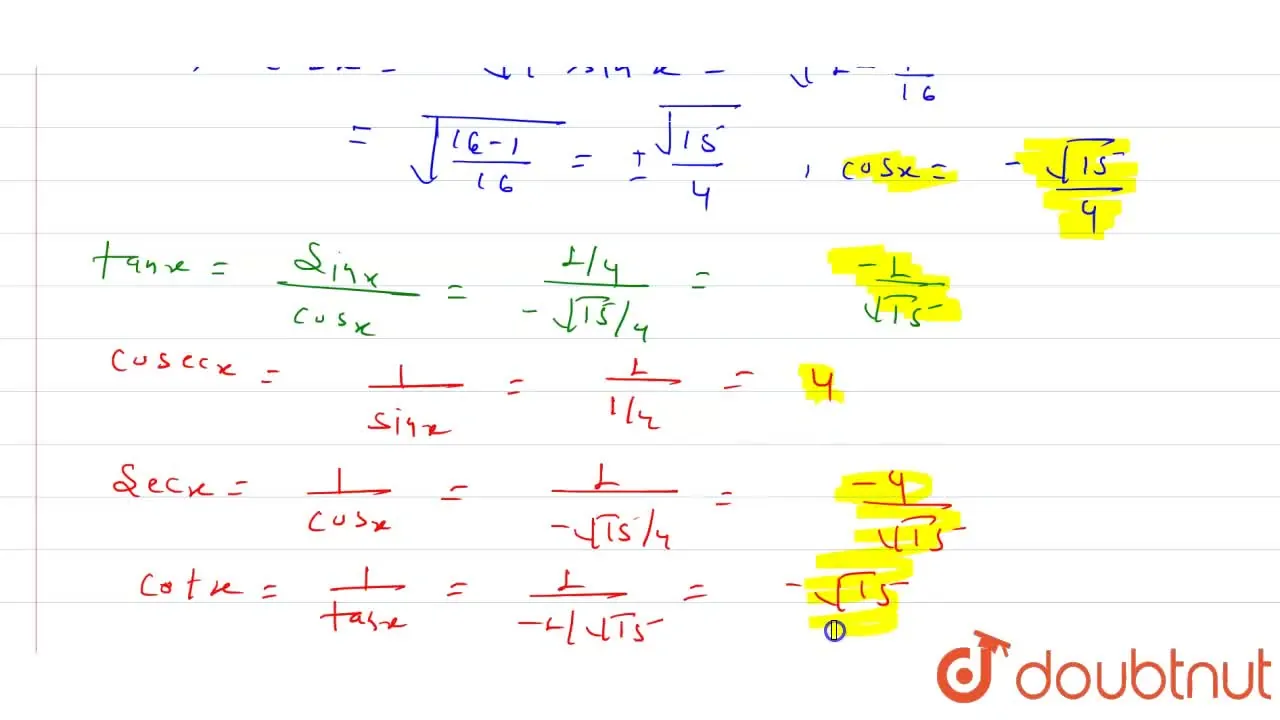
sinx=14, x in quadrant II. Find the values of other five trignometric functions
sinx=14 and sin2x+cos2=1
⇒(14)2+cos2x=1
⇒cos2x=1−16=1516
⇒cosx=−√154 (since x lies in 2nd quadrant)
∴cosx=−√154⇒2cos2x2−1=−√154
=2cos2x2=1−√154=4−√158
⇒cosx2=√4−√158=√8−2√1516
⇒cosx2=√8−2√154
∴ x lies in 2nd quadrant ⇒π2<x<π
then π2 will be lie in first quadrant in which all ratios will be positive.
∴cosx2=√8−2√154
∴cos2x2=8−2√1516 then sin2x2=1−cos2x2
or sin2x2=8+2√1516
or sinx2=√8+2√154
Now, tanx2=sinx2cosx2=√8+2√154√8−2√154
√8+2√158−2√15×√8+2√15√8−2√15
=√8+2√152(8)2−(2√15)2=8+2√15√64−60
8+2√152=4+√15
Therefore, sinx2=√8+2√154,cosx2=√8−2√154 and tanx2=4+√15 Ans.
Related Playlists
Similar Questions
cosx=−13, x in quadrant III. Find the values of other five trignometric functions
View SolutionIf cosecθ=53 and θ lies in Quadrant II find the values of all the other five trigonometric functions.
View SolutionIf cos θ=−√32and θ lies in Quadrant III find the values of all the other five trigonometric functions .
View Solutionlf cosx = −35,x lies in the third quadrant, find the values of other five trignometric function.
View SolutionIf cotx=−512, lies in second quadrant, find the values of other five trigonometric functions.
View SolutionIf cosx=−35, x lies m the third quadrant, find the values of other five trigonometric functions.
View SolutionIf sin θ=−12 and θ lies in Quadrant IV , find the values of all the other five trigonometric fucntions.
View SolutionIf secθ=−1312 and θ lies in the second quadrant then find the values of all the other five trigonometric functions
View SolutionIf secθ=√2andθ lies in Quadrant IV, find the values of all the other trigonometric functions.
View Solutionif sec x=−2 and π<x<.3π2 find the values of all the other five trigonometric functions .
View Solution