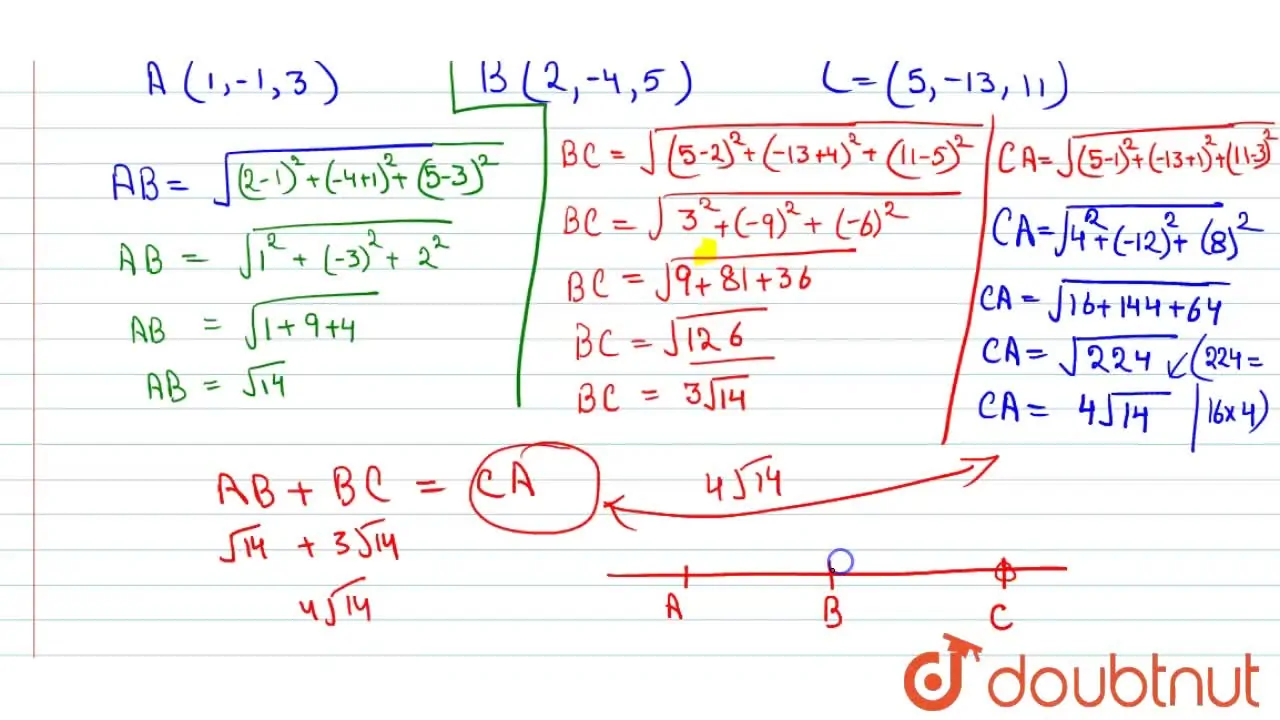
Show that the point A (1,-1,3), B ( 2,-4,5) and C ( 5,-13,11) are collinear.
Given points , A (1,-1,3), B ( 2,-4,5) and C ( 5,-13,11)
AB=√(1−2)2+(−1+4)2+(3−5)2
√1+9+4=√14
BC=√(2−5)2+(−4+13)2+(5+11)2
√9+81+36=√126
AC=√(1−5)2+(−1+13)2+(3−11)2
√16+144+64=√224
AB + BC+AC
√14+√126=√224
√14+3√14=4√14 ltbr> So, the points A,B, and C are collinear.
Related Playlists
- BOOK - NCERT EXEMPLARCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - Long Answer type Questions4videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
- BOOK - NCERT EXEMPLARCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - Objective type Questions13videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
- BOOK - NCERT EXEMPLARCHAPTER - CONIC SECTIONSEXERCISE - Objective type13videos
CONIC SECTIONS
- BOOK - NCERT EXEMPLARCHAPTER - LIMITS AND DERIVATIVES EXERCISE - FILLERS4videos
LIMITS AND DERIVATIVES
Similar Questions
Using vector method, show that the points A(2,−1,3),B(4,3,1)andC(3,1,2) are collinear
View SolutionUsing vector method show that the points A(-5, 7) B(-4, 5) and C(1, -5) are collinear.
View SolutionShow by using direction ratios, that the points (2,−4,5),(1,−1,3)and(5,−13,11) are collinear
View SolutionUsing direction ratios show that the points A(2,3,-4), B(1,-2,3) and C(3,8,-11) are collinear.
View SolutionProve that the points A(2,0,−3),B(1,−2,−5) and C(3,2,−1) are collinear.
View SolutionUsing distance formula, show that the points (1,5),(2,4)and(3,3) are collinear.
View SolutionShow that the points A(1,−2,−8),B(5,0,−2)andC(11,3,7) are collinear, and find the ratio in which B divides AC.
View SolutionShow that the points A(5, 4, 2), B(6, 2, -1) and C(8, -2, -7) are collinear.
View SolutionUsing slopes, show that the points A(6, -1), B(5, 0) and C(2, 3) are collinear.
View SolutionProve that the point A(1,2,3),B(−2,3,5) and C(7,0,−1) are collinear.
View Solution