Show that the normal at any point θ to the curve x=acosθ+aθsinθ, y=asinθ−a θcosθ is at a constant distance from the origin.
First, let us find the slope of the tangent to the curve at a point θ.
mT=dydx=dydθdxdθ
=-(asinθ+asinθ+aθcosθ)/(acosθ−acosθ+aθsinθ)
Since, normal is always perpendicular to the tangent, slope of the normal will be
mN=−cosθsinθ
Normal passes through the point (x,y) given in the question. Using the equation of the line in slope-point form,
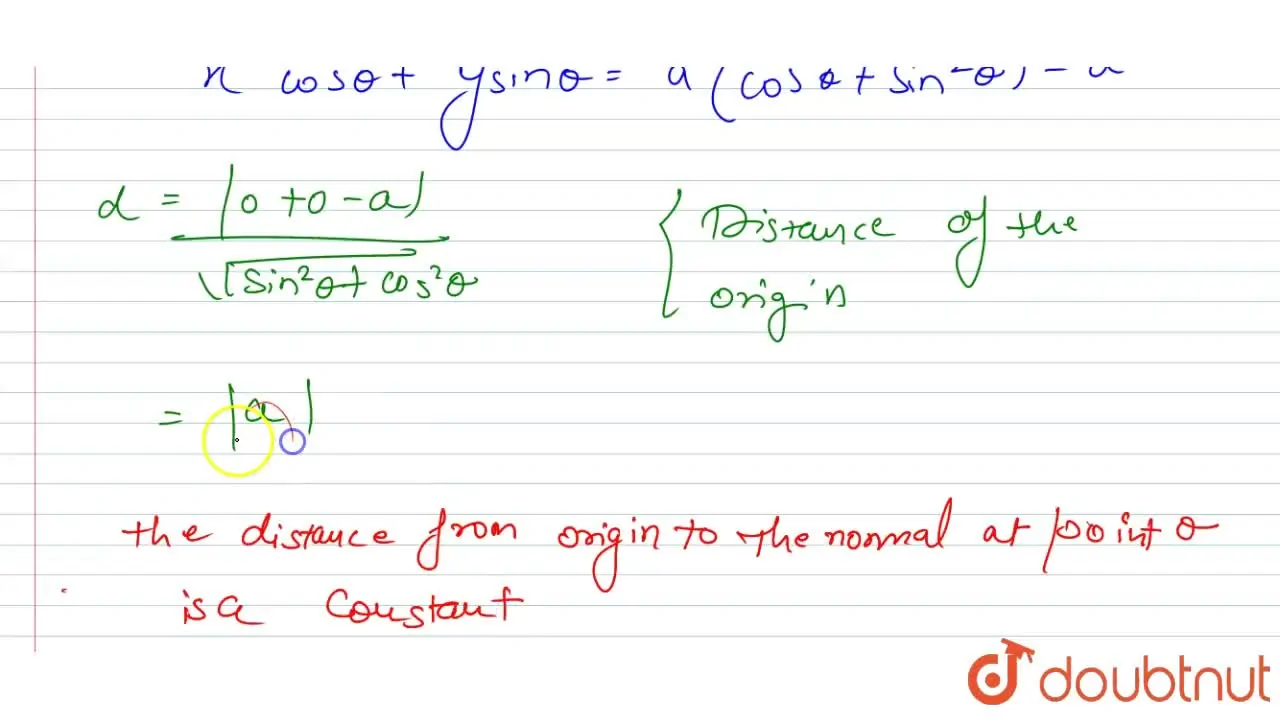
⇒ysinθ−asin2θ+aθsinθcosθ=acos2θ+aθsinθcosθ−xcosθ
⇒xcosθ+ysinθ=a(cos2θ+sin2θ)=a
Distance of origin from this line will be
d=|a|
Hence, the distance from origin to the normal at any point θ is a constant.
Related Playlists
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.49videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.529videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
Similar Questions
The normal to the curve x=a(cosθ+θsinθ),y=a(sinθ−θcosθ) at any θ is such that
View SolutionThe length of normal at any point to the curve, y=ccosh(xc) is
View SolutionFind the equation of the normal to the curve x=acosθ and y=bsinθ at θ
View SolutionProve that all normals to the curve x=acost+atsint, y=asint−atcost are at a distance a from the origin.
View SolutionFind the length of normal to the curve x=a(θ+sinθ),y=a(1−cosθ) at θ=π2.
View SolutionFind the length of normal to the curve x=a(θ+sinθ),y=a(1−cosθ) at θ=π2.
View SolutionFind the slope of the normal to the curve x=a cos3θ , y=a sin3θ at θ=π4 .
View SolutionFind the equations of the tangent and the normal to the curve x=a(θ+sinθ), y=a(1−cosθ) at θ at indicated points.
View SolutionFind the slopes of the tangent and the normal to the curve x=a cos3θ, y=a sin3θ at θ=π/4
View SolutionFind the slopes of the tangent and the normal to the curve x=a(θ−sinθ) , y=a(1+cosθ) at θ=−π/2
View Solution