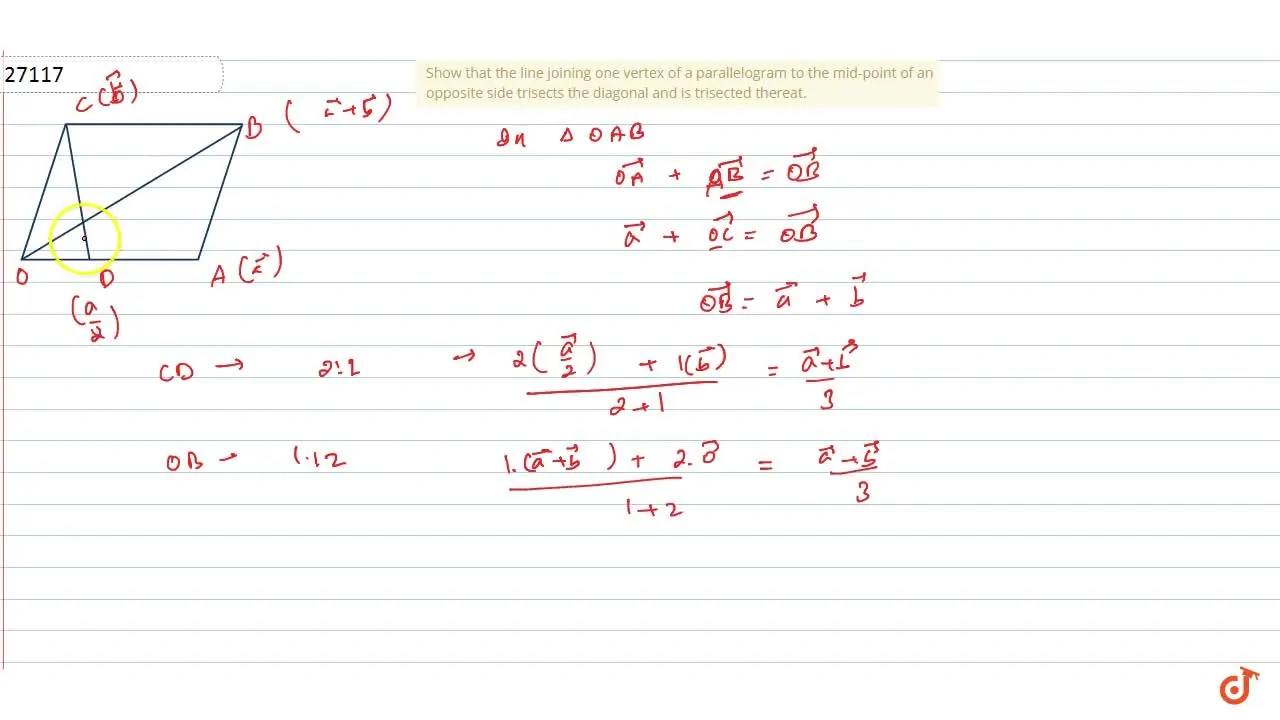
Show that the line joining one vertex of a parallelogram to the mid-point of an opposite side trisects the diagonal and is trisected there at.
Let {OABC} be a parallelogram Table {O} as origin. Let −−→{a} and −−→{b} be position vectors of −−→{A} and −−→{C} such that −−→OA=−−→{a}, −−→OC=−−→{b}.
Now, −−→OB=−−→OA+−−→AB=−−→OA+−−→OC
∴ −−→OB=→a+→b
∴ position vector of B is (→a+→b)
Position vector of mid-point of D of A is →a2
P.V. of a point dividing CD in the ratio
2:1 is 2(→a2)+(1)(→b)2+1 i.e. →a+→b3
Again position vector of point divides OB in the ratio 1:2 is
(1)(→a+→b)+2(→0)1+2 i.e. →a+→b3
∴ position vectors of points trisecting CD and OB are same.
∴CD trisects OB and CD is trisected there at.
Related Playlists
Similar Questions
Show that the line joining one vertex of a parallelogram to the mid-point of an opposite side trisects the diagonal and is trisected there at.
View SolutionShow that the line joining any vertex of a parallelogram to the mid - point of an opposite side divides the opposite diagonal in the ratio 2 : 1.
View SolutionThe line segment joining a vertex of a triangle to the mid-point of its opposite side is called its __________.
View SolutionShow that the line segments joining the mid-points of opposite sides of a quadrilateral bisects each other.
View SolutionShow that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
View SolutionShow that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
View SolutionMedians the line segments joining the vertices to the mid-points of the opposite side of a triangle are known as its medians.
View SolutionShow that the lines joining the mid-points of opposite sides of a quadrilateral bisect each other.
View SolutionIn a parallelogram ABCD, points P and Q are points of trisection of diagonal BD. Prove that CQ is parallel to AP.
View SolutionShow that the area of the triangle formed by joining the extremities of an oblique side of a trapezium to the mid point of opposite side in half that of the trapezium.
View Solution