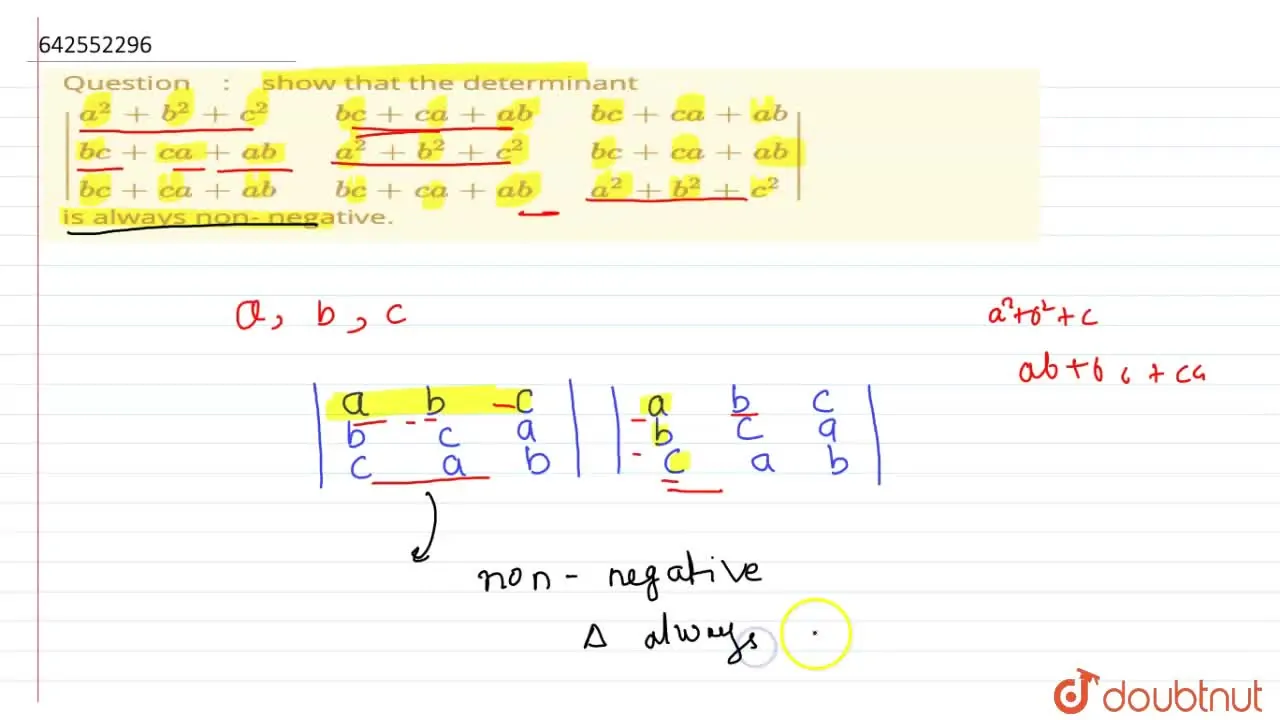
show that the determinant
∣∣
∣
∣∣a2+b2+c2bc+ca+abbc+ca+abbc+ca+aba2+b2+c2bc+ca+abbc+ca+abbc+ca+aba2+b2+c2∣∣
∣
∣∣
is always non- negative.
Video Solution
Text Solution
∣∣
∣
∣∣a2+b2+c2bc+ca+abbc+ca+abbc+ca+aba2+b2+c2bc+ca+abbc+ca+abbc+ca+aba2+b2+c2∣∣
∣
∣∣
=∣∣
∣∣a b cb c ac a b∣∣
∣∣∣∣
∣∣a b cb c ac a b∣∣
∣∣
Which is always non-negative.
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTEXERCISE - Multiple Correct Answer5videos
DETERMINANT
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
Similar Questions
if a +b+c=9 and ab+bc+ca=26,find a2+b2+c2.
View SolutionIf a−b−c=3anda2+b2+c2=77, find: ab−bc+ca
View SolutionThe value of determinant ∣∣ ∣ ∣∣a2a2−(b−c)2bcb2b2−(c−a)2cac2c2−(a−b)2ab∣∣ ∣ ∣∣ is
View SolutionFind : a2+b2+c2, if a+b+c=9andab+bc+ca=24
View SolutionIf a+b+c=9anda2+b2+c2=29, find ab+bc+ca.
View SolutionSimply ∣∣ ∣ ∣∣abca2b2c2bccaab∣∣ ∣ ∣∣
View SolutionProve the identities: ∣∣ ∣ ∣∣b2+c2abacabc2+a2bccabca2+b2∣∣ ∣ ∣∣=4a2b2c2
View SolutionIf a+b+c=11anda2+b2+c2=81, find : ab+bc+ca.
View SolutionIf a+b−c=4anda2+b2+c2=38, find ab−bc−ca
View SolutionIf a+b+c=pandab+bc+ca=q, find a2+b2+c2
View Solution