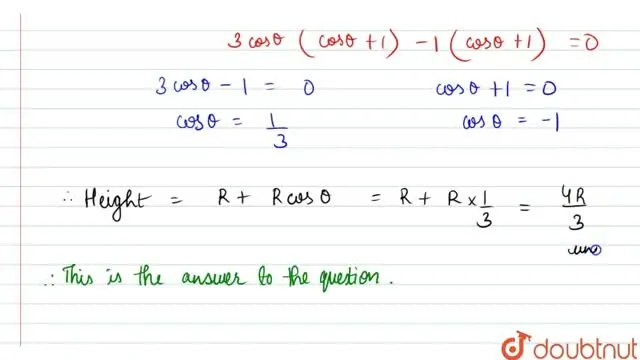
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius R is 4R3.
Step by step video & image solution for Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius R is (4R)/3dot by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is 4r/3. Also, find maximum volume in terms of volume of the sphere.
View SolutionShow that the height of the right circular cone of maximum volume that can be inscribed in a sphere of radius 12 cm is 16 cm.
View SolutionShow that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is 2R√3 .
View SolutionShow that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is 2R√3 .
View SolutionShow that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is 2R√3
View SolutionShow that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is 2R√3 .
View SolutionThe altitude of a right circular cone of minimum volume circumscired about a sphere of radius r is
View SolutionThe height of a right circular cylinder of maxium volume inscribed in a sphere of radius 3 cm is
View SolutionShow that the height of the cylinder of maximum volume that can be inscribed in a cone of height h is 13h
View SolutionShow that the height of the right circular cylinder of maximum volume that can be inscribed in a given right circular cone of height h is h3
View Solution