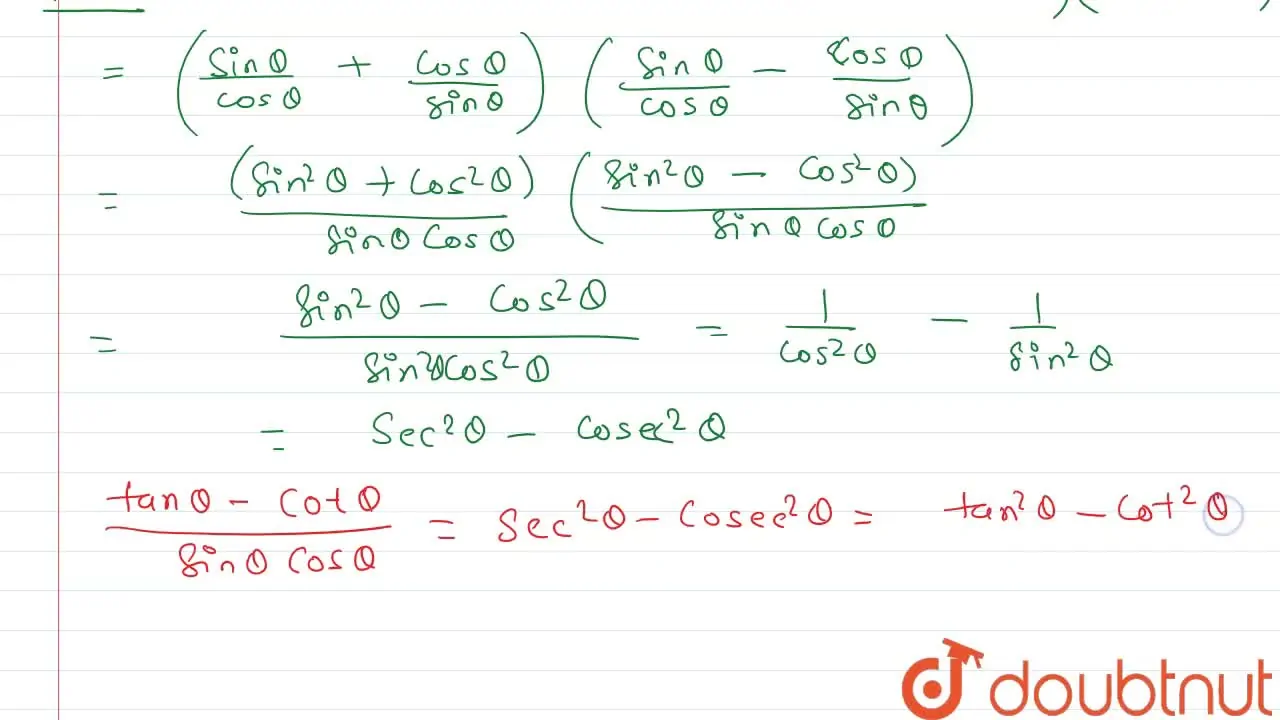
Prove the following identities: tanθ−cotθsinθcosθ=sec2θ−cosec2θ=tan2θ−cot2θ
Step by step video & image solution for Prove the following identities: (tantheta-cottheta)/(sinthetacostheta)=sec^2theta-cos e c^2theta=tan^2theta-cot^2theta by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
Similar Questions
Prove the following identity: (1+cotθ+tanθ)(sinθ−cosθ)sec3θ−cosec3θ=sin2θcos2θ
View SolutionProve the following identities: tan2θ+cot2θ+2=sec2θcosec2θ
View SolutionProve the following identity: 2sinθcosθ−cosθ1−sinθ+sin2θ−cos2θ=cotθ
View SolutionProve the following identities: (sinθ+cosecθ)2+(cosθ+secθ)2=7+tan2θ+cot2θ
View SolutionProve the following identities: (sinθ+cosecθ)2+(cosθ+secθ)2=7+tan2θ+cot2θ
View SolutionProve the following identities: cos2θ1−tanθ+sin3θsinθ−cosθ=1+sinθcosθ
View SolutionProve the following trigonometric identities: tanθ−cotθ=2sin2θ−1sinθcosθ
View SolutionProve the following trigonometric identities: tanθ−cotθ=2sin2θ−1sinθcosθ
View SolutionProve the following identities: √sec2θ+cosec2θ=tanθ+cotθ
View SolutionProve: cosec2θ+sec2θ=cosec2θsec2θ
View Solution