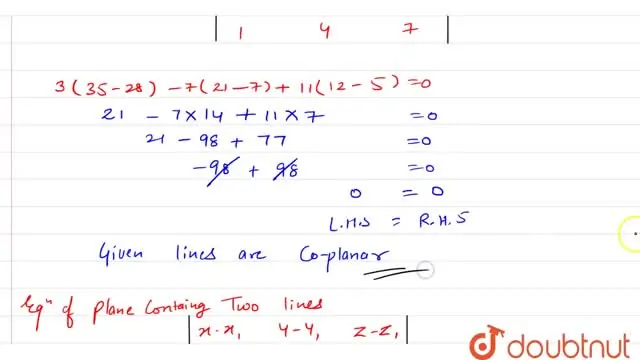
Prove that the lines x+13=y+35=z+57andx−21=y−44=z−67 are coplanar . Also, find the plane containing these two lines.
Direction ratio's of L1(3,5,7)andL2(1,4,7) and B(2−(−1),4−(−3),6−(−5))=(3,7,11)
So,
∣∣
∣∣3711357147∣∣
∣∣=3(35−28)−7(21−7)+11(12−5)=0
So, these given lines are coplanar.
Related Playlists
Similar Questions
Show that the lines x+3−3=y−11=z−55;x+1−1=y−22=z−55 are coplanar. Also find the equation of the plane containing the lines.
View SolutionShow that the lines x+1−3=y−32=z+21 and x1=y−7−3=z+72 are coplanar. Also, find the equation of the plane containing them.
View SolutionShow that the lines x+13=y+35=z+57 and x−21=y−43=z−65 intersect. Also find the their point of intersection.
View SolutionShow that the lines x−32=y+1−3=z+21andx−7−3=y1=z+72 are coplanar. Also find the equation of the plane containing them.
View SolutionIf the straight lines x−12=y+1k=z2andx+15=y+12=zk are coplanar, then the plane(s) containing these two lines is/are
View SolutionShow that lines x−25=y+11=z−2−6andx+3−8=y−2−1=z−87 are coplanar.
View SolutionThe lines x−21=y−31=z−4−kandx−1k=y−42=z−51 are coplanar, if
View SolutionShow that the lines x+3−3=y−1=z−55 and x+1−1=y−22=z−55 are coplanar. Also find their point of intersection.
View SolutionFind the equation of a plane passing through the parallel lines x−31=y+2−4=z5
and z−41=y−3−4=z−25 are coplanar. Also find the equation of plane in which these lines lie.View SolutionStatement 1: The lines x−11=y−1=z+11 and x−22=y+12=z3 are coplanar and the equation of the plnae containing them is 5x+2y−3z−8=0
Statement 2: The line x−21=y+12=z3 is perpendicular to the plane 3x+5y+9z−8=0 and parallel to the plane x+y−z=0View Solution