Prove that the curves x=y2and xy=k cut at right angles* if 8k2=1.
The equation of the given curves are given as x=y2 and xy=k
Putting x=y2 and xy=k we get,
y3=k⇒y=(k)13
x=k23 Thus, the point of intersection of the given curves as (k23,k13)
Differentiating x=y2 with respect to x, we have:
1=2ydydx⇒dydx=12y
Therefore, the slope of the tangent to the curve is x=y2 at (k23,k13)
dydx⎤⎥⎦k23,k13=1(2k)13
On differentiating xy=k with respect to x, we have:
xdydx+y=0⇒dydx=−yx
Slope of a tangent to the curve xy=k at (k23,k13)
dydx⎤⎦k23,k13=−yx⎤⎦k23,k13=−k13k23=−1k13
We know that two curves intersect at right angles if the tangents to the curve at the point of intersection at (k23,k13) are perpendicular to each other. This implies that we should have the product of the tangents as-1.Thus , the given two curves cut at right angles if the product of the slopes of their respective tangents at (k23,K13) is −1.
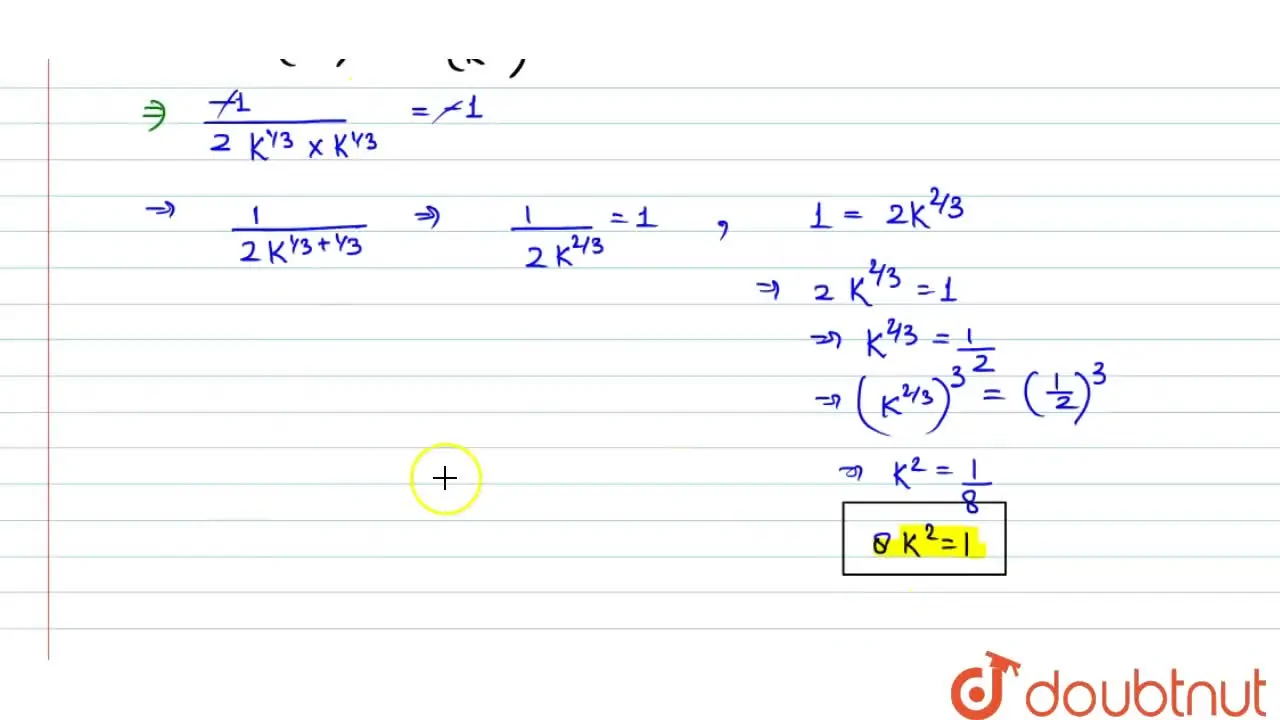
(12k13)(−1k13)=−1
⇒2k23=1
(2k23)3=(1)3
8k2=1
Hence the given two curves cut at right angles if 8k2=1
Related Playlists
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - MISCELLANEOUS EXERCISE23videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.49videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.118videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
Similar Questions
Show that the curves x=y2 and xy=k cut at right angles, if 8k2=1 .
View SolutionShow that the curves x=y2 and xy=k cut at right angles; if 8k2=1
View SolutionShow that the curves 4x=y2 and 4xy=k cut at right angles, if k2=512 .
View SolutionShow that the curves 2x=y2 and 2xy=k cut at right angles, if k2=8 .
View SolutionShow that the curves x=y2andxy=k cut at right angles, if 8k2=1
View SolutionProve that the curves x=y2 and x y=k intersect at right angles if 8k2=1.
View SolutionShow that the curves 2x=y2and2xy=k cut at right angles, if k2=8.
View SolutionShow that the curves 2x=y2 and 2xy=k cut each other at right angles if k2=8
View SolutionProve that the curves y2=4axandxy=c2 cut at right angles if c4=32a4.
View SolutionProve that the curves x=y2and xy=k cut at right angles* if 8k2=1.
View Solution