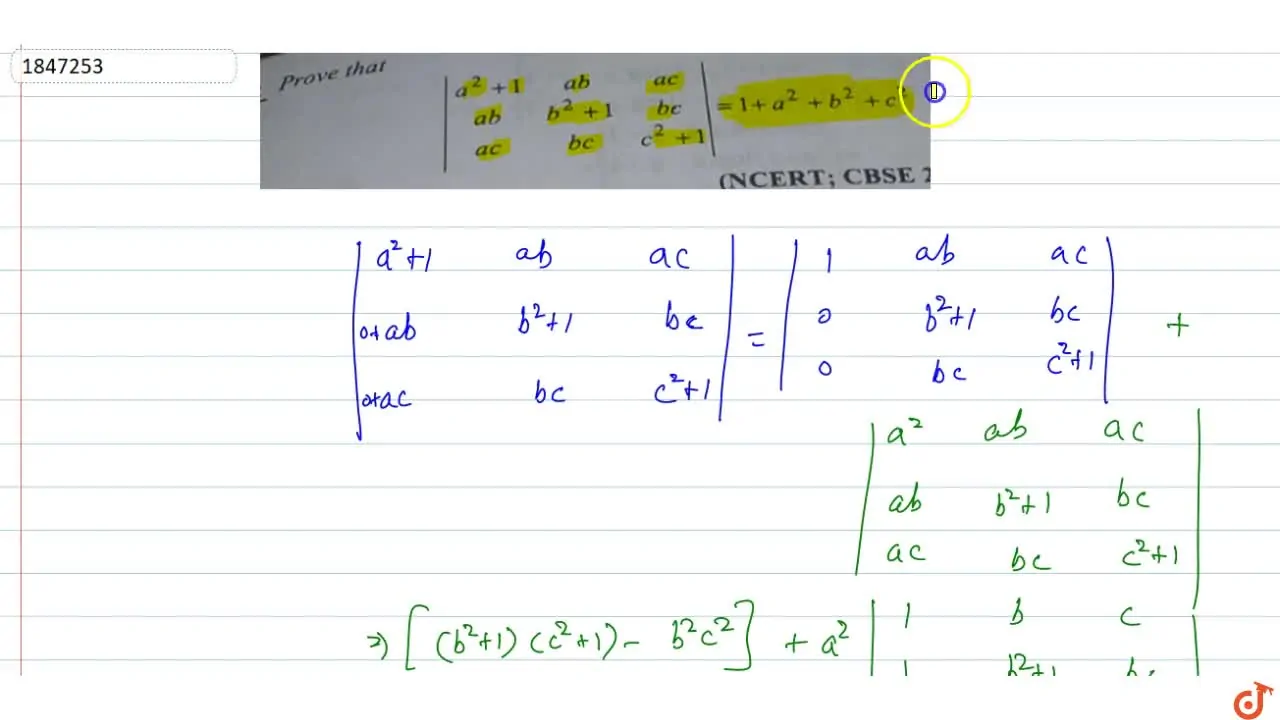
Prove that ∣∣ ∣ ∣∣a2+1abacabb2+1bcacbcc2+1∣∣ ∣ ∣∣=1+a2+b2+c2
Video Solution
Text Solution
Let the given determinant be Δ. Then,
Δ=∣∣
∣
∣
∣
∣∣a(a+1a) ab ac ab(b+1b)b bc ca cb(c+12)c∣∣
∣
∣
∣
∣∣
=(abc)⋅∣∣
∣
∣
∣∣a+1a a a bb+1b b c cc+1c∣∣
∣
∣
∣∣[takinga,b,ccommon fromC1,C2,C3respectively]
=(abc)(abc)⋅∣∣
∣
∣∣a2+1 a2 a2 b2b2+1 b2 c2 c2c2+1∣∣
∣
∣∣[applyingR1→aR1,R2→bR2,R3→cR3and dividing the whole det by abc]
=∣∣
∣
∣∣1+a2+b2+c21+a2+b2+c21+a2+b2+c2 b2b2+1 b2 c2 c2c2+1∣∣
∣
∣∣[byR1→R1+R2+R2+R3]
=(1+a2+b2+c2)⋅∣∣
∣
∣∣1 11b2b2+1b2c2 c2c2+1∣∣
∣
∣∣[taking out(1+a2+b2+c2)common fromR1]
=(1+a2+b2+c2)⋅∣∣
∣
∣∣100b210c201∣∣
∣
∣∣[byC2→C2−C1 and C3→C3−C1]
=(1+a2+b2+c2)⋅1⋅∣∣∣1001∣∣∣[expanded byR1]
=(1+a2+b2+c2)⋅1⋅(1−0)=(1+a2+b2+c2)
Hence, Δ=(1+a2+b2+c2)
|
Updated on:21/07/2023
Related Playlists
- BOOK - RS AGGARWALCHAPTER - DETERMINANTSEXERCISE - Exercise 6A22videos
DETERMINANTS
- BOOK - RS AGGARWALCHAPTER - DETERMINANTSEXERCISE - Exercise 6B44videos
DETERMINANTS
- BOOK - RS AGGARWALCHAPTER - DEFINITE INTEGRALSEXERCISE - Objective Questions73videos
DEFINITE INTEGRALS
- BOOK - RS AGGARWALCHAPTER - DIFFERENTIAL EQUATIONS AND THEIR FORMATION EXERCISE - Exercise 18C16videos
DIFFERENTIAL EQUATIONS AND THEIR FORMATION
Similar Questions
∣∣ ∣ ∣∣x2+a2abacabx2+b2bcacbcx2+c2∣∣ ∣ ∣∣=
View Solutionif ⎡⎢⎣−a2abacab−b2bcacbc−c2⎤⎥⎦=λa2b2c2 then find the value of λ
View SolutionIf ∣∣ ∣ ∣∣−a2abacab−b2bcacbc−c2∣∣ ∣ ∣∣=ka2b2c2 , then k =
View SolutionProve that ∣∣ ∣ ∣∣111abca2−bcb2−cac2−ab∣∣ ∣ ∣∣=0
View SolutionIf A=⎡⎢⎣a2abacabb2bcacbcc2⎤⎥⎦ and a2+b2+c2=1, then A2
View SolutionUsing properties of determinants, prove that ∣∣ ∣ ∣∣a2bcac+c2a2+abb2acabb2+bcc2∣∣ ∣ ∣∣ = 4a2b2c2
View SolutionProve that ∣∣ ∣ ∣∣1aa2−bc1bb2−ca1cc2−ab∣∣ ∣ ∣∣=0
View SolutionProve that ∣∣ ∣∣1abc1bca1cab∣∣ ∣∣=∣∣ ∣ ∣∣1aa21bb21cc2∣∣ ∣ ∣∣
View Solutionthe determinant Δ=∣∣ ∣ ∣∣a2+xabacabb2+xbcacbcc2+x∣∣ ∣ ∣∣ is divisible by
View SolutionIf ∣∣ ∣ ∣∣−a2abacab−b2bcacbc−c2∣∣ ∣ ∣∣=λa2b2c2, then λ equals
View Solution