Prove that a necessary and sufficient condition for three vectors →a,→b and →c to be coplanar is that there exist scalars l,m,n not all zero simultaneously such that l→a+m→b+n→c=→0.
Here, it is given that ,
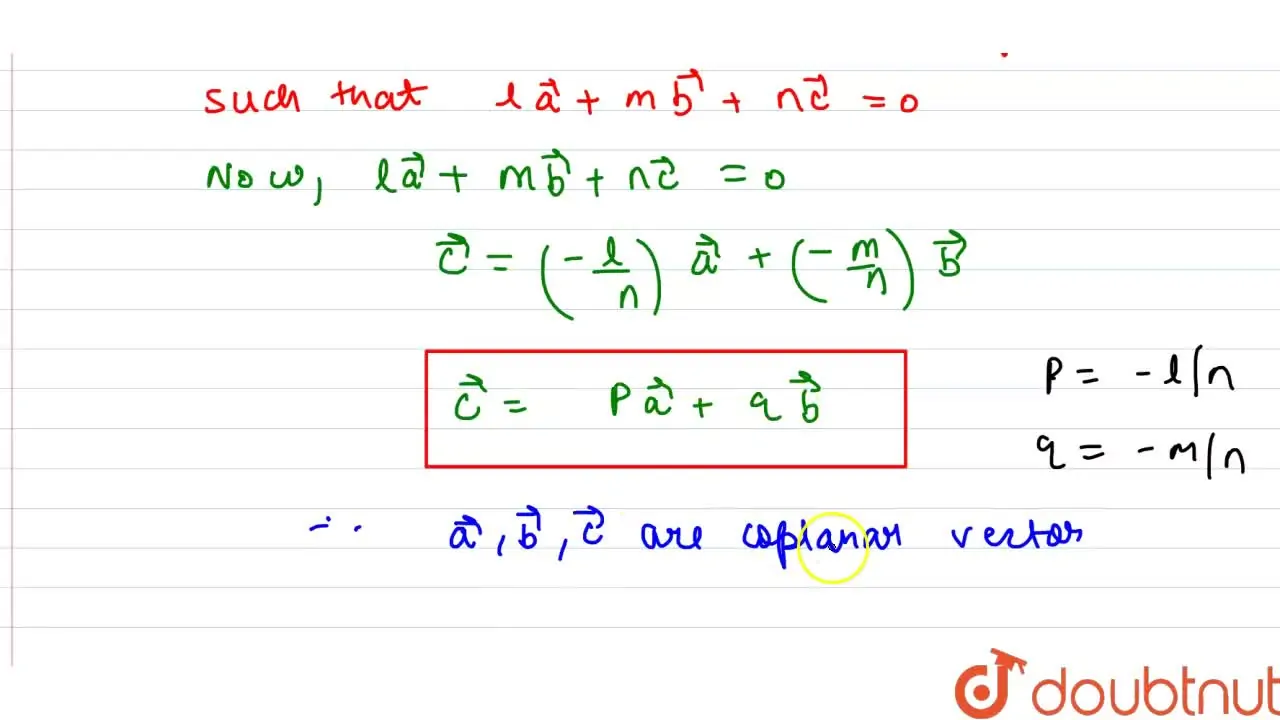
l→a+m→b+n→c=0
If we put, l=−1,m=xandn=y, then,
−→a+x→b+y→c=0
⇒→a=x→b+y→c, which is the neccessary condition for all three vectors to be coplanar.
Now,
l→a+m→b+n→c=0
⇒l→a=−m→b−n→c
⇒→a=−ml→b−nl→c, which is the sufficient condition for all three vectors to be coplanar.
Related Playlists
Similar Questions
Prove that a necessary and sufficient condition for three vectors →a,→b and →c to be coplanar is that there exist scalars l,m,n not all zero simultaneously such that l→a+m→b+n→c=→0.
View SolutionThe vectors →a,→b,→c,→d are coplanar then
View SolutionShow that the vectors →a, →b and →c are coplanar if →a+→b, →b+→c and →c+→a are coplanar.
View SolutionShow that the vectors →a,→b and→c are coplanar if →a+→b,→b+→c and →c+→a are coplanar.
View SolutionIf →a×→b=→b×→c≠0, where →a,→band→c are coplanar vectors, then for some scalar k
View SolutionIf vectors →a,→b,and→c are coplanar, show that ∣∣∣→a→b→c→a.→a→a.→b→a.→c→b.→a→b.→b→b.→c∣∣∣=⊙
View SolutionIf the three vectors →a, →b , →c are coplanar, prove that the vectors →a+→b,→b+→c and →c+→a are also coplanar.
View SolutionShow that vectors →a, →b, →c are coplanar if →a+→b, →b+→c, →c+→a are coplanar.
View SolutionFor any three vectors →a,→b,→c find [→a+→b→b+→c→c+→a]
View SolutionIf the vectors →a,→b,→c are coplanar, then the value of ∣∣ ∣ ∣∣→a→b→c→a⋅→a→a⋅→b→a⋅→c→b⋅→a→b⋅→b→b⋅→c∣∣ ∣ ∣∣=
View Solution