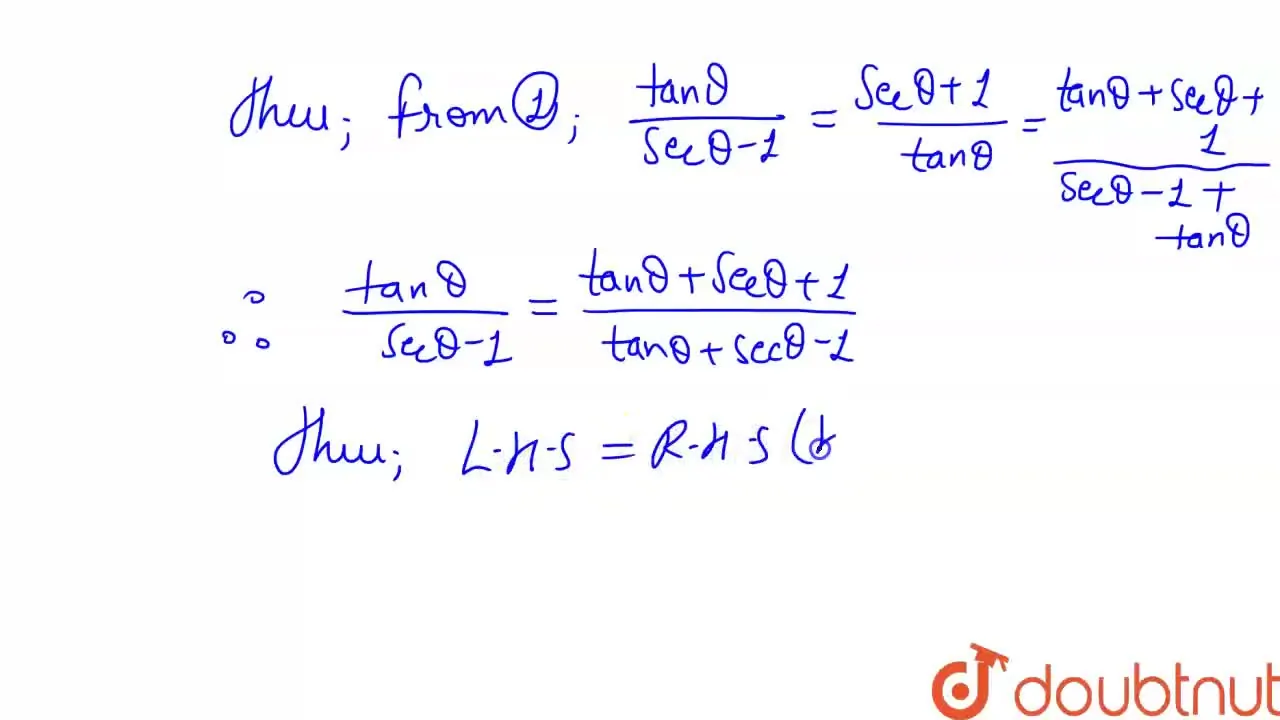
Prove: tanθsecθ−1=tanθ+secθ+1tanθ+secθ−1
Video Solution
Text Solution
Proof: 1+tan2θ=sec2θ …(Identity)
∴tan2θ=sec2θ−1
∴tanθ×taθ=(secθ+1)(secθ−1)
∴tanθ(secθ−1)=(secθ+1)tanθ
By theorem on equal ratios,
tanθ(secθ−1)=(secθ+1)tanθ=tanθ+secθ+1secθ−1+tanθ
∴tanθsecθ−1=tanθ+secθ+1tanθ+secθ−1
|
Updated on:21/07/2023
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIGONOMETRYEXERCISE - Assignment 7.17videos
TRIGONOMETRY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIGONOMETRYEXERCISE - Assignment 7.25videos
TRIGONOMETRY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIGONOMETRYEXERCISE - CHALLENGING QUESTIONS6videos
TRIGONOMETRY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THEOREMS EXERCISE - CIRCLE15videos
THEOREMS
Similar Questions
tan2θ(sec8θ−1)=tan8θ(sec4θ−1)
View Solutiontanθ+secθ−1tanθ−secθ+1=1+sinθcosθ
View SolutionProve that :
tanθsecθ+1=secθ−1tanθView Solution(tanθ+secθ−1)(tanθ+1+secθ)=2sinθ1−sinθ
View Solutiontanθsecθ−1+tanθsecθ+1=2cosec θ
View SolutionProve : secθ−1tanθ=tanθsecθ+1
View Solutiontanθsecθ+1+secθ+1tanθ=2cosecθ
View SolutionProve the following identities: tanθ+secθ−1tanθ−secθ+1=1+sinθcosθ
View Solutiontanθ+1tanθ=secθcosecθ
View SolutionProve that :
tanθsecθ−1+tanθsecθ+1=2cosecθView Solution