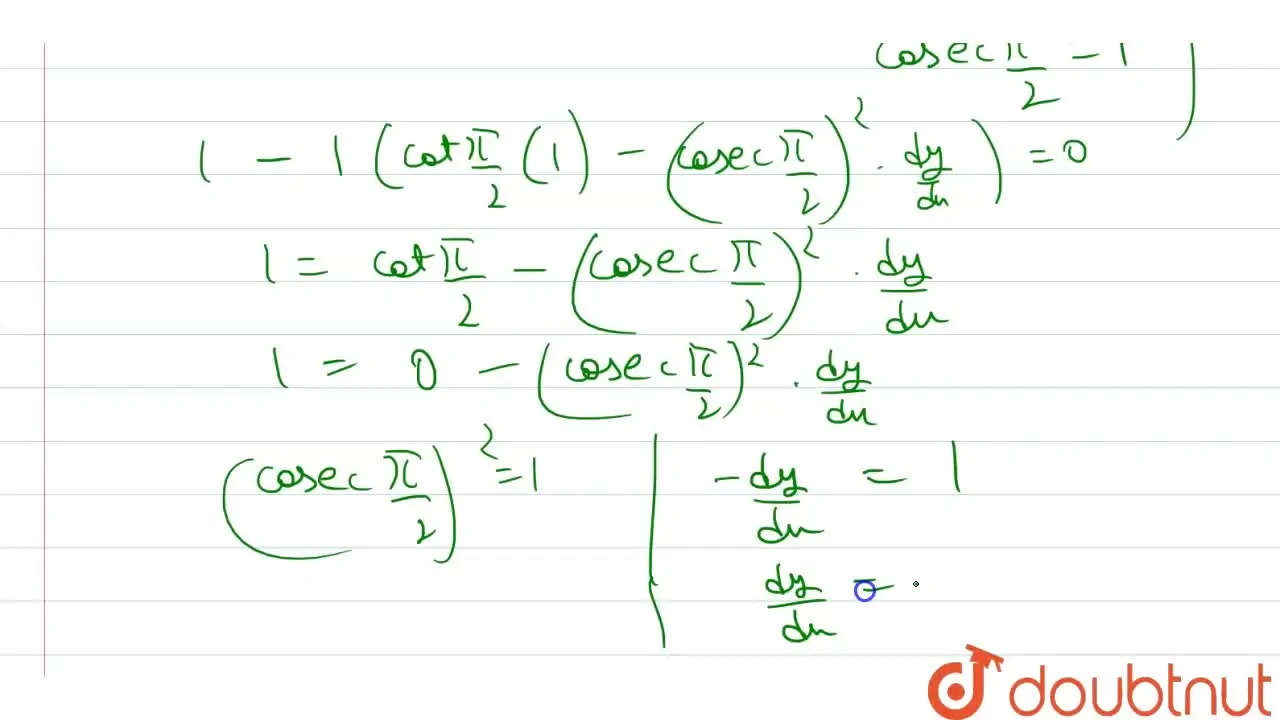
Let y be an implicit function of x defined by x2x−2xxcoty−1=0. Then y' (1) equals
-1
1
log 2
-log 2
x2x−2xxcoty−1=0 (i)
Now at x=1,
1−2coty−1=0⇒coty=0⇒y=π2
Now differentiating (i) w.r.t. x, we get
2x2x(1+logx)−2[xx(−cosec2y)dydx+cotx2(1+logx)]=0
Now at (1,π/2),
2(1+log1)−2[1(−1)(dydx)(1π/2)+0]=0
⇒ 2+2(dydx)(1π/2)=0
(dydx)(1π/2)=−1
Related Playlists
Similar Questions
Let y be an implicit function of x defined by x2x−2xxcoty−1=0. Then y'(1) equals: 1 b. log2 c. −log2 d. −1
View SolutionLet y=f(x) be a real valued function satisfying xdydx=x2+y−2, f(1)=1 then f(3) equal
View SolutionLet a function of 2 variables be defined by g(x,y)=xy+3xy2−(x−y2), what is the value of g(2,−1)?
View SolutionLet a function of 2 variables be defined by h(x,y)=x2+3xy−(y−x), what is the value of h(5,4)?
View SolutionLet ∅ be an operation on x and y defined as x∅y=x−2+y−2x−1+y−1 . Find the value of (1∅1)∅3 ?
View SolutionIf a function y=f(x) is defined as y=1t2−t−6andt=1x−2,t∈R. Then f(x) is discontinuous at
View SolutionWrite explicit functions of y defined by the following equations and also find the domains of definitions of the given implicit functions: x+|y|=2y (b) ey−e−y=2x (c)10x+10y=10 (d) x2−sin−1y=π2
View SolutionLet f(x, y) be a periodic function satisfying f(x,y)=f(2x+2y,2y−2x) for all x, y; Define g(x)=f(2x,0). Show that g(x) is a periodic function with period 12.
View SolutionIf y(x) is the solution of the differential equation dydx=−2x(y−1) with y(0)=1, then limx→∞y(x) equals
View SolutionIf y(x) is the solution of the differential equation dydx=−2x(y−1) with y(0)=1, then limx→∞y(x) equals
View Solution