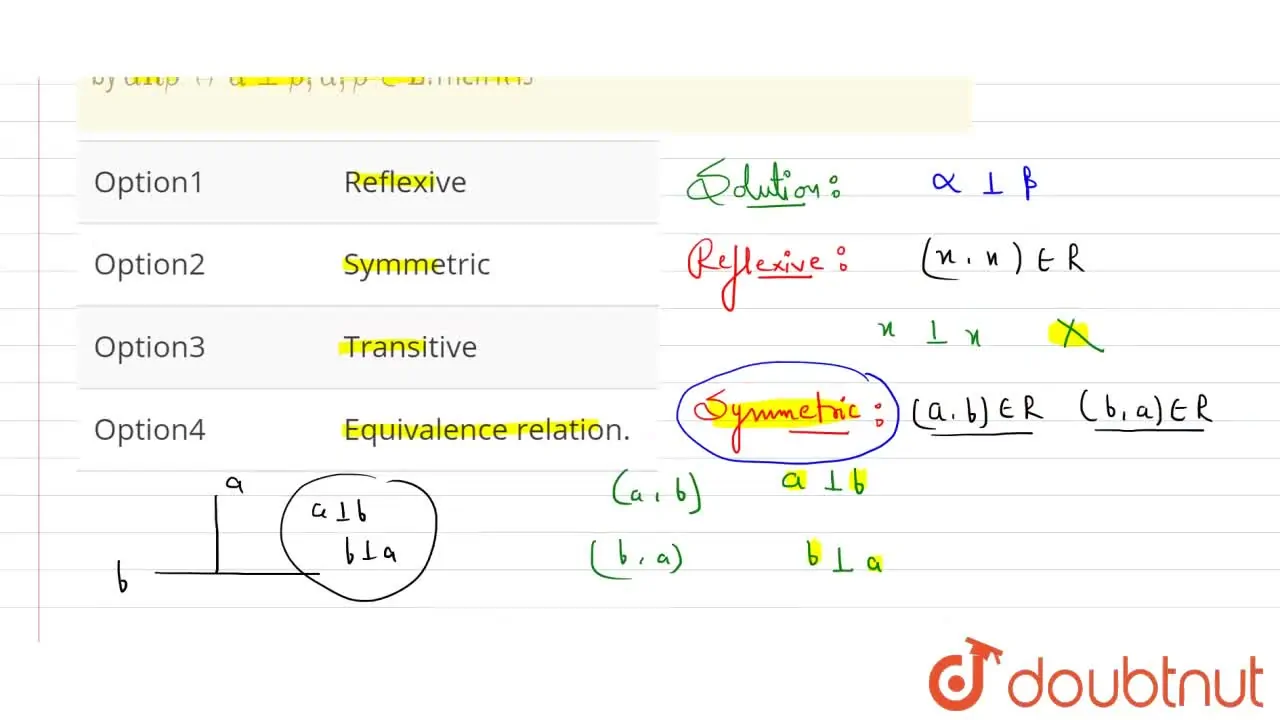
Let L denote the set of all straight lines in a plane.Let a relation R be defined by αRβ⇔α⊥β,α,β∈L.Then R is
Reflexive
Symmetric
Transitive
Equivalence relation.
The correct Answer is:B
NA
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - MATCH THE COLUMN2videos
RELATION, FUNCTION & ITF
- BOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - INTEGER_TYPE21videos
RELATION, FUNCTION & ITF
- BOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - SUBJECTIVE_TYPE179videos
RELATION, FUNCTION & ITF
- BOOK - RESONANCE ENGLISHCHAPTER - NUMBER THEORYEXERCISE - Exercise -2 (PART - II)4videos
NUMBER THEORY
- BOOK - RESONANCE ENGLISHCHAPTER - SEQUENCE & SERIES EXERCISE - EXERCISE -2 (PART-II : PREVIOUSLY ASKED QUESTION OF RMO)3videos
SEQUENCE & SERIES
Similar Questions
Let L denote the set of all straight lines in a plane. Let a relation R be defined by a R b⇔a⊥b,∀a,b∈L. Then, R is
View SolutionLet L denote the set of all straight lines in a plane. Let a relation R be defined by l R m iff l is perpendicular to m for all, l, m∈L . Then, R is (a) reflexive (b) symmetric (c) transitive (d) none of these
View SolutionLet L be the set of all lines in a plane and let R be a relation defined onL by the rule (x,y)εR→x is perpendicular to y. Then prove that R is a symmetric relation on L.
View SolutionIf R is a relation on the set of all straight lines drawn in a plane defined by l1 R l2 iff l1⊥l2, then R is
View SolutionLet L be the set of all lines in XY -plane and R be the relation in L defined as R={(L1,L2):L1 is parallel to L2} . Show that R is an equivalence relation. Find the set of all lines related to the line y=2x+4 .
View SolutionLet L be the set of all lines in a plane and R be the relation in L defined as R={(L1,L2):L1(is perpendicular to L2} Show that R is symmetric but neither reflexive nor transitive.
View SolutionLet R be a relation on the set of all lines in a plane defined by (l1, l2)∈R <=> line l1 is parallel to line l2 . Show that R is an equivalence relation.
View SolutionLet L be the set of all lines in XY=plane and R be the relation in L defined as R={(L1,L2):L1 is parallel to L2}. Show that R is an equivalence relation. Find the set of all lines related to the line y=2x+4.
View SolutionLet L be the set of all lines in XY=plane and R be the relation in L defined as R={(L1,L2):L1 is parallel to L2}. Show that R is an equivalence relation. Find the set of all lines related to the line y=2x+4.
View SolutionLet L be the set of all straight lines in plane. l1 and l2 are two lines in the set. R1,R2 and R3 are defined relations.
(i) l1R1l2:l1 is parallel to l2
(ii) l1R2l2:l1 is perpendicular to l2
(iii) l1R3l2:l1 intersects l2
Then which of the following is true ?View Solution