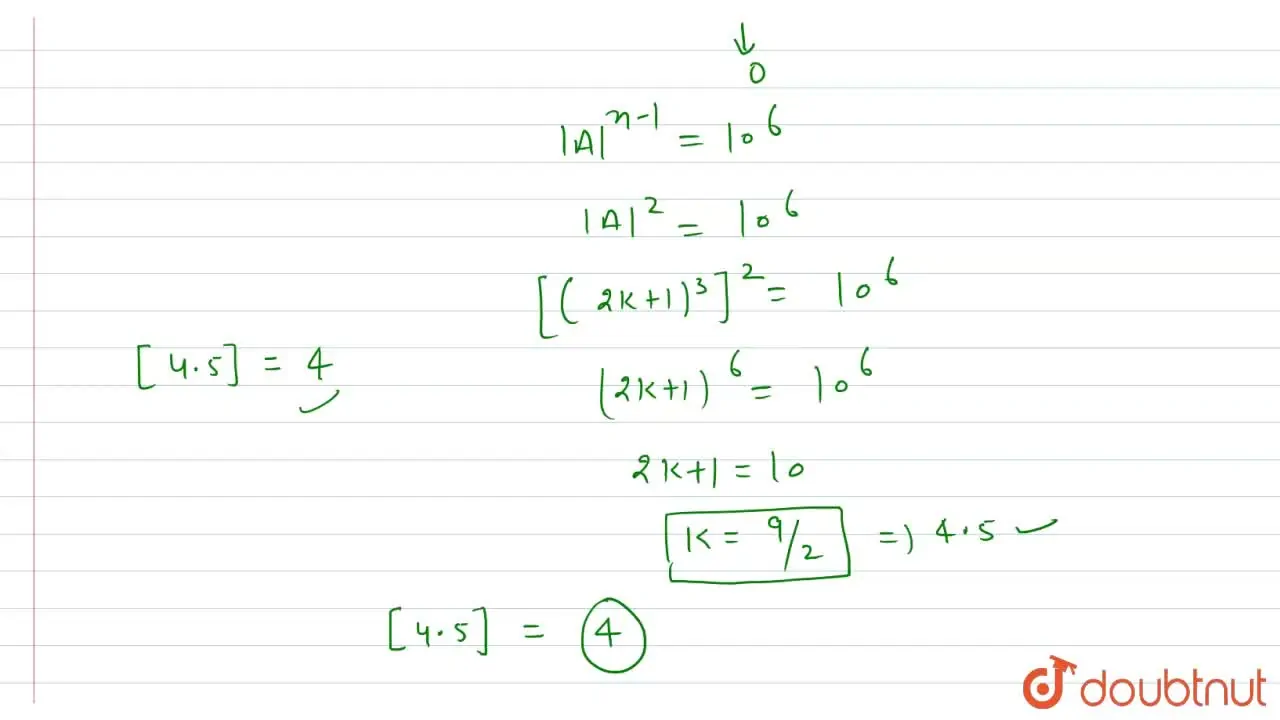Let K be a positive real number and
A=⎡⎢
⎢⎣2k−12√k2√k2√k1−2k−2√k2k−1⎤⎥
⎥⎦ and ⎡⎢
⎢⎣02k−1√k1−2k02−√k−2√k0⎤⎥
⎥⎦
If det (adjA)+det(adjB)=106 , then [k] is equal to
The correct Answer is:D
Step by step video, text & image solution for Let K be a positive real number and A=[(2k-1, 2sqrtk, 2sqrtk),(2sqrtk, 1,-2k),(-2sqrtk,2k,-1)] and [(0,2k-1, sqrtk),(1-2k, 0,2),(-sqrtk, -2sqrtk,0)] If det (adjA)+det(adjB)=10^6 , then [k] is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Let k be a positive real number and let
A=⎡⎢ ⎢⎣2k−12√k2√k2√k1−2k−2√k2k−1⎤⎥ ⎥⎦
b=⎡⎢ ⎢⎣02k−1√k1−2k00√k−√k−2√k0⎤⎥ ⎥⎦
If det(Adj(A))+det(Adj(B))=106 then [k] is equal toView SolutionLet K be a positive real number and A=[2k−12√k2√k2√k1−2k−2√k2k−1]andB=[02k−1√k1−2k02−√k−2√k0] . If det (adjA)+det(adjB)=106,then[k] is equal to. [Note: adjM denotes the adjoint of a square matix M and [k] denotes the largest integer less than or equal to K ].
View SolutionLet S=∑k=0∞2k72^k+1 then 1S is equal to
View SolutionLet A=⎡⎢⎣2070101−21⎤⎥⎦andB=⎡⎢⎣−k14k7k010k−4k−2k⎤⎥⎦. If AB=I, where I is an identity matrix of order 3, then the sum of all elements of matrix B is equal to
View SolutionIf A=[1221] and A(adjA)=k, then the value of k is
View SolutionIf k is positive and k4−47k2+1=0
View SolutionFor what value of k are the points (k,2−2k)(−k+1,2k)and(−4−k,6,6−2k) are collinear?
View Solution∞∑k=11k√k+2+(k+2)√k=2+√2a then value of a is
View SolutionSolve (k−2)(2k+3)(k−1)>0
View SolutionA=⎡⎢⎣10k213k01⎤⎥⎦ is invertible for
View Solution