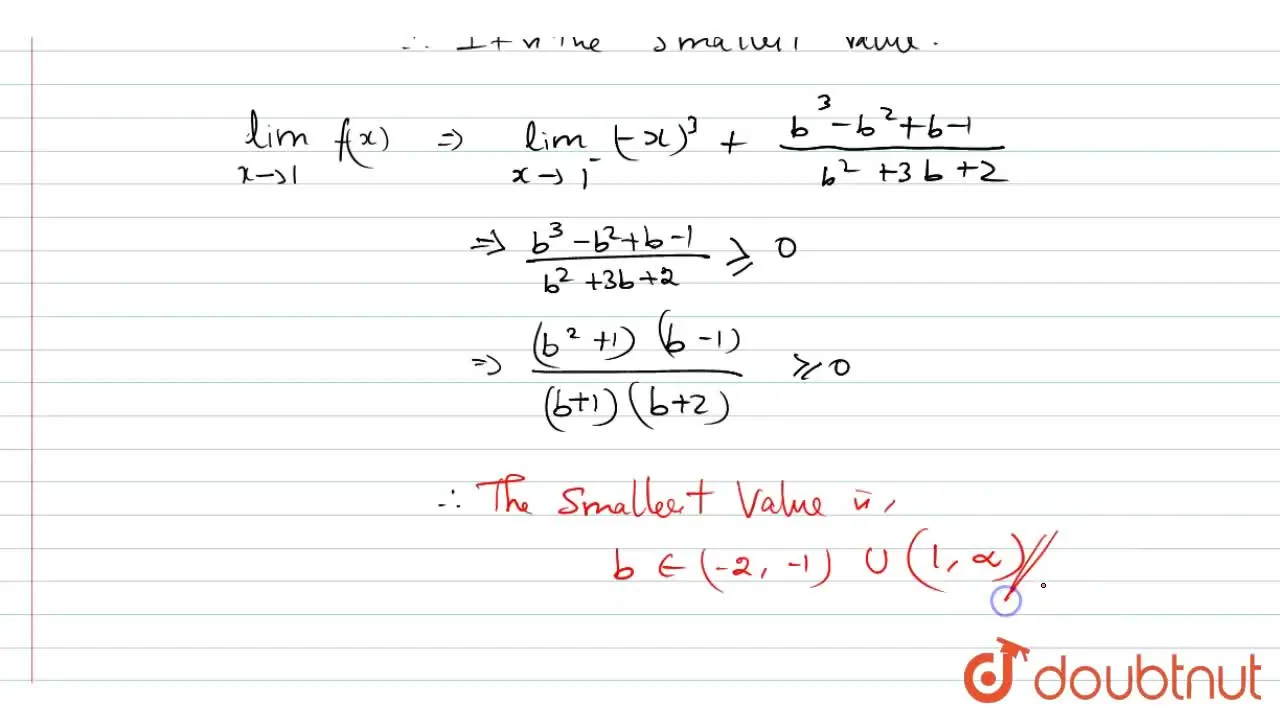
let f(x)=−x3+b3−b2+b−1b2+3b+2 if x is 0 to 1 and f(x)=2x−3 if x if 1 to 3.All possible real values of b such that f(x) has the smallest value at x=1 are
Step by step video & image solution for let f(x)=-x^3+(b^3-b^2+b-1)/(b^2+3b+2) if x is 0 to 1 and f(x)=2x-3 if x if 1 to 3.All possible real values of b such that f (x) has the smallest value at x=1 are by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Let f(x)={3x2−2x+10x<1−2x>1
The set of values of b for which f(x) has greatest value at x=1 isView SolutionLet f(x) ={3−x 0≤x<1x2+xb x≥1. Find the set of values of b such that f(x) has a local minima at x=1.
View SolutionLet f(x)={x3−x2+10x−5,x≤1, −2x+(log)2(b2−2),x>1 Find the values of b for which f(x) has the greatest value at x=1.
View SolutionIf f(x)={3ax+bifx>1,11ifx=1,5ax−2b,ifx<1.Determinethevaluesofaandbso that f(x) is continuous.
View SolutionLet f(x)=x3−3x+b and g(x)=x2+bx−3 where b is a real number. What is the sum of all possible values of b for which the equations f(x)= 0 and g(x) = 0 have a common root ?
View SolutionLet f(x)=⎧⎪⎨⎪⎩x3−x2+10x−5,x≤1−2x+log2(b2−2),x>1. The set of values of b for which f(x) has greatest value at x= 1 is given by .
View SolutionIf f(x)=x3+x2−ax+b is divisible by x2−x write the values of a and b .
View SolutionLet f(x)={1−sin3x3cos2xifx<π2,aifx=π2 . If f(x) is continuous at x=π2 b(1-sinx)/(pi-2x)^2, if x>pi/2 find a and b. .
View SolutionLet f(x)=2x3+3(1−3a)x2+6(a2−a)x+b where a,b∈R. Find the smallest integral value of a for which f(x) has a positive point of local maximum.
View SolutionIf 2x+3(x+1)(x+2)=Ax+1+Bx+2 for all real values of x(≠−1,−2) , what is the value of (A+B)?
View Solution