Let f be a function defined on [a, b] such that f′(x)>0, for all x∈(a,b). Then prove that f is an increasing function on (a, b).
We have to prove that the function is always increasing i.e.
f(x1)<f(x2) for x1<x2 where x1,x2∈[a,b]
PROOF
Let x1,x2be 2 numbers in the interval [a,b] i.e. x1,x2∈[a,b]
And,x1<x2
In the interval [x1,x2]
As f is defined everywhere, f is continuous and differentiable in [x1,x2]
By mean value theorem, there exists c in(x1,x2) i.e c∈(x1,x2) such that
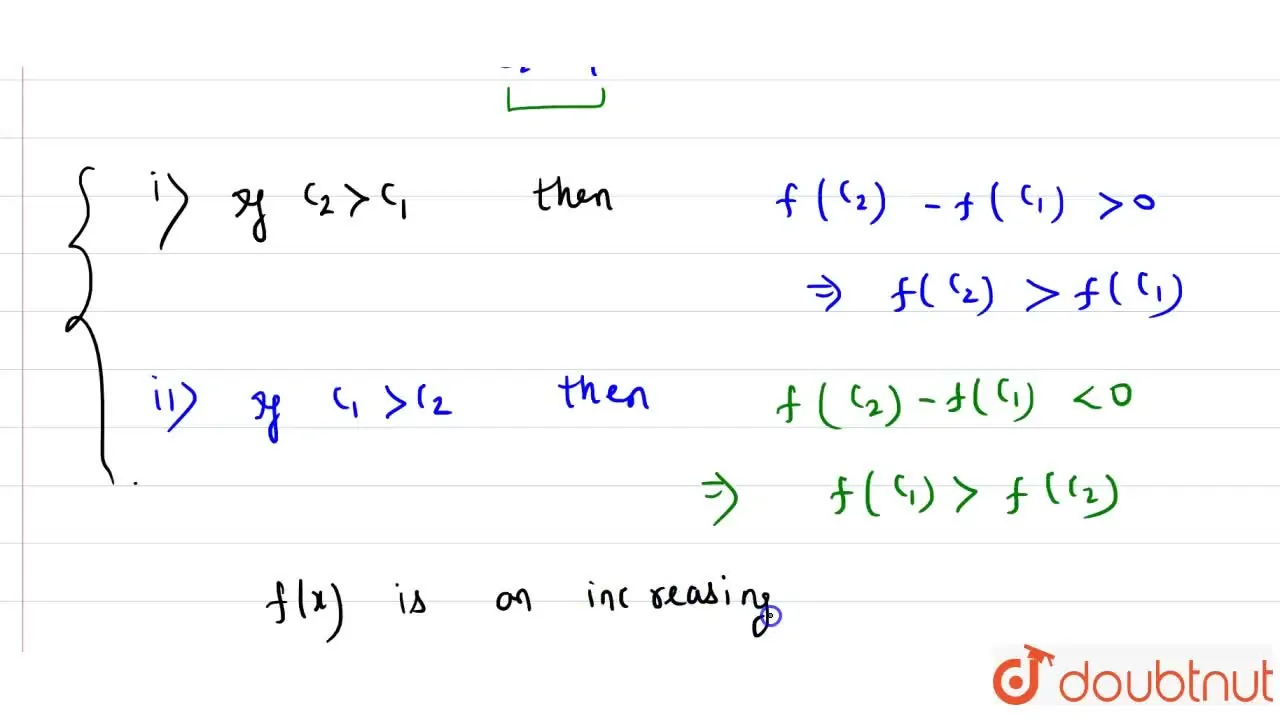
f'(c)=f(x2)−f(x1)x2−x1
So,f'(c)>0 for all c∈(x1,x2)
f(x2)−f(x1)x2−x1>0
f(x2)−f(x1)>0
So, we can say that for any 2 points x1,x2 in interval [a,b]
Where x2>x1
∴f(x2)>f(x1)
Thus, f is an increasing function in the interval [a,b]
Hence Proved.
Related Playlists
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.49videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.529videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.327videos
APPLICATION OF DERIVATIVES
- BOOK - NCERTCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
Similar Questions
Let f be a function defined on [a, b] such that f′(x)>0, for all x∈(a,b). Then prove that f is an increasing function on (a, b).
View SolutionLet f be a continuous function [a,b] such that f(x)>0 for all x∈[a,b]. If F(x)=∫xaf(t)dt then
View SolutionLet f be a differentiable function defined on R such that f(0) =−3. If f'(x) ≤5 for all x then
View SolutionLet f be a differentiable real function defined on (a;b) and if f'(x)>0 for all x∈(a;b) then f is increasing on (a;b) and if f'(x)<0 for all x∈(a;b) then f(x) is decreasing on (a;b)
View SolutionLet f be a function defined for every x, such that f''=−f,f(0)=0,f'(0)=1 then f(x) is equal to
View SolutionLet f be a function defined for every x, such that f''=−f, f(0)=0,f'(0) =1 then f(x) is equal to
View SolutionProve that f(x)=ax+b , where a, b are constants and a>0 is an increasing function on R .
View SolutionProve that f(x)=ax+b, where a,b are constants and a>0 is an increasing function on R.
View SolutionLet f (x) be a conitnuous function defined on [0,a] such that f(a−x)=f(x)for allx∈[0,a]. If ∫a/20f(x)dx=α, then ∫a0f(x)dx is equal to
View SolutionLet f:R→R be a function defined b f(x)=cos(5x+2). Then,f is
View Solution