Question

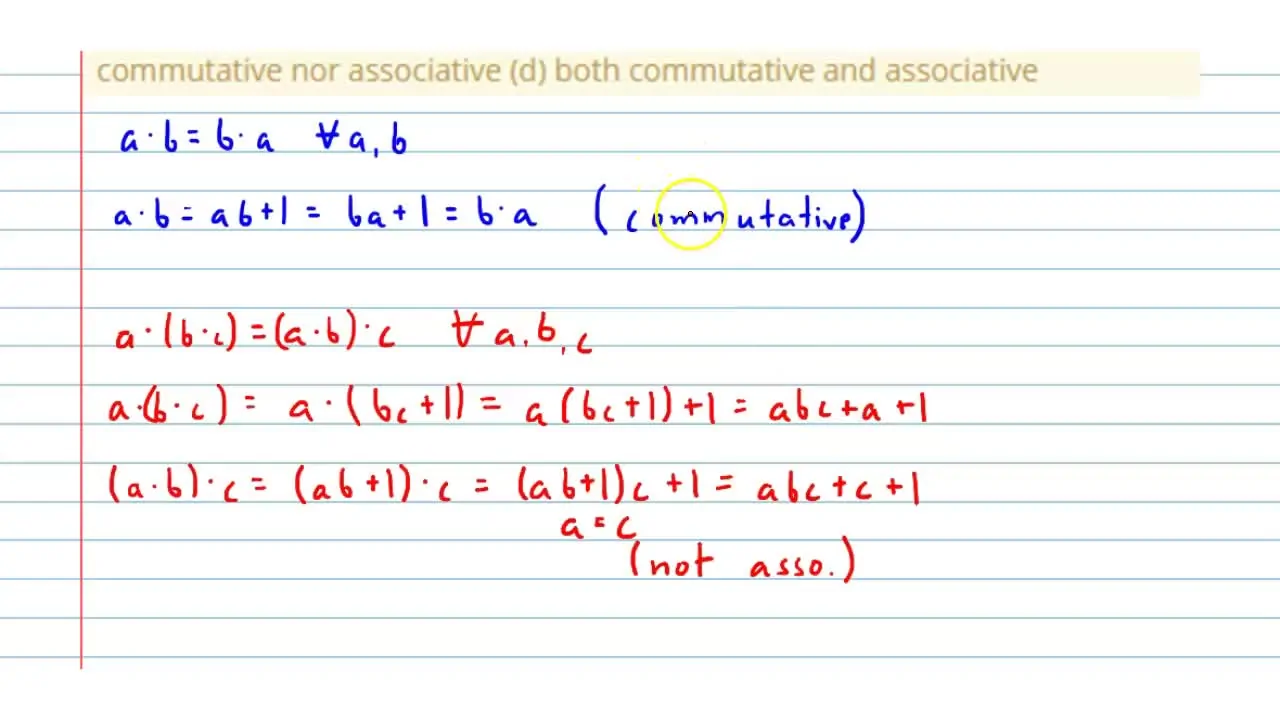
Let * be a binary
operation on ${\displaystyle R}$
defined by ${\displaystyle a\cdot b=ab+1}$
. Then, * is
commutative but not
associative
associative but not
commutative
neither commutative nor
associative
(d) both commutative
and associative

Answer

N/a

Explanation

To determine whether the binary operation defined by $$a\ast b=ab+1$$ is commutative and/or associative, we will analyze both properties step by step.

Step 1: Check for Commutativity
A binary operation is commutative if:
\( a * b = b * a \quad \text{for all } a, b \in \mathbb{R} \)

Calculation:
1. Calculate \( a * b \):
\( a * b = ab + 1 \)
2. Calculate \( b * a \):
\( b * a = ba + 1 \)
3. Since multiplication is commutative, \( ab = ba \):
\( b * a = ab + 1 \)
4. Thus, we have:
\( a * b = b * a \)

Conclusion:
The operation is commutative.

Step 2: Check for Associativity
A binary operation is associative if:
\( (a * b) * c = a * (b * c) \quad \text{for all } a, b, c \in \mathbb{R} \)

Calculation:
1. Calculate \( (a * b) * c \):
- First, find \( a * b \):
\( a * b = ab + 1 \)
- Now, compute \( (a * b) * c \):
\( (a * b) * c = (ab + 1) * c = (ab + 1)c + 1 = abc + c + 1 \)

2. Calculate \( a * (b * c) \):
- First, find \( b * c \):
\( b * c = bc + 1 \)
- Now, compute \( a * (b * c) \):
\( a * (b * c) = a * (bc + 1) = a(bc + 1) + 1 = abc + a + 1 \)

3. Now we compare \( (a * b) * c \) and \( a * (b * c) \):
\( (a * b) * c = abc + c + 1 \)
\( a * (b * c) = abc + a + 1 \)

Conclusion:
Since \( abc + c + 1 \neq abc + a + 1 \) for all \( a, b, c \) (unless \( a = c \)), the operation is not associative.

Final Conclusion
The binary operation defined by \( a * b = ab + 1 \) is commutative but not associative.

---