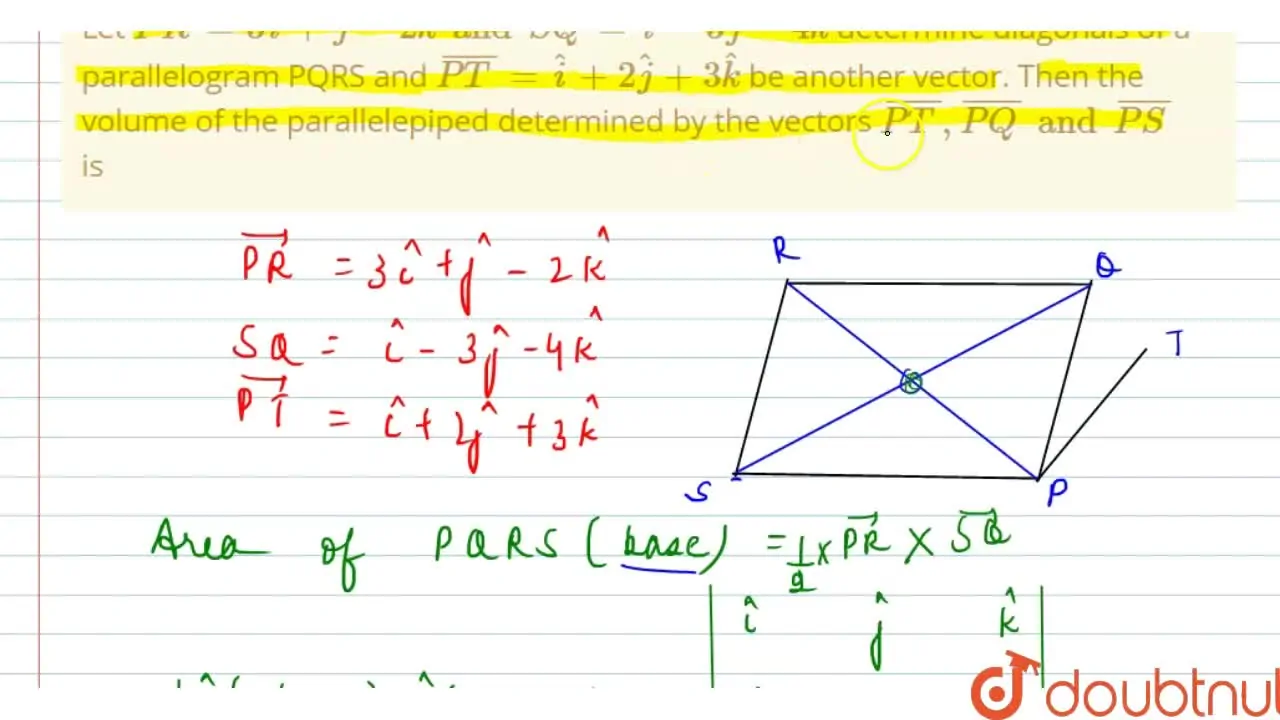
Let determine diagonals of a parallelogram PQRS and be another vector. Then the volume of the parallelepiped determined by the vectors is
5
20
10
30
The correct Answer is:c
Area of base (PQRS)
Height= projection of PT on
volume = = 10 cu. unit

Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise 2.318videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - All Questions1344videos
CONIC SECTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - LIMITS AND DERIVATIVESEXERCISE - All Questions691videos
LIMITS AND DERIVATIVES
Similar Questions
If the diagonals of a parallelogram are 3ˆi+ˆj−2ˆkandˆi−3ˆj+4ˆk, then the lengths of its sides are
View SolutionThe sides of a parallelogram are 2ˆi+4ˆj−5ˆkandˆi+2ˆj+3ˆk. The unit vector parallel to one of the diagonals is
View SolutionThe sides of a parallelogram are 2ˆi+4ˆj−5ˆkandˆi+2ˆj+3ˆk, then the unit vector parallel to one of the diagonals is
View SolutionFind the area oif the parallelogram determined A=2ˆi+ˆj−3ˆk and B=12ˆj−2ˆk
View SolutionThe acute angle that the vector 2ˆi−2ˆj+ˆk makes with the plane determined by the vectors 2ˆi+3ˆj−ˆk and ˆi−ˆj+2ˆk is
View SolutionArea of a parallelogram, whose diagonals are 3ˆi+ˆj−2ˆkandˆi−3ˆj+4ˆk will be:
View SolutionShow that the vectors ˆi−ˆj−ˆk,2ˆi+3ˆj+ˆkand7ˆi+3ˆj−4ˆk are coplanar.
View SolutionFind the area of the parallelogram having diagonals 2ˆi−ˆj+ˆkand3ˆi+3ˆj−ˆk
View SolutionThe resultant vector of of ¯¯¯¯¯¯OA=2ˆi+3ˆj+6ˆk and ¯¯¯¯¯¯OB=2ˆi−5ˆj+3ˆk is
View SolutionIf the diagonals of a parallelogram are represented by the vectors 3ˆi+ˆj−2ˆkandˆi+3ˆj−4ˆk, then its area in square units , is
View Solution