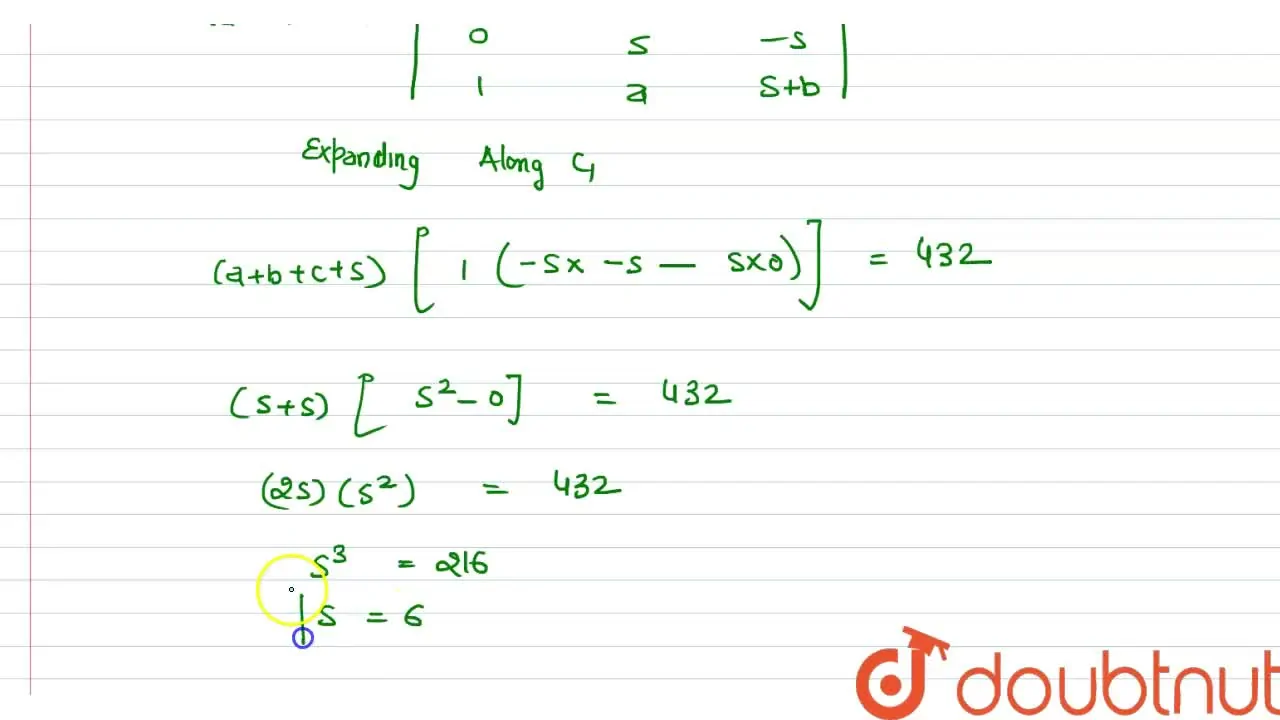
Let a+b+c =s and ∣∣ ∣∣s+cabcs+abcas+b∣∣ ∣∣=432 then the value of s is ____
The correct Answer is:6
Given that
∣∣
∣∣s+cabcs+abcas+b∣∣
∣∣=532
Applying C1→C1+C2+C3 we get
(a+b+c+s)∣∣
∣∣1ab1s+ab1as+b∣∣
∣∣=532
⇒2s∣∣
∣∣0−s00s−s1as+b∣∣
∣∣=532
⇒2s3=532
∴s3=216
s=6
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTEXERCISE - Multiple Correct Answer5videos
DETERMINANT
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
Similar Questions
If s=(a+b+c),then value of ∣∣ ∣∣s+cabcs+abcas+b∣∣ ∣∣is
View SolutionIf S=[abcd], then adj S is equal to
View SolutionWithout expanding show that, if s= a +b +c, then 2s is a factor of
∣∣ ∣∣s+cabcs+abcas+b∣∣ ∣∣View SolutionIf xy=a2andS=b2x+c2y where a,bandc are constants then the minimum value of S is
View SolutionIf the determinant ∣∣b−−aa−^(')−c'c′−a'a′−b'b−c''c−a''a−b''∣∣=m|abca'b'c'a''b''c''| , then the value of m s 0 b. 2 c. 1 d. −1
View SolutionIn a cyclic quadrilateral ABCD, prove that tan2B2=(s−a)(s−b)(s−c)(s−d),a,b,c,andd being the lengths of sides ABC, CD and DA respectively and s is semi-perimeter of quadrilateral.
View SolutionThe point P(a,b), Q(c,d), R(a,d) and S(c,b) , where a,b,c ,d are distinct real numbers, are
View SolutionLet A = {1, 2, 3}, B = {a, b, c}, C {a1,b1,c1,d1,e1} and consider the following statements.
S1 : The number of one-one functions from A to C is 60.
S2 : The number of onto functions from C to A is 150
S3 : The number of onto functions from B to C is 0
S4 : The number of objective functions from A to B is 6
Which of the following combination is true ?View SolutionIf the angles of ∆ABC are in ratio 1:1:2, respectively (the largest angle being angle C), then the value of s e c A c o s e c B secAcosecB - t a n A c o t B tanAcotB is (a) 0 (b) 1/2 (c) 1 (d) √3/2
View SolutionIf in a triangle ABC, right angled at B, s-a=3, s-c=2, then the values of a and c are respectively
View Solution