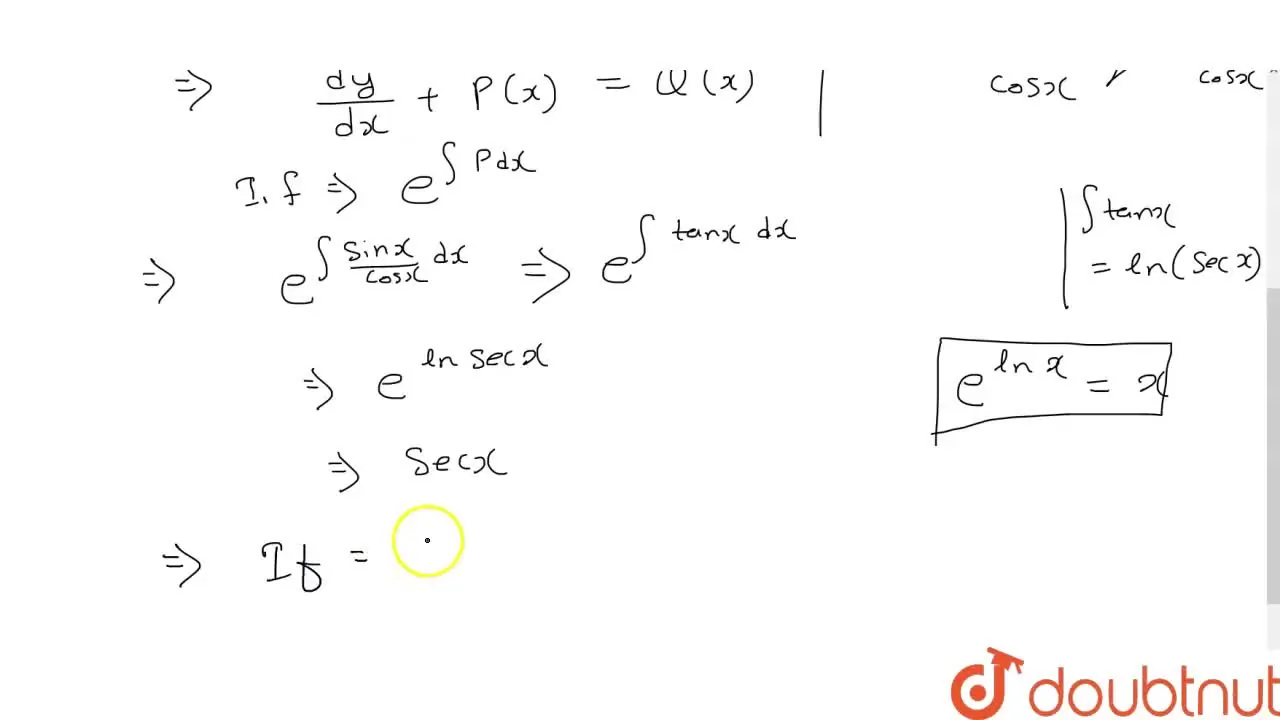
Integrating factor of differential equation cosxdydx+ysinx=1 is
Step by step video & image solution for Integrating factor of differential equation cosx(dy)/(dx)+ysinx=1 is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
The integrating factor of differential equation cosxdydx+ysinx=1 is
View SolutionThe integrating factor of differential equation dydx+y=1+yxis
View SolutionThe integrating factor of differential equation dydx+ytanx−secx=0 is
View SolutionThe integrating factor of differential euation (1−x2)dydx−xy=1 is
View SolutionIntegrating factor of the differential equation (x.logx)dydx+y=2logx is
View SolutionThe Integrating Factor of the differential equation xdydx−y=2x2is(A) e−x (B) e−y (C) 1x (D) x
View SolutionThe Integrating Factor of the differential equation xdydx−y=2x2is(A) e−x (B) e−y (C) 1x (D) x
View SolutionThe Integrating Factor of the differential equation xdydx−y=2x2 is
(A) e−x (B) e−y (C) 1x (D) xView SolutionThe integrating factor of the differential equation dydx+y=1+yx is
View SolutionIntegrating factor of the differential equation (x+1)dydx−y=e3x(x+1)2, is
View Solution