Integrate the function (x2+1)logx
Video Solution
Text Solution
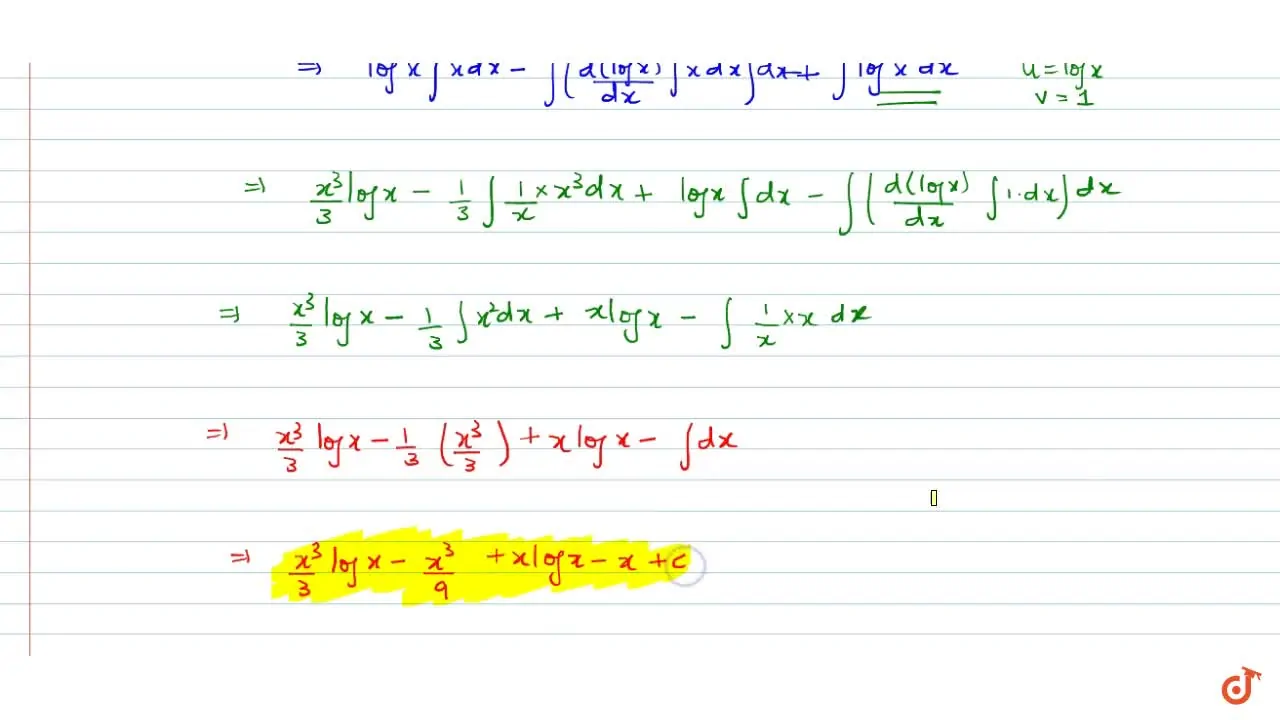
Let I=∫(x2+1)logxdx
=∫x2logxdx+∫logxdx
Let I=I1+I2...........(1)
Where, I1=∫x2logxdxandI2<br≥∫logxdx
From (1):−−−−
I1=∫x2logxdx
Solving by parts,
I1=logx∫x2dx−∫{dlogxdx∫x2dx}dx
=logx⋅x33−∫(13)⋅x33dx
=x33logx−(13)(∫x2dx)
=x33logx−x39+C1..........(2)
Again,
I2=∫logxdx
Taking logx as first function and 1 as second function
and integrating by part x, we obtain
I2=logx∫1⋅dx−∫{dlogxxd∫1⋅dx}
=logx⋅x−∫(1x)⋅xdx
=xlogx−∫1dx
=xlogx−x+C2......(3)
Using equation (2) and (3) in (1), we obtain
I=x33logx−x39+C1+xlogx−x+C2
=x33logx−x39+xlogx−x+(C1+C2)
=(x33+x)logx−x39−x+C
|
Updated on:21/07/2023
Related Playlists
- BOOK - NCERTCHAPTER - INTEGRALSEXERCISE - EXERCISE 7.1010videos
INTEGRALS
- BOOK - NCERTCHAPTER - INTEGRALSEXERCISE - EXERCISE 7.714videos
INTEGRALS
- BOOK - NCERTCHAPTER - INTEGRALSEXERCISE - EXERCISE 7.922videos
INTEGRALS
- BOOK - NCERTCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - EXERCISE 9.112videos
DIFFERENTIAL EQUATIONS
- BOOK - NCERTCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - MISCELLANEOUS EXERCISE17videos
INVERSE TRIGONOMETRIC FUNCTIONS
Similar Questions
Integrate the function (x2+1)logx
View SolutionIntegrate the functionsx21−x6
View SolutionIntegrate the functions(1+logx)2x
View SolutionIntegrate the function xcos−1x√1−x2
View SolutionIntegrate the functions1x2(x4+1)34
View SolutionIntegrate the functions(1+x)(x+logx)2x
View SolutionIntegrate the functionsx2+x+1(x+1)2(x+2)
View SolutionIntegrate the functions3x2x6+1
View SolutionIntegrate the functions1x+xlogx
View SolutionIntegrate the function xtan−1x
View Solution