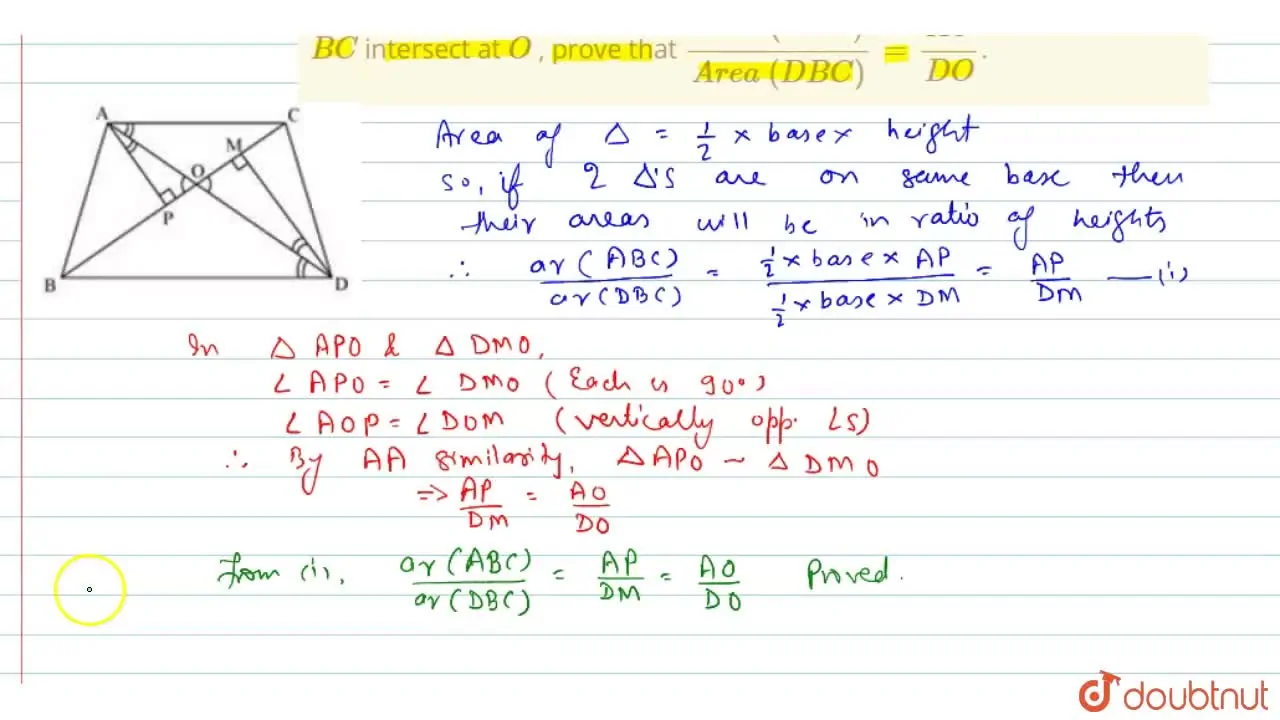
In Figure, △ABC and △DBC are on the same base BC . If AD and BC intersect at O , prove that Area (ABC)Area (DBC)=AODO.
Step by step video & image solution for In Figure, /_\ A B C and /_\ D B C are on the same base B C . If A D and B C intersect at O , prove that (A r e a\ ( A B C))/(A r e a\ ( D B C))=(A O)/(D O). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
Similar Questions
In triangle ABC , if AD is the bisector of ∠A , prove that: Area(ABD)Area(ACD)=ABAC
View SolutionIn figure ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)ar(DBC)=AODO.
 View Solution
View SolutionThe diagonals of quadrilateral ABCD intersect at O . Prove that ar(ACB)ar(ACD)=BODO
View SolutionIn ABC , AD and BE are altitudes. Prove that ar(DEC)ar(ABC)=DC2AC2
View SolutionIn Figure, ABC and ABD are two triangles on the base AB. If line segment CD is bisected by AB at O, show that ar (△ABC)=ar ( △ABD)
View SolutionIn Figure, if △ABE≅△ACD , prove that △ADE~△ABC .
View SolutionIn Figure, ABC and BDE are two equilateral triangls such that D is the mid-point of BC. If AE intersects BC in F, Prove that: ar(BDE)=14ar ( ABC).
View SolutionDiagonals AC and BD of a trapezium ABCD with AB∣∣DC intersect each other at O . Prove that ar (AOD)= ar (BOC) .
View SolutionIn Figure, AC=AE, AB=AD and ∠BAD=∠EAC. Prove that BC=DE
View SolutionIn Figure, it is given that AB=BC and AD=EC . Prove that ABE≅CBD
View Solution