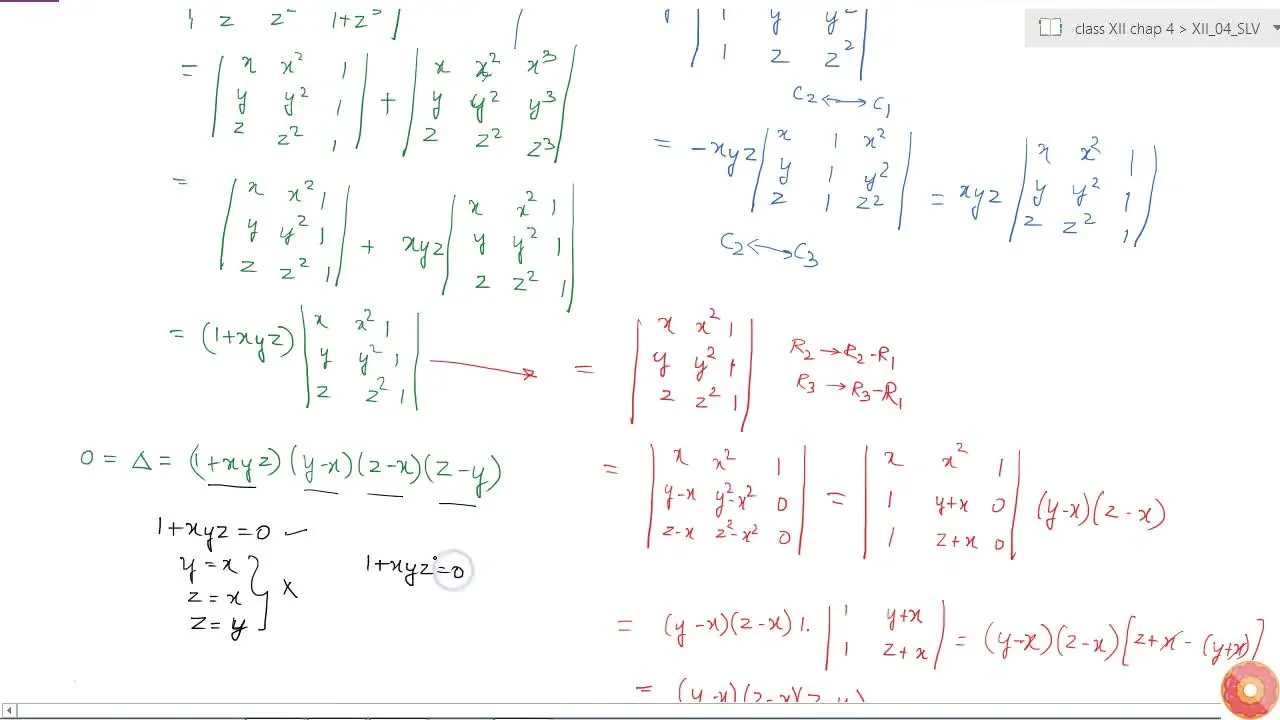
If x, y, z are different and Δ=∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0, then show that 1+xyz=0
Solving
Δ=∣∣
∣
∣∣xx21+x3yy21+y3zz21+z3∣∣
∣
∣∣
Here, expanding elements of C3 into two determinants
=∣∣
∣
∣∣xx21yy21zz21∣∣
∣
∣∣+∣∣
∣
∣∣xx2x3yy2y3zz2z3∣∣
∣
∣∣
Taking x, y, z common from R1,R2,R3 respectively from 2nd matrix.
=∣∣
∣
∣∣xx21yy21zz21∣∣
∣
∣∣+xyz∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣
In matrix 1st, Replacing C3↔C2
If any two columns of a determinant are interchanged, then sign of determinant changes.
=(−1)∣∣
∣
∣∣x1x2y1y2z1z2∣∣
∣
∣∣+xyz∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣
In matrix 1st, Replacing C1↔C2
=(−1)(−1)∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣+xyz∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣
=∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣+xyz∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣
=∣∣
∣
∣∣1xx21yy21zz2∣∣
∣
∣∣(1+xyz)
Using R2→R2−R1 and R3→R3−R1
=∣∣
∣
∣∣1xx21−1y−xy2−x21−1z−xz2−x2∣∣
∣
∣∣(1+xyz)
=∣∣
∣
∣∣1xx20(y−x)(y−x)(y+x)0(z−x)(z−x)(z+x)∣∣
∣
∣∣(1+xyz)
Taking common factor (y−x) from R2 & (z−x) from R3
=(1+xyz)(y−x)(z−x)∣∣
∣
∣∣1xx201(y+x)01(z+x)∣∣
∣
∣∣
Expanding determinant
=(1+xyz)(y−x)(z−x)(1∣∣∣1y+x1z+x∣∣∣−0∣∣∣xx21z+x∣∣∣+0∣∣∣xx21y+x∣∣∣)
=(1+xyz)(y−x)(z−x)[1(z+x)−1(y+x)−0+0]
=(1+xyz)(y−x)(z−x)(z+x−y−x)
=(1+xyz)(y−x)(z−x)(z−y)
∴Δ=(1+xyz)(y−x)(z−x)(z−y)
Since, Δ=0 given
(1+xyz)(y−x)(z−x)(z−y)=0
Since it is given that x,y,z all are different,
i.e. y−x≠0,z−x≠0,z−y≠0
So, only possibility is
(1+xyz)=0
Hence Proved
Related Playlists
- BOOK - NCERTCHAPTER - DETERMINANTSEXERCISE - MISCELLANEOUS EXERCISE19videos
DETERMINANTS
- BOOK - NCERTCHAPTER - DETERMINANTSEXERCISE - EXERCISE 4.45videos
DETERMINANTS
- BOOK - NCERTCHAPTER - DETERMINANTSEXERCISE - EXERCISE 4.615videos
DETERMINANTS
- BOOK - NCERTCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - QUESTION3videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - NCERTCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - EXERCISE 9.112videos
DIFFERENTIAL EQUATIONS
Similar Questions
If x, y, z are different and Δ=∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0 then show that 1+xyz=0
View SolutionIf x, y, z are different and Δ=∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0, then show that 1+xyz=0
View SolutionIf x,y,z are different and Δ=∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0, show that :
(i)1+xyz=0
(ii)xyz=−1View SolutionIf x,y,z are all distinct and
∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0
then the value of xyz isView SolutionIf x,y,z are different and Δ=∣∣ ∣ ∣∣xx2x3−1yy2y3−1zz2z3−1∣∣ ∣ ∣∣=0, then using properties of determinants, show that xyz=1
View SolutionIf x≠y≠zand∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0, then the value of xyz is a.1 b. 2 c. −1 d. 2
View SolutionIf ∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0 then relation of x,y and z is
View SolutionProve that : Det⎡⎢⎣xx2x3yy2y3zz2z3⎤⎥⎦=xyz(x−y)(y−z)(z−x)
View SolutionIf x,y,z are distinct and ∣∣ ∣ ∣∣xx(x2+1)x+1yy(y2+1)y+1zz(z2+1)z+1∣∣ ∣ ∣∣=0 then (A) xyz=0 (B) x+y+z=0 (C) xy+yz+zx=0 (D) x2+y2+z2=1
View SolutionIf x≠y≠z and ∣∣ ∣ ∣∣xx21+x3yy21+y3zz21+z3∣∣ ∣ ∣∣=0, then xyz =
View Solution