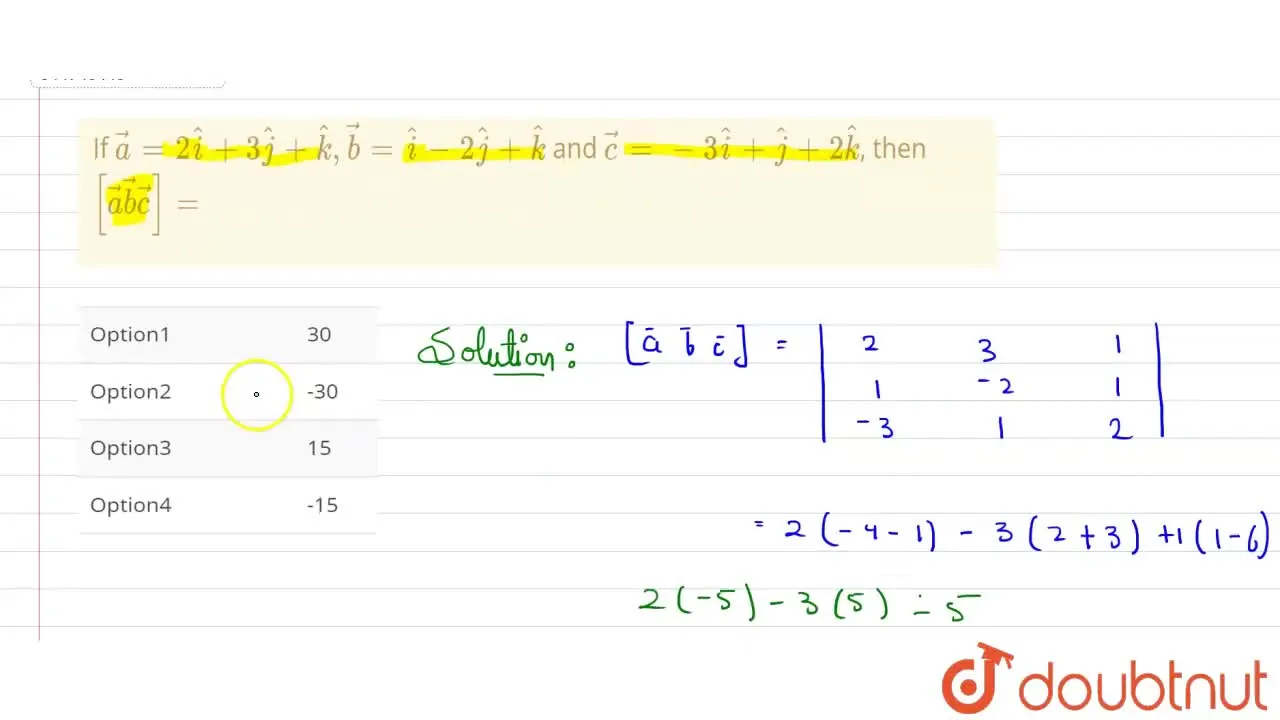
If →a=2ˆi+3ˆj+ˆk,→b=ˆi−2ˆj+ˆk and →c=−3ˆi+ˆj+2ˆk, then [→a→b→c]=
30
-30
15
-15
The correct Answer is:B
We have
[→a→b→c]=∣∣
∣∣2311−21−312∣∣
∣∣
⇒[→a→b→c]=2(−4−1)−3(2+3)+1(1−6)
⇒[→a→b→c]=−10−15−5=−30
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SCALAR AND VECTOR PRODUCTS OF THREE VECTORS EXERCISE - Section I - Solved Mcqs64videos
SCALAR AND VECTOR PRODUCTS OF THREE VECTORS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SCALAR AND VECTOR PRODUCTS OF THREE VECTORS EXERCISE - Section II - Assertion Reason Type12videos
SCALAR AND VECTOR PRODUCTS OF THREE VECTORS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - REAL FUNCTIONS EXERCISE - Chapter Test58videos
REAL FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SCALER AND VECTOR PRODUCTS OF TWO VECTORS EXERCISE - Section I - Solved Mcqs12videos
SCALER AND VECTOR PRODUCTS OF TWO VECTORS
Similar Questions
If →a=2ˆi−3ˆj−ˆk and →b=ˆi+4ˆj−2ˆk, then →a×→b is
View SolutionIf →aa=ˆi+2ˆj+3ˆk,→b=2ˆi−ˆj+ˆkand→c=ˆi+ˆj−2ˆk, verify that →a×(→b×→c)=(→a.→c)→b−(→a.→b)→c.
View SolutionIf →a=2ˆi+2ˆj−ˆk,→b=3ˆi−ˆj−ˆkand→c=ˆi+2ˆj−3ˆk then verify that →a×(→b×→c)=(→a.→c)→b−(→a.→b)→c.
View SolutionLet →a=ˆi−ˆj+ˆk,→b=2ˆi+ˆj+ˆkand→c=ˆi+ˆj−2ˆk, then the value of [→a→b→c] is equal to
View Solutionif →a=ˆi−ˆj−3ˆk,→b=4ˆi−3ˆj+ˆkand→c=2ˆi+ˆj+2ˆk, verify that →a×(→b+→c)=→a×→b+→a×→c
View SolutionLet →a=2ˆi+3ˆj+4ˆk,→b=ˆi−2ˆj+ˆk and →c=ˆi+ˆj−ˆk. If →r×→a=→b and →r.→c=3, then the value of |→r| is equal to
View SolutionIf →a=7ˆi+3ˆj−6ˆk, →b=2ˆi+5ˆj−ˆk and →c=−ˆi+2ˆj+4ˆk. Find (→a−→b)×(→c−→b).
View SolutionIf →a=ˆi+ˆj−ˆk,→b=−ˆi+2ˆj+2ˆkand→c=−ˆi+2ˆj−ˆk , then a unit vector normal to the vectors →a+→band→b−→c, is
View SolutionIf →a=2ˆi−3ˆj+5ˆk,→b=3ˆi−4ˆj+5ˆk and →c=5ˆi−3ˆj−2ˆk, then the volume of the parallelopiped with coterminous edges →a+→b,→b+→c,→c+→a is
View SolutionIf →a=7ˆi+3ˆj−5ˆk,→b=2ˆi+5ˆj−ˆkand→c−ˆi+2ˆj+4ˆk, then verify that →a×(b+c)=→a×→b+→a×→c
View Solution