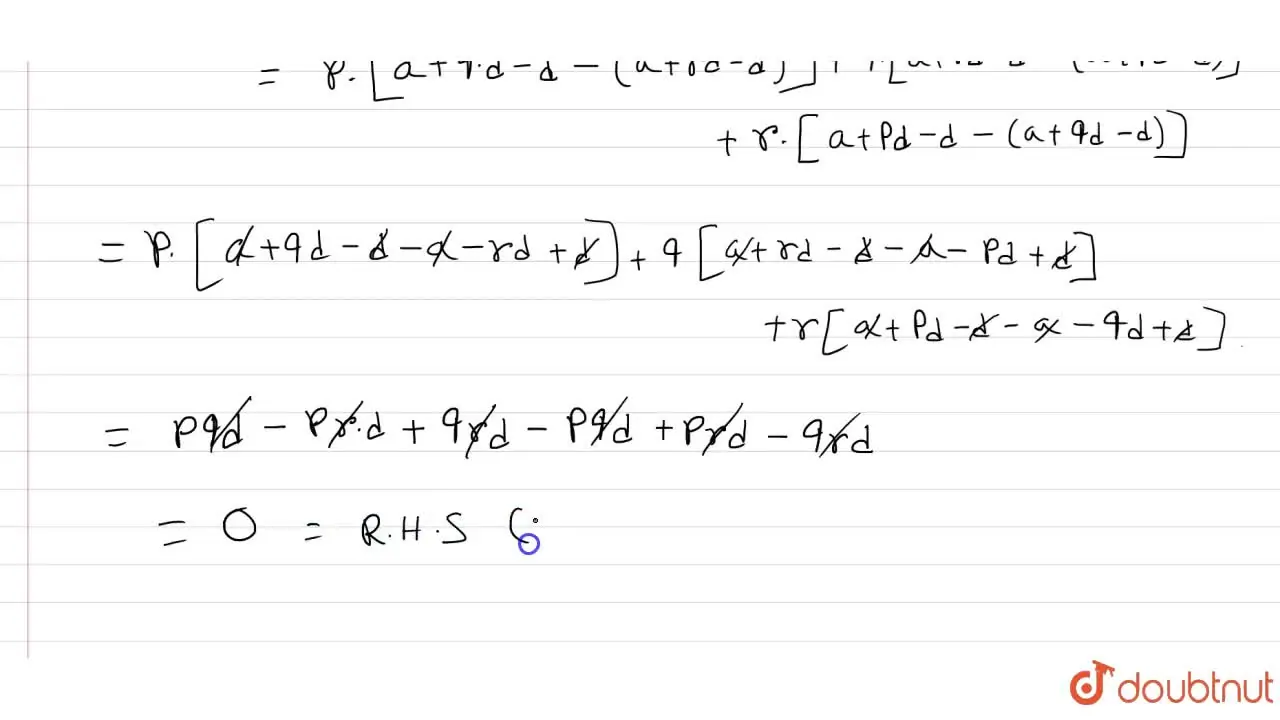If the pth , qth and rth terms of an A.P. be P, Q and R respectively then show that,
p(Q−R)+q(R−P)+r(P−Q)=0.Solution in Bengali
Step by step video & image solution for If the pth , qth and rth terms of an A.P. be P, Q and R respectively then show that, p(Q-R) + q(R-P) + r(P-Q) = 0. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - CHHAYA PUBLICATIONCHAPTER - SEQUENCE AND SERIESEXERCISE - Exercise 9 B (Long Answer Type Questions)52videos
SEQUENCE AND SERIES
- BOOK - CHHAYA PUBLICATIONCHAPTER - SEQUENCE AND SERIESEXERCISE - Exercise 9 C (Multiple Choice Type Questions)14videos
SEQUENCE AND SERIES
- BOOK - CHHAYA PUBLICATIONCHAPTER - SEQUENCE AND SERIESEXERCISE - Exercise 9 B (Very Short Answer Type Questions)18videos
SEQUENCE AND SERIES
- BOOK - CHHAYA PUBLICATIONCHAPTER - SECOND ORDER DERIVATIVEEXERCISE - Sample Question for Competitive Examination (Assertion-Reason type)2videos
SECOND ORDER DERIVATIVE
- BOOK - CHHAYA PUBLICATIONCHAPTER - SET THEORY EXERCISE - Assertion-Reason Type2videos
SET THEORY
Similar Questions
The sum of first 21 terms of an A.P. is 28 and the sum of the 1st 28 terms is 21. Show that one term of the progression is zero and find the sum of the preceding terms.
04:59View SolutionThe third term of a G.P. is b and its common ratio is r , then its first term =
03:31View SolutionThe 10th term of an A.P. is (-15) and the 31st term is (-57). Find the first term and common difference of the A.P.
06:17View SolutionGiven, x2+y2=1. Y' and y'' are first and second order derivative of y respectively.
Statement-I 1+yy''+(y')2=0
Statement- II xx'+2y=004:33View SolutionLet A={1,2,3}andB={3,8}
Statement-I : (A∪B)×(A∩B)={(1,3),(2,3),(3,3),(8,3)}
Statement-II : (A×B)∩(B×A)={(3,3)}08:38View SolutionIf the prth term of an A.P. is q and qth term is p, then rth term is (A) q−p+r (B) p−q+r (C) p+q+r (D) p+q−r
07:06View SolutionIf pth, qth, rth terms of an A.P. are in G.P. then common ratio of ths G.P. is (A) q−rp−q (B) q−sp−r (C) r−sq−r (D) qp
05:39View SolutionIf pth, qth and rth terms of an A.P. are a,b,c, respectively, then show that
(i) a(q-r)+b(r-p)+c(p-q)=0
(ii) (a-b)r+(b-c)p+(c-a)q=004:13View SolutionThe pth , qth and rth terms of an A.P. are in G.P. Prove that the common ratio of the G.P. is q−rp−q or p−qq−r
05:29View SolutionIf pth, qth, rth terms of an A.P are a,b,c then a(q - r ) + b(r - p ) + c(p - q) =
05:22View SolutionIf the pth ,qth and rth terms of a G.P are a,b and c respectively show that
(q−r)loga+(r−p)logb+(p−q)logc=005:39View SolutionThe pth, qth and rth terms of an A.P. are a, b and c respectively. Show that a(q – r) + b(r-p) + c(p – q) = 0
05:13View SolutionIf pth , qth, rth and sth terms of an A.P. and in G.P then p-q, q-r , r-s are in :
05:36View SolutionIf the pth, qth, and rth terms of an A.P. are in G.P., then the common ratio of the G.P. is a.prq2 b. rp c. q+rp+q d. q−rp−q
04:33View SolutionIf a,b,andc are respectively, the pth, qth , and rth terms of a G.P., show that (q−r)loga+(r−p)logb+(p−q)logc=0.
06:56View Solution